Brauner space

From Wikipedia, the free encyclopedia

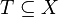In functional analysis and related areas of mathematics Brauner space is a complete compactly generated locally convex space $X$ having a sequence of compact sets $K_{n}$ such that every other compact set $T\subseteq X$ is contained in some $K_{n}$ .

Brauner spaces are named after Kalman Brauner,^[1] who first started to study them. All Brauner spaces are stereotype and are in the stereotype duality relations with Fréchet spaces:^[2]^[3]

for any Fréchet space $X$ its stereotype dual space^[4] $X^{\star }$ is a Brauner space,
and vice versa, for any Brauner space $X$ its stereotype dual space $X^{\star }$ is a Fréchet space.

Examples

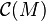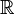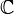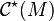Let $M$ be a $\sigma$ -compact locally compact topological space, and ${{\mathcal C}}(M)$ the space of all functions on $M$ (with values in ${{\mathbb R}}$ or ${{\mathbb C}}$ ), endowed with the usual topology of uniform convergence on compact sets in $M$ . 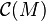The dual space ${{\mathcal C}}^{\star }(M)$ of measures with compact support in $M$ with the topology of uniform convergence on compact sets in ${{\mathcal C}}(M)$ is a Brauner space.

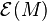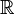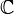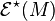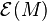Let $M$ be a smooth manifold, and ${{\mathcal E}}(M)$ the space of smooth functions on $M$ (with values in ${{\mathbb R}}$ or ${{\mathbb C}}$ ), endowed with the usual topology of uniform convergence with each derivative on compact sets in $M$ . The dual space ${{\mathcal E}}^{\star }(M)$ of distributions with compact support in $M$ with the topology of uniform convergence on bounded sets in ${{\mathcal E}}(M)$ is a Brauner space.

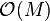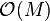Let $M$ be a Stein manifold and ${{\mathcal O}}(M)$ the space of holomorphic functions on $M$ with the usual topology of uniform convergence on compact sets in $M$ . The dual space ${{\mathcal O}}^{\star }(M)$ of analytic functionals on $M$ with the topology of uniform convergence on biunded sets in ${{\mathcal O}}(M)$ is a Brauner space.

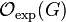Let $G$ be a compactly generated Stein group. The space ${{\mathcal O}}_{{\exp }}(G)$ of holomorphic functions of exponential type on $G$ is a Brauner space with respect to a natural topology.^[3]

Notes

↑ K.Brauner (1973).
↑ S.S.Akbarov (2003).
↑ 3.0 3.1 S.S.Akbarov (2009).
↑ The stereotype dual space to a locally convex space $X$ is the space $X^{\star }$ of all linear continuous functionals $f:X\to {\mathbb {C}}$ endowed with the topology of uniform convergence on totally bounded sets in $X$ .

References

Schaefer, Helmuth H. (1966). Topological vector spaces. New York: The MacMillan Company. ISBN 0-387-98726-6.

Robertson, A.P.; Robertson, W.J. (1964). Topological vector spaces. Cambridge Tracts in Mathematics 53. Cambridge University Press. Cite uses deprecated parameters (help)

Brauner, K. (1973). "Duals of Frechet spaces and a generalization of the Banach-Dieudonne theorem". Duke Math. Jour. 40 (4): 845–855. doi:10.1215/S0012-7094-73-04078-7.

Akbarov, S.S. (2003). "Pontryagin duality in the theory of topological vector spaces and in topological algebra". Journal of Mathematical Sciences 113 (2): 179–349. doi:10.1023/A:1020929201133.

Akbarov, S.S. (2009). "Holomorphic functions of exponential type and duality for Stein groups with algebraic connected component of identity(subscription required)". Journal of Mathematical Sciences 162 (4): 459–586. doi:10.1007/s10958-009-9646-1.

v t e Functional analysis

Local Notions on Sets	Absolutely convex Absorbing Balanced Bounded Cones (Linear, Convex cone) Convex Radial Star-shaped Symmetric

Types of TVSs	Banach Barrelled Bornological Brauner F-space Finite-dimensional Fréchet (Tame) Hilbert LF Locally convex Mackey Montel Nuclear Normed (Norm) Reflexive Riesz Smith Stereotype Topological tensor product (of Hilbert spaces) Webbed

Spaces of Maps	Dual space Dual topology Operator topologies Strong Topology (Polar, Operator) Topology of uniform convergence Weak topology (operator) Ultrastrong topology Ultraweak topology

Types of Linear operators	Adjoint Bilinear (form) Bounded (Unbounded) Closed Continuous (Discontinuous) Compact Fredholm Hilbert–Schmidt Functionals (Positive) Normal Nuclear Self-adjoint Strictly singular Trace class Transpose Unitary

Operations on Sets	Algebraic interior (core) Interior Minkowski addition Polar

Banach Algebras	C-algebras Spectrum (of a C-algebra, Spectral radius) Spectral theory

Important Theorems	Banach–Alaoglu Banach–Saks Banach–Mazur Bessel's inequality Cauchy–Schwarz inequality Closed range Closed graph Eberlein–Šmulian Freudenthal spectral Gelfand–Mazur Goldstine Hahn–Banach (Hyperplane separation) Kakutani fixed-point Lomonosov's Invariant Subspace Mackey–Arens Mazur's lemma M. Riesz extension Open mapping Parseval's identity Schauder fixed-point

Analysis	Bochner space Derivatives (Fréchet derivative, Gâteaux derivative, Functional, Holomorphy) Differentiation in Fréchet spaces Integrals (Bochner, Dunford, Gelfand–Pettis, Regulated, Weak) Functional calculus Inverse function theorem (Nash–Moser theorem) Vector measure

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.