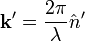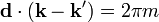Bragg plane

In physics, a Bragg plane is a plane in reciprocal space which bisects one reciprocal lattice vector  .[1] It is relevant to define this plane as part of the definition of the Von Laue condition for diffraction peaks in x-ray diffraction crystallography.
.[1] It is relevant to define this plane as part of the definition of the Von Laue condition for diffraction peaks in x-ray diffraction crystallography.
Considering the diagram at right, the arriving x-ray plane wave is defined by:
Where  is the incident wave vector given by:
is the incident wave vector given by:
where  is the wavelength of the incident photon. While the Bragg formulation assumes a unique choice of direct lattice planes and specular reflection of the incident X-rays, the Von Laue formula only assumes monochromatic light and that each scattering center acts as a source of secondary wavelets as described by the Huygens principle. Each scattered wave contributes to a new plane wave given by:
is the wavelength of the incident photon. While the Bragg formulation assumes a unique choice of direct lattice planes and specular reflection of the incident X-rays, the Von Laue formula only assumes monochromatic light and that each scattering center acts as a source of secondary wavelets as described by the Huygens principle. Each scattered wave contributes to a new plane wave given by:
The condition for constructive interference in the 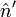 direction is that the path difference between the photons is an integer multiple (m) of their wavelength. We know then that for constructive interference we have:
direction is that the path difference between the photons is an integer multiple (m) of their wavelength. We know then that for constructive interference we have:
where  . Multiplying the above by
. Multiplying the above by  we formulate the condition in terms of the wave vectors
we formulate the condition in terms of the wave vectors  and
and  :
:

Now consider that a crystal is an array of scattering centres, each at a point in the Bravais lattice. We can set one of the scattering centres as the origin of an array. Since the lattice points are displaced by the Bravais lattice vectors  , scattered waves interfere constructively when the above condition holds simultaneously for all values of
, scattered waves interfere constructively when the above condition holds simultaneously for all values of  which are Bravais lattice vectors, the condition then becomes:
which are Bravais lattice vectors, the condition then becomes:
An equivalent statement (see mathematical description of the reciprocal lattice) is to say that:
By comparing this equation with the definition of a reciprocal lattice vector, we see that constructive interference occurs if  is a vector of the reciprocal lattice. We notice that
is a vector of the reciprocal lattice. We notice that  and
and  have the same magnitude, we can restate the Von Laue formulation as requiring that the tip of incident wave vector
have the same magnitude, we can restate the Von Laue formulation as requiring that the tip of incident wave vector  must lie in the plane that is a perpendicular bisector of the reciprocal lattice vector
must lie in the plane that is a perpendicular bisector of the reciprocal lattice vector 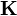 . This reciprocal space plane is the Bragg plane.
. This reciprocal space plane is the Bragg plane.
References
See also
- X-ray crystallography
- Reciprocal lattice
- Bravais lattice
- Powder diffraction
- Kikuchi line
- Brillouin zone