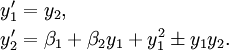Bogdanov–Takens bifurcation

From Wikipedia, the free encyclopedia
Bifurcation diagrams with parameters β1, β2 = (from top-left to bottom-right): (−1,1), (1/4,−1), (1,0), (0,0), (−6/25,−1), (0,1).

In bifurcation theory, a field within mathematics, a Bogdanov–Takens bifurcation is a well-studied example of a bifurcation with co-dimension two, meaning that two parameters must be varied for the bifurcation to occur. It is named after Rifkat Bogdanov and Floris Takens, who independently and simultaneously described this bifurcation.

A system y' = f(y) undergoes a Bogdanov–Takens bifurcation if it has a fixed point and the linearization of f around that point has a double eigenvalue at zero (assuming that some technical nondegeneracy conditions are satisfied).

Three codimension-one bifurcations occur nearby: a saddle-node bifurcation, an Andronov–Hopf bifurcation and a homoclinic bifurcation. All associated bifurcation curves meet at the Bogdanov–Takens bifurcation.

The normal form of the Bogdanov–Takens bifurcation is

{\begin{aligned}y_{1}'&=y_{2},\\y_{2}'&=\beta _{1}+\beta _{2}y_{1}+y_{1}^{2}\pm y_{1}y_{2}.\end{aligned}}

It has also been found the existence of a codimension-three degenerate Takens–Bogdanov bifurcation, also known as Dumortier–Roussarie–Sotomayor bifurcation.

References

  • Bogdanov, R. "Bifurcations of a Limit Cycle for a Family of Vector Fields on the Plane." Selecta Math. Soviet 1, 373–388, 1981.
  • Kuznetsov, Y. A. Elements of Applied Bifurcation Theory. New York: Springer-Verlag, 1995.
  • Takens, F. "Forced Oscillations and Bifurcations." Comm. Math. Inst. Rijksuniv. Utrecht 2, 1–111, 1974.
  • Dumortier F., Roussarie R., Sotomayor J. and Zoladek H., Bifurcations of Planar Vector Fields, Lecture Notes in Math. vol. 1480, 1–164, Springer-Verlag (1991).

External links

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.