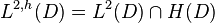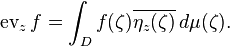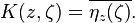Bergman kernel

From Wikipedia, the free encyclopedia

In the mathematical study of several complex variables, the Bergman kernel, named after Stefan Bergman, is a reproducing kernel for the Hilbert space of all square integrable holomorphic functions on a domain D in Cn.

In detail, let L2(D) be the Hilbert space of square integrable functions on D, and let L2,h(D) denote the subspace consisting of holomorphic functions in D: that is,

L^{{2,h}}(D)=L^{2}(D)\cap H(D)

where H(D) is the space of holomorphic functions in D. Then L2,h(D) is a Hilbert space: it is a closed linear subspace of L2(D), and therefore complete in its own right. This follows from the fundamental estimate, that for a holomorphic square-integrable function ƒ in D

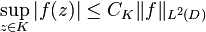
\sup _{{z\in K}}|f(z)|\leq C_{K}\|f\|_{{L^{2}(D)}}

 

 

 

 

(1)

for every compact subset K of D. Thus convergence of a sequence of holomorphic functions in L2(D) implies also compact convergence, and so the limit function is also holomorphic.

Another consequence of (1) is that, for each z ∈ D, the evaluation

\operatorname {ev}_{z}:f\mapsto f(z)

is a continuous linear functional on L2,h(D). By the Riesz representation theorem, this functional can be represented as the inner product with an element of L2,h(D), which is to say that

\operatorname {ev}_{z}f=\int _{D}f(\zeta )\overline {\eta _{z}(\zeta )}\,d\mu (\zeta ).

The Bergman kernel K is defined by

K(z,\zeta )=\overline {\eta _{z}(\zeta )}.

The kernel K(z,ζ) holomorphic in z and antiholomorphic in ζ, and satisfies

f(z)=\int _{D}K(z,\zeta )f(\zeta )\,d\mu (\zeta ).

See also

References

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.