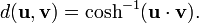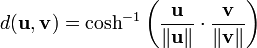Beltrami–Klein model

From Wikipedia, the free encyclopedia

Lines in the projective model of the hyperbolic plane.

A hyperbolic triheptagonal tiling in a Beltrami–Klein model projection

The regular hyperbolic dodecahedral honeycomb, {5,3,4}

In geometry, the Beltrami–Klein model, also called the projective model, Klein disk model, and the Cayley–Klein model, is a model of n-dimensional hyperbolic geometry in which points are represented by the points in the interior of the n-dimensional unit ball (or unit disk, in two dimensions) and lines are represented by the chords, straight line segments with endpoints on the boundary sphere. It made its first appearance in two memoirs of Eugenio Beltrami published in 1868, first for n = 2 and then for general n, devoted to showing equiconsistency of hyperbolic geometry with ordinary Euclidean geometry.^[1]^[2]

The Beltrami–Klein model is strongly analogous to the gnomonic projection of spherical geometry, which maps great circles to straight lines; the formulae relating these two to the hyperboloid model and the sphere, respectively, are very similar.

The distance is given by the Cayley–Klein metric and was first written down by Arthur Cayley in the context of projective and spherical geometry. Felix Klein recognized its importance for non-Euclidean geometry and popularized the subject.

Distance formula

Arthur Cayley applied the cross-ratio from projective geometry to measurement of distances and angles in spherical geometry.^[3] Later, Felix Klein realized that Cayley's ideas give rise to a projective model of the non-Euclidean plane.^[4] Given two distinct points p and q in the open unit ball, the unique straight line connecting them intersects the unit sphere in two points, a and b, labeled so that the points are, in order, a, p, q, b. Then the hyperbolic distance between p and q is expressed as

$d(p,q)={\frac {1}{2}}\log {\frac {|qa||bp|}{|pa||bq|}}$

where the vertical bars indicate Euclidean distances. The factor of one half is needed to make the curvature −1.

Relation to the hyperboloid model

The hyperboloid model is a model of hyperbolic geometry within (n + 1)-dimensional Minkowski space. The Minkowski inner product is given by

${\mathbf {x}}\cdot {\mathbf {y}}=x_{0}y_{0}-x_{1}y_{1}-\cdots -x_{n}y_{n}\,$

and the norm by $\|{\mathbf {x}}\|={\sqrt {{\mathbf {x}}\cdot {\mathbf {x}}}}$ . The hyperbolic plane is embedded in this space as the vectors x with ||x|| = 1 and x₀ (the "timelike component") positive. The intrinsic distance (in the embedding) between points u and v is then given by

$d({\mathbf {u}},{\mathbf {v}})=\cosh ^{{-1}}({\mathbf {u}}\cdot {\mathbf {v}}).\,$

This may also be written in the homogeneous form

$d({\mathbf {u}},{\mathbf {v}})=\cosh ^{{-1}}\left({\frac {{\mathbf {u}}}{\|{\mathbf {u}}\|}}\cdot {\frac {{\mathbf {v}}}{\|{\mathbf {v}}\|}}\right)$

which allows the vectors to be rescaled for convenience.

The Beltrami–Klein model is obtained from the hyperboloid model by rescaling all vectors so that the timelike component is 1, that is, by projecting the hyperboloid embedding through the origin onto the plane x₀ = 1. The distance function, in its homogeneous form, is unchanged. Since the intrinsic lines (geodesics) of the hyperboloid model are the intersection of the embedding with planes through the Minkowski origin, the intrinsic lines of the Beltrami–Klein model are the chords of the sphere.

In the gyrovector space approach to hyperbolic geometry, vector algebra in the Beltrami–Klein model can be developed using relativistic 3-velocities as the vectors, analogously to the use of ordinary vectors in Euclidean geometry.

Relation to the Poincaré disk model

Both the Poincaré disk model and the Beltrami–Klein model are models of the n-dimensional hyperbolic space in the n-dimensional unit ball in Rⁿ. If $u$ is a vector of norm less than one representing a point of the Poincaré disk model, then the corresponding point of the Beltrami–Klein model is given by

$s={\frac {2u}{1+u\cdot u}}.$

Conversely, from a vector $s$ of norm less than one representing a point of the Beltrami–Klein model, the corresponding point of the Poincaré disk model is given by

$u={\frac {s}{1+{\sqrt {1-s\cdot s}}}}={\frac {\left(1-{\sqrt {1-s\cdot s}}\right)s}{s\cdot s}}.$

Given two points on the boundary of the unit disk, which are traditionally called ideal points, the straight line connecting them in the Beltrami–Klein model is the chord between them, while in the corresponding Poincaré model the line is a circular arc on the two-dimensional subspace generated by the two boundary point vectors, orthogonal to the boundary of the disk. The two models are related through a projection from the center of the disk; a ray from the center passing through a point of one model line passes through the corresponding point of the line in the other model.

Notes

↑ Beltrami, Eugenio (1868). "Saggio di interpretazione della geometria non-euclidea". Giornale di Mathematiche VI: 285–315.
↑ Beltrami, Eugenio (1868). "Teoria fondamentale degli spazii di curvatura costante". Annali. di Mat., ser II 2: 232–255. doi:10.1007/BF02419615.
↑ Cayley, Arthur (1859). "A Sixth Memoire upon Quantics". Philosophical Transactions of the Royal Society 159: 61–91. doi:10.1098/rstl.1859.0004.
↑ Klein, Felix (1871). "Ueber die sogenannte Nicht-Euklidische Geometrie". Mathematische Annalen 4: 573–625. doi:10.1007/BF02100583.

References

Luis Santaló (1961), Geometrias no Euclidianas, EUDEBA.
Stahl, Saul (1993). The Poincaré Half-Plane. Jones and Bartlett.
Nielsen, Frank; Nock, Richard (2009). "Hyperbolic Voronoi diagrams made easy". arXiv:0903.3287.

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.