Wilks' lambda distribution

In statistics, Wilks' lambda distribution (named for Samuel S. Wilks), is a probability distribution used in multivariate hypothesis testing, especially with regard to the likelihood-ratio test and Multivariate analysis of variance. It is a multivariate generalization of the univariate F-distribution, and generalizes Hotelling's T-squared distribution in the same way that the F-distribution generalizes Student's t-distribution.

Wilks' lambda distribution is related to two independent Wishart distributed variables, and is defined as follows,[1]

given

A \sim W_p(I, m) \qquad B \sim W_p(I, n)

independent and with m \ge p

\lambda = \frac{\det(A)}{\det(A%2BB)} = \frac{1}{\det(I%2BA^{-1}B)} \sim \Lambda(p,m,n)

where p is the number of dimensions. In the context of likelihood-ratio tests m is typically the error degrees of freedom, and n is the hypothesis degrees of freedom, so that 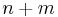n%2Bm is the total degrees of freedom.[1]

The distribution can be related to a product of independent Beta distributed random variables

u_i \sim B\left(\frac{m%2Bi-p}{2},\frac{p}{2}\right)
\prod_{i=1}^n u_i \sim \Lambda(p,m,n).

For large m Bartlett's approximation [2] allows Wilks' lambda to be approximated with a Chi-squared distribution

\left(\frac{p-n%2B1}{2}-m\right)\log \Lambda(p,m,n) \sim \chi^2_{np}.[1]

References

  1. ^ a b c Mardia, K.V.; J.T. Kent, J.M. Bibby (1979). Multivariate Analysis. Academic Press. 
  2. ^ Bartlett, M.S. (1954). "A note on multiplying factors for various 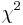\chi^2 approximations". J. Royal Statist. Soc. Series B 16: 296–298. 
 
Benini · Benktander 1st kind · Benktander 2nd kind · Beta prime · Bose–Einstein · Burr · chi-squared · chi · Coxian · Dagum · Davis · Erlang · exponential · F · Fermi–Dirac · folded normal · Fréchet · Gamma · generalized inverse Gaussian · half-logistic · half-normal · Hotelling's T-squared · hyper-exponential · hypoexponential · inverse chi-squared (scaled-inverse-chi-squared) · inverse Gaussian · inverse gamma · Kolmogorov · Lévy · log-Cauchy · log-Laplace · log-logistic · log-normal · Maxwell–Boltzmann · Maxwell speed · Mittag–Leffler · Nakagami · noncentral chi-squared · Pareto · phase-type · Rayleigh · relativistic Breit–Wigner · Rice · Rosin–Rammler · shifted Gompertz · truncated normal · type-2 Gumbel · Weibull · Wilks' lambda