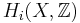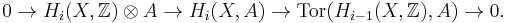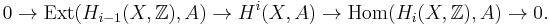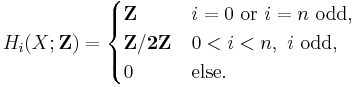Universal coefficient theorem
In mathematics, the universal coefficient theorem in algebraic topology establishes the relationship in homology theory between the integral homology of a topological space X, and its homology with coefficients in any abelian group A. It states that the integral homology groups
completely determine the groups
Here 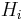 might be the simplicial homology or more general singular homology theory: the result itself is a pure piece of homological algebra about chain complexes of free abelian groups. The form of the result is that other coefficients A may be used, at the cost of using a Tor functor.
might be the simplicial homology or more general singular homology theory: the result itself is a pure piece of homological algebra about chain complexes of free abelian groups. The form of the result is that other coefficients A may be used, at the cost of using a Tor functor.
For example it is common to take A to be 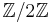 , so that coefficients are modulo 2. This becomes straightforward in the absence of 2-torsion in the homology. Quite generally, the result indicates the relationship that holds between the Betti numbers bi of X and the Betti numbers bi,F with coefficients in a field F. These can differ, but only when the characteristic of F is a prime number p for which there is some p-torsion in the homology.
, so that coefficients are modulo 2. This becomes straightforward in the absence of 2-torsion in the homology. Quite generally, the result indicates the relationship that holds between the Betti numbers bi of X and the Betti numbers bi,F with coefficients in a field F. These can differ, but only when the characteristic of F is a prime number p for which there is some p-torsion in the homology.
Contents |
Statement
Consider the tensor product 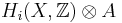 . The theorem states that there is an injective group homomorphism ι from this group to
. The theorem states that there is an injective group homomorphism ι from this group to 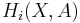 , which has cokernel
, which has cokernel  .
.
In other words, there is a natural short exact sequence
Furthermore, this is a split sequence (but the splitting is not natural).
The Tor group on the right can be thought of as the obstruction to ι being an isomorphism.
Universal coefficient theorem for cohomology
There is also a universal coefficient theorem for cohomology involving the Ext functor, stating that there is a natural short exact sequence
As in the homological case, the sequence splits, though not naturally.
Example: mod 2 cohomology of the real projective space
Let 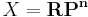 , the real projective space. We compute the singular cohomology of X with coefficients in
, the real projective space. We compute the singular cohomology of X with coefficients in
 .
.
knowing that the integer homology is given by:
We have 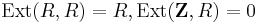 , so that the above exact sequences yield
, so that the above exact sequences yield
 .
.
In fact the total cohomology ring structure is
![H^*(X; R) = R [w]/<w^{n%2B1}>](/2012-wikipedia_en_all_nopic_01_2012/I/e7beafee2eb32dfe737c2db86e7f8b8e.png) .
.
References
- Allen Hatcher, Algebraic Topology , Cambridge University Press, Cambridge, 2002. ISBN 0-521-79540-0. A modern, geometrically flavored introduction to algebraic topology. The book is available free in PDF and PostScript formats on the author's homepage.