Space diagonal
In a rectangular box or a magic cube, the four space diagonals are the lines that go from a corner of the box or cube, through the center of the box or cube, to the opposite corner. These lines are also called triagonals or volume diagonals.
For the cube to be considered magic, these four lines must sum correctly.
The word triagonal is derived from the fact that as you travel down the line, three coordinates change. The equivalent in a square is diagonal, because two coordinates change. In a tesseract it is quadragonal because 4 coordinates change, etc.
The space diagonal of a cube with side length a is 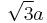 .
.
Contents |
r-agonals
This section applies particularly to Magic hypercubes.
The magic hypercube community has started to recognize an abbreviated expression for these space diagonals. By using r as a variable to describe the various agonals, a concise notation is possible.
If r =
- 2 then we have a diagonal. 2 coordinates change.
- 3 = a triagonal. 3 coordinates change
- 4 = a quadragonal. 4 coordinates change
- n = the dimension of the hypercube. These 2n-1 agonals are required to sum correctly for the hypercube to be considered magic.
... By extension,
- 1 = the lines parallel to the faces. Only 1 coordinate changes. A 1-agonal may be called a monagonal, in keeping with a diagonal, a triagonal, etc. Lines parallel to the faces of the hypercube have, in the past, also been referred to as i-rows.
Because the prefix pan indicates all, we can concisely state the characteristics or a magic hypercube.
Post the formula for the number of space diagonals, as it is a necessary formula.
For example;
- If pan-r-agonals sum correctly for r = 1 and 2, we know our square is pandiagonal magic.
- If pan-r-agonals sum correctly for r = 1 and 3, we have a pantriagonal magic cube (the equivalent of a pandiagonal magic square).
- If the r-agonals sum correctly for r = 1 and n, then we know that the magic hypercube is simple magic regardless of what dimension it is!!
The length of an r-agonal of a hypercube with side length a is 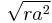 .
.
See also
References
- John R. Hendricks, The Pan-3-Agonal Magic Cube, Journal of Recreational Mathematics 5:1:1972, pp 51-54. First published mention of pan-3-agonals
- Hendricks, J. R., Magic Squares to Tesseracts by Computer, 1998, 0-9684700-0-9, page 49
- Heinz & Hendricks, Magic Square Lexicon: Illustrated, 2000, 0-9687985-0-0, pages 99,165