Shelling (topology)
In mathematics, a shelling of a simplicial complex is a way of gluing it together from its maximal simplices in a well-behaved way. A complex admitting a shelling is called shellable.
Contents |
Definition
A d-dimensional simplicial complex is called pure if its maximal simplices all have dimension d. Let  be a finite or countably infinite simplicial complex. An ordering
be a finite or countably infinite simplicial complex. An ordering 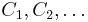 of the maximal simplices of
of the maximal simplices of  is a shelling if the complex
is a shelling if the complex 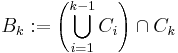 is pure and
is pure and 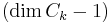 -dimensional for all
-dimensional for all 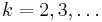 . If
. If  is the entire boundary of
is the entire boundary of 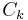 then
then 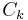 is called spanning.
is called spanning.
For  not necessarily countable, one can define a shelling as a well-ordering of the maximal simplices of
not necessarily countable, one can define a shelling as a well-ordering of the maximal simplices of  having analogous properties.
having analogous properties.
Properties
- A shellable complex is homotopy equivalent to a wedge sum of spheres, one for each spanning simplex and of corresponding dimension.
- A shellable complex may admit many different shellings, but the number of spanning simplices, and their dimensions, do not depend on the choice of shelling. This follows from the previous property.
Examples
References
- Dmitry Kozlov (2008). Combinatorial Algebraic Topology. Berlin: Springer. ISBN 978-3-540-71961-8.
- ^ Björner, Anders (1984-06). "Some combinatorial and algebraic properties of Coxeter complexes and Tits buildings". Advances in Mathematics 52 (3): 173–212. doi:10.1016/0001-8708(84)90021-5. ISSN 0001-8708.