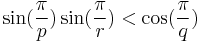Schläfli–Hess polychoron
In four-dimensional geometry, Schläfli–Hess polychora are the complete set of 10 regular self-intersecting star polychora (four-dimensional polytopes). They are named in honor of their discoverers: Ludwig Schläfli and Edmund Hess. Each is represented by a Schläfli symbol {p,q,r} in which one of the numbers is 5/2. They are thus analogous to the regular nonconvex Kepler–Poinsot polyhedra.
Allowing for regular star polygons as cells and vertex figures, these 10 polychora add to the set of six regular convex 4-polytopes. All may be derived as stellations of the 120-cell {5,3,3} or the 600-cell {3,3,5}.
Contents |
History
Four of them were found by Ludwig Schläfli while the other six were skipped because he would not allow forms that failed the Euler characteristic on cells or vertex figures (for zero-hole tori: F − E + V = 2). That excludes cells and vertex figures as {5,5/2}, and {5/2,5}.
Edmund Hess (1843–1903) published the complete list in his 1883 German book Einleitung in die Lehre von der Kugelteilung mit besonderer Berücksichtigung ihrer Anwendung auf die Theorie der Gleichflächigen und der gleicheckigen Polyeder.
Names
Their names given here were given by John Conway, extending Cayley's names for the Kepler–Poinsot polyhedra: along with stellated and great, he adds a grand modifier. Conway offered these operational definitions:
- stellation – replaces edges by longer edges in same lines. (Example: a pentagon stellates into a pentagram)
- greatening – replaces the faces by large ones in same planes. (Example: an icosahedron greatens into a great icosahedron)
- aggrandizement – replaces the cells by large ones in same 3-spaces.
Symmetry
All ten polychora have [3,3,5] (H4) hexacosichoric symmetry. They are generated from 6 related rational-order symmetry groups: [3,5,5/2], [5,5/2,5], [5,3,5/2], [5/2,5,5/2], [5,5/2,3], [3,3,5/2].
Each group has 2 regular star-polychora, except for two groups which are self-dual, having only one. So there are 4 dual-pairs and 2 self-dual forms among the ten regular star polychora.
Table of elements
Note:
- There are 2 unique vertex arrangements, matching those of the 120-cell and 600-cell.
- There are 4 unique edge arrangements, which are shown as wireframes orthographic projections.
- There are 7 unique face arrangements, shown as solids (face-colored) orthographic projections.
The cells (polyhedra), their faces (polygons), the polygonal edge figures and polyhedral vertex figures are identified by their Schläfli symbols.
| Name |
Wireframe | Solid | Schläfli {p, q,r} Coxeter–Dynkin |
Cells {p, q} |
Faces {p} |
Edges {r} |
Vertices {q, r} |
Density | χ | Dual {r, q,p} |
|---|---|---|---|---|---|---|---|---|---|---|
| Icosahedral 120-cell | {3,5,5/2} |
120 {3,5} |
1200 {3} |
720 {5/2} |
120 {5,5/2} |
4 | 480 | Small stellated 120-cell | ||
| Small stellated 120-cell | {5/2,5,3} |
120 {5/2,5} |
720 {5/2} |
1200 {3} |
120 {5,3} |
4 | −480 | Icosahedral 120-cell | ||
| Great 120-cell | {5,5/2,5} |
120 {5,5/2} |
720 {5} |
720 {5} |
120 {5/2,5} |
6 | 0 | Self-dual | ||
| Grand 120-cell | {5,3,5/2} |
120 {5,3} |
720 {5} |
720 {5/2} |
120 {3,5/2} |
20 | 0 | Great stellated 120-cell | ||
| Great stellated 120-cell | {5/2,3,5} |
120 {5/2,3} |
720 {5/2} |
720 {5} |
120 {3,5} |
20 | 0 | Grand 120-cell | ||
| Grand stellated 120-cell | {5/2,5,5/2} |
120 {5/2,5} |
720 {5/2} |
720 {5/2} |
120 {5,5/2} |
66 | 0 | Self-dual | ||
| Great grand 120-cell | {5,5/2,3} |
120 {5,5/2} |
720 {5} |
1200 {3} |
120 {5/2,3} |
76 | −480 | Great icosahedral 120-cell | ||
| Great icosahedral 120-cell | {3,5/2,5} |
120 {3,5/2} |
1200 {3} |
720 {5} |
120 {5/2,5} |
76 | 480 | Great grand 120-cell | ||
| Grand 600-cell | {3,3,5/2} |
600 {3,3} |
1200 {3} |
720 {5/2} |
120 {3,5/2} |
191 | 0 | Great grand stellated 120-cell | ||
| Great grand stellated 120-cell | {5/2,3,3} |
120 {5/2,3} |
720 {5/2} |
1200 {3} |
600 {3,3} |
191 | 0 | Grand 600-cell |
Existence
The existence of a regular polychoron 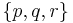 is constrained by the existence of the regular polyhedra
is constrained by the existence of the regular polyhedra 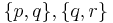 and a dihedral angle constraint:
and a dihedral angle constraint:
The six regular convex polytopes and 10 star polytopes above are the only solutions to these constraints.
There are four nonconvex Schläfli symbols {p,q,r} that have valid cells {p,q} and vertex figures {q,r}, and pass the dihedral test, but fail to produce finite figures: {3,5/2,3}, {4,3,5/2}, {5/2,3,4}, {5/2,3,5/2}.
See also
- List of regular polytopes
- Convex regular 4-polytope – Set of convex regular polychoron
- Kepler-Poinsot polyhedra – regular star polyhedron
- Star polygon – regular star polygons
References
- Edmund Hess, (1883) Einleitung in die Lehre von der Kugelteilung mit besonderer Berücksichtigung ihrer Anwendung auf die Theorie der Gleichflächigen und der gleicheckigen Polyeder [1].
- Edmund Hess Uber die regulären Polytope höherer Art, Sitzungsber Gesells Beförderung gesammten Naturwiss Marburg, 1885, 31-57
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [2]
- (Paper 10) H.S.M. Coxeter, Star Polytopes and the Schlafli Function f(α,β,γ) [Elemente der Mathematik 44 (2) (1989) 25–36]
- Coxeter, Regular Polytopes, 3rd. ed., Dover Publications, 1973. ISBN 0-486-61480-8. (Table I(ii): 16 regular polytopes {p, q,r} in four dimensions, pp. 292–293)
- H. S. M. Coxeter, Regular Complex Polytopes, 2nd. ed., Cambridge University Press 1991. ISBN 978-0521394901. [3]
- Peter McMullen and Egon Schulte, Abstract Regular Polytopes, 2002, PDF
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 26, Regular Star-polytopes, pp. 404–408)
External links
- Weisstein, Eric W., "Regular polychoron" from MathWorld.
- Olshevsky, George, Hecatonicosachoron at Glossary for Hyperspace.
- Olshevsky, George, Hexacosichoron at Glossary for Hyperspace.
- Olshevsky, George, Stellation at Glossary for Hyperspace.
- Olshevsky, George, Greatening at Glossary for Hyperspace.
- Olshevsky, George, Aggrandizement at Glossary for Hyperspace.
- Jonathan Bowers, 16 regular polychora
- Discussion on names
- Reguläre Polytope
- The Regular Star Polychora
- Stella4D Stella (software) produces interactive views of all 1849 known uniform polychora including the 64 convex forms and the infinite prismatic families. Was used to create images for this page.