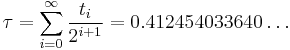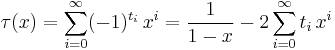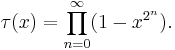Prouhet–Thue–Morse constant

In mathematics, the Prouhet–Thue–Morse constant, named for Eugène Prouhet, Axel Thue, and Marston Morse, is the number—denoted by 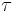$\tau$ —whose binary expansion .01101001100101101001011001101001... is given by the Thue–Morse sequence. That is,

$\tau = \sum_{i=0}^{\infty} \frac{t_i}{2^{i%2B1}} = 0.412454033640 \ldots$

where $t_i$ is the i^th element of the Prouhet–Thue–Morse sequence.

The generating series for the $t_i$ is given by

$\tau(x) = \sum_{i=0}^{\infty} (-1)^{t_i} \, x^i = \frac{1}{1-x} - 2 \sum_{i=0}^{\infty} t_i \, x^i$

and can be expressed as

$\tau(x) = \prod_{n=0}^{\infty} ( 1 - x^{2^n} ).$

This is the product of Frobenius polynomials, and thus generalizes to arbitrary fields.

The Prouhet–Thue–Morse constant was shown to be transcendental by Kurt Mahler in 1929.^[1]

Applications

The Prouhet–Thue–Morse constant occurs as the angle of the Douady–Hubbard ray at the end of the sequence of western bulbs of the Mandelbrot set. This property is tied to the nature of period doubling in the Mandelbrot set.^[2]

Notes

^ Kurt Mahler, "Arithmetische Eigenschaften der Lösungen einer Klasse von Funktionalgleichungen", Math. Annalen, t. 101 (1929), pp. 342–66.
^ Parameter Ray Atlas (2000) provides a link to the Mandelbrot set.

External links

Sloane's A010060 : Thue-Morse sequence. The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
The ubiquitous Prouhet-Thue-Morse sequence, John-Paull Allouche and Jeffrey Shallit, (undated, 2004 or earlier) provides many applications and some history
PlanetMath entry