Collineation
In projective geometry, a collineation is a one-to-one and onto map (a bijection) from one projective space to another, or from a projective space to itself, such that the images of collinear points are themselves collinear. All projective linear transformations induce a collineation. A collineation of a projective space to itself is also called an automorphism, and the set of all collineations of a space to itself form a group, called the collineation group.
Contents |
Definition
Simply, a collineation is a one-to-one map from one projective space to another, or from a projective space to itself, such that the images of collinear points are themselves collinear. One may formalize this using various ways of presenting a projective space. Also, the case of the projective line is special, and hence generally treated differently.
Linear algebra
For a projective space defined in terms of linear algebra (as the projectivization of a vector space), a collineation is a map between the projective spaces that is order-preserving with respect to inclusion of subspaces; this is also referred to as a projectivity.
Formally, let V be a vector space over a field K and W a vector space over a field L. Consider the projective spaces PG(V) and PG(W). Call D(V) and D(W) the set of subspaces of V and W respectively. A collineation from PG(V) to PG(W) is a map α : D(V) → D(W), such that:
- α is a bijection.
- A ⊆ B ↔ Aα ⊆ Bα for all A, B in D(V).
Axiomatically
Given a projective space defined axiomatically in terms of an incidence structure (a set of points P, lines L, and an incidence relation I specifying which points lie on which lines, satisfying certain axioms), a collineation between projective spaces thus defined then being a bijective function f between the sets of points and a bijective function g between the set of lines, preserving the incidence relation.[note 1]
Every projective space of dimension greater than or equal to three is isomorphic to the projectivization of a linear space over a division ring, so in these dimensions this definition is no more general than the linear-algebraic one above, but in dimension two there are other projective planes, namely the non-Desarguesian planes, and this definition allows one to define a map of such projective planes.
For dimension one, any set of points lying on a single projective line defines a projective space, though the resulting notion of collineation is just any bijection of the set.
Collineations of the projective line
For a projective space of dimension one (a projective line; the projectivization of a vector space of dimension two), all points are collinear, so the collineation group is exactly the symmetric group of the points of the projective line. This is different from the behavior in higher dimensions, and thus alternatively, one can give a more restrictive definition, so that the fundamental theorem of projective geometry holds.
In this definition, when V has dimension two, a collineation from PG(V) to PG(W) is a map α : D(V) → D(W), such that :
- 0 is mapped onto the trivial subspace of W.
- V is mapped onto W.
- There is a nonsingular semilinear map β from V to W such that, for all v in V,
This last requirement ensures that collineations are all semilinear maps.
Types
The main examples of collineations are projective linear transformations (also known as homographies) and automorphic collineations. For projective spaces coming from a linear space, the fundamental theorem of projective geometry states that all collineations are a combination of these, as described below.
A duality is a collineation from a projective space onto its dual space, taking points to hyperplanes (and vice versa) and preserving incidence. A correlation is a duality from a projective space onto itself (this implies that the space is self-dual). A polarity is an involutory correlation.
Projective linear transformations
Projective linear transformations (homographies) are collineations (planes in a vector space correspond to lines in the associated projective space, and linear transformations map planes to planes, so projective linear transformations map lines to lines), but in general not all collineations are projective linear transformations – PGL is in general a proper subgroup of the collineation group.
Automorphic collineations
An automorphic collineation is a map that, in coordinates, is a field automorphism applied to the coordinates.
Fundamental theorem of projective geometry
Briefly, every collineation is the product of a homography (a projective linear transformation) and an automorphic collineation. More precisely, the collineation group is the projective semilinear group, which is the semidirect product of homographies by automorphic collineations – assuming 
In particular, the collineations of PG(2, R) are exactly the homographies, as Gal(R/Q) is trivial.
Suppose φ is a semilinear nonsingular map from V to W, with the dimension of V at least three. Define α : D(V) → D(W) by saying that Zα = { φ(z) | z ∈ Z } for all Z in D(V). As φ is semilinear, one easily checks that this map is properly defined, and further more, as φ is not singular, it is bijective. It is obvious now that α is a collineation. We say α is induced by φ.
The fundamental theorem of projective geometry states the converse:
Suppose V is a vector space over a field K with dimension at least three, W is a vector space over a field L, and α is a collineation from PG(V) to PG(W). This implies K and L are isomorphic fields, V and W have the same dimension, and there is a semilinear map φ such that φ induces α.
For 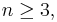 the collineation group is the projective semilinear group,
the collineation group is the projective semilinear group, 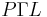 – this is PGL, twisted by field automorphisms; formally, the semidirect product
– this is PGL, twisted by field automorphisms; formally, the semidirect product  where k is the prime field for K.
where k is the prime field for K.
Linear structure
Thus for K a prime field (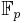 or
or 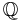 ), we have
), we have 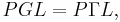 but for K not a prime field (such as
but for K not a prime field (such as  for
for 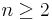 or
or 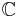 ), the projective linear group is in general a proper subgroup of the collineation group, which can be thought of as "transformations preserving a projective semi-linear structure". Correspondingly, the quotient group
), the projective linear group is in general a proper subgroup of the collineation group, which can be thought of as "transformations preserving a projective semi-linear structure". Correspondingly, the quotient group 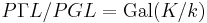 corresponds to "choices of linear structure", with the identity (base point) being the existing linear structure. Given a projective space without an identification as the projectivization of a linear space, there is no natural isomorphism between the collineation group and PΓL, and the choice of a linear structure (realization as projectivization of a linear space) corresponds to a choice of subgroup
corresponds to "choices of linear structure", with the identity (base point) being the existing linear structure. Given a projective space without an identification as the projectivization of a linear space, there is no natural isomorphism between the collineation group and PΓL, and the choice of a linear structure (realization as projectivization of a linear space) corresponds to a choice of subgroup 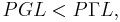 these choices forming a torsor over
these choices forming a torsor over 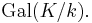
See also
Notes
- ^ "Preserving the incidence relation" means that if point p is on line l then
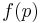 is in
is in 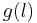 ; formally, if
; formally, if  then
then 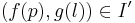 .
.
External links
|
|||||||||||
