Potential evaporation

Potential evaporation or potential evapotranspiration (PET) is defined as the amount of evaporation that would occur if a sufficient water source were available. If the actual evapotranspiration is considered the net result of atmospheric demand for moisture from a surface and the ability of the surface to supply moisture, then PET is a measure of the demand side. Surface and air temperatures, insolation, and wind all affect this. A dryland is a place where annual potential evaporation exceeds annual precipitation.

1 Estimates of potential evaporation (mm)
2 See also
3 References
4 External links

Estimates of potential evaporation (mm)

Thornthwaite equation (1948)

$PET = 1.6 \left(\frac{L}{12}\right)\left(\frac{N}{30}\right)\left(\frac{10\, T_a}{I}\right)^\alpha$

Where

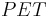$PET$ is the estimated potential evapotranspiration (cm/month)

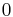$T_a$ is the average daily temperature (degrees Celsius; if this is negative, use $0$ ) of the month being calculated

$N$ is the number of days in the month being calculated

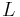$L$ is the average day length (hours) of the month being calculated

$\alpha = (6.75 \times 10^{-7}) I^3 - (7.71 \times 10^{-5}) I^2 %2B (1.792 \times 10^{-2}) I %2B 0.49239$

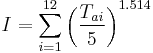$I = \sum_{i=1}^{12} \left(\frac{T_{ai}}{5}\right)^{1.514}$ is a heat index which depends on the 12 monthly mean temperatures $T_{ai}$ .^[1]

Somewhat modified forms of this equation appear in later publications (1955 and 1957) by Thornthwaite and Mather. ^[2]

Penman equation (1948)

Penman-Monteith equation (1965)

References

^ Thornthwaite, C. W. (1948). "An approach toward a rational classification of climate". Geographical Review 38 (1): 55–94. doi:10.2307/210739. http://www.unc.edu/courses/2007fall/geog/801/001/www/ET/Thornthwaite48-GeogrRev.pdf
^ Black, Peter E. (2007). "Revisiting the Thornthwaite and Mather water balance". Journal of the American Water Resources Association 43 (6): 1604–1605. doi:10.1111/j.1752-1688.2007.00132.x. http://www.watershedhydrology.com/pdf/T&M%20Revisited.pdf

Penman, H.L. (1948). "Natural evaporation from open water, bare soil, and grass". Proc. Roy. Soc. (London, U.K.) A193 (1032): 120–145. Bibcode 1948RSPSA.193..120P. doi:10.1098/rspa.1948.0037.
Brutsaert, W.H. (1982). Evaporation into the Atmosphere: theory, history, and applications. Dortrecht, Holland: D. Reidel. ISBN 9027712476.
Bonan, Gordon (2002). Ecological Climatology. Cambridge, U.K.: CUP. ISBN 978-0-521-80476-0.

External links

ag.arizona.edu Global map of potential evaporation.