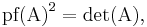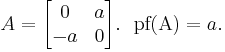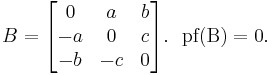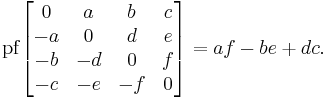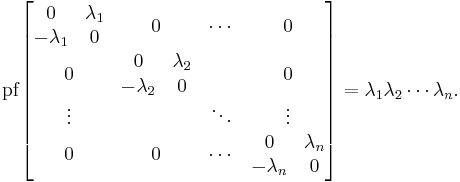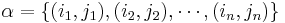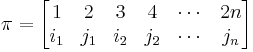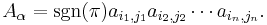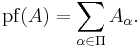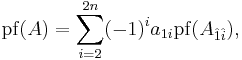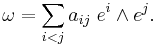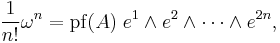Pfaffian
In mathematics, the determinant of a skew-symmetric matrix can always be written as the square of a polynomial in the matrix entries. This polynomial is called the Pfaffian of the matrix, The term Pfaffian was introduced by Cayley (1852) who named them after Johann Friedrich Pfaff. The Pfaffian is nonvanishing only for 2n × 2n skew-symmetric matrices, in which case it is a polynomial of degree n.
Explicitly, for a skew-symmetric matrix A,
which was first proved by Thomas Muir in 1882 (Muir 1882).
Contents |
Examples
(3 x 3 is odd, so Pfaffian of B is 0)
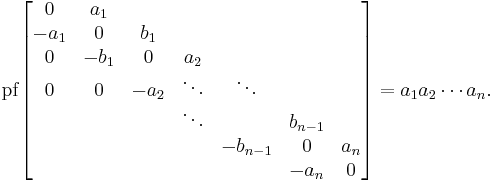
The Pfaffian of a 2n × 2n skew-symmetric tridiagonal matrix is given as
which contains the important case of a 2n × 2n skew-symmetric matrix with 2 × 2 blocks on the diagonal:
(Note that any skew-symmetric matrix can be reduced to this form, see Spectral theory of a skew-symmetric matrix)
Formal definition
Let A = {ai,j} be a 2n × 2n skew-symmetric matrix. The Pfaffian of A is defined by the equation
where S2n is the symmetric group and sgn(σ) is the signature of σ.
One can make use of the skew-symmetry of A to avoid summing over all possible permutations. Let Π be the set of all partitions of {1, 2, …, 2n} into pairs without regard to order. There are (2n − 1)!! such partitions. An element α ∈ Π can be written as
with ik < jk and  . Let
. Let
be the corresponding permutation. Given a partition α as above, define
The Pfaffian of A is then given by
The Pfaffian of a n×n skew-symmetric matrix for n odd is defined to be zero, as the determinant of an odd skew-symmetric matrix is zero, since for a skew-symmetric matrix, 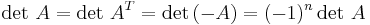 , and for n odd, this implies
, and for n odd, this implies 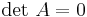 .
.
Recursive definition
By convention, the Pfaffian of the 0×0 matrix is equal to one. The Pfaffian of a skew-symmetric 2n×2n matrix A with n>0 can be computed recursively as
where 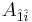 denotes the matrix A with both the first and i-th rows and columns removed.
denotes the matrix A with both the first and i-th rows and columns removed.
Alternative definitions
- One can associate to any skew-symmetric 2n×2n matrix A ={aij} a bivector
where {e1, e2, …, e2n} is the standard basis of R2n. The Pfaffian is then defined by the equation
here ωn denotes the wedge product of n copies of ω with itself.
Identities
For a 2n × 2n skew-symmetric matrix A
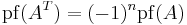 .
.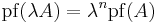 .
. .
.
For an arbitrary 2n × 2n matrix B,
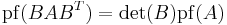 .
.
For a block-diagonal matrix
-
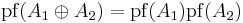 .
.
For an arbitrary n × n matrix M:
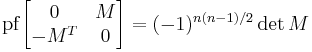 .
.
If A depends on some variable xi, then the gradient of Pfaffian is given by
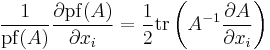 ,
,
and the Hessian of Pfaffian is given by
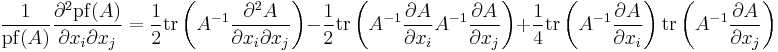 .
.
Properties
Pfaffians have the following properties similar to that of determinants
- Multiplication of a row and a column by a constant is equivalent to multiplication of Pfaffian by the same constant.
- Simultaneous interchange of two different rows and corresponding columns changes the sign of Pfaffian.
- A multiple of a row and corresponding column added to another row and corresponding column does not change the value of Pfaffian.
These properties can be derived from the identity 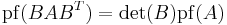 .
.
Applications
- The Pfaffian is an invariant polynomial of a skew-symmetric matrix under a a proper orthogonal change of basis. As such, it is important in the theory of characteristic classes. In particular, it can be used to define the Euler class of a Riemannian manifold which is used in the generalized Gauss-Bonnet theorem.
- The number of perfect matchings in a planar graph is given by a Pfaffian, hence is polynomial time computable via the FKT algorithm. This is surprising given that for general graphs, the problem is very difficult (so called #P-complete). This result is used to calculate the number of domino tilings of a rectangle, the partition function of Ising models in physics, or of Markov random fields in machine learning (Globerson & Jaakkola 2007;Schraudolph & Kamenetsky 2009), where the underlying graph is planar. It is also used to derive efficient algorithms for some otherwise seemingly intractable problems, including the efficient simulation of certain types of restricted quantum computation. Read Holographic algorithm for more information.
See also
References
- Cayley, Arthur (1852). "On the theory of permutants". Cambridge and Dublin Mathematical Journal VII: 40–51. http://www.archive.org/stream/collectedmathema02cayluoft#page/16/mode/2up Reprinted in Collected mathematical papers, volume 2.
- Kasteleyn, P. W. (1961). "The statistics of dimers on a lattice. I. The number of dimer arrangements on a quadratic lattice". Physica 27 (12): 1209–1225. doi:10.1016/0031-8914(61)90063-5.
- Propp, James (2004). ""Lambda-determinants and domino-tilings"". arXiv:math.CO/0406301.
- Globerson, Amir; Jaakkola, Tommi (2007). "Approximate inference using planar graph decomposition". Advances in Neural Information Processing Systems 19. MIT Press. http://books.nips.cc/papers/files/nips19/NIPS2006_0703.pdf.
- Schraudolph, Nicol; Kamenetsky, Dmitry (2009). "Efficient exact inference in planar Ising models". Advances in Neural Information Processing Systems 21. MIT Press. http://books.nips.cc/papers/files/nips21/NIPS2008_0401.pdf.
- Jeliss, G.P.; Chapman, Robin J. (1996). "Dominizing the Chessboard". The Games and Puzzles Journal 2 (14): 204–5.
- Sellers, James A. (2002). "Domino Tilings and Products of Fibonacci and Pell numbers". Journal of Integer Sequences 5 (1): 02.1.2. http://www.cs.uwaterloo.ca/journals/JIS/VOL5/Sellers/sellers4.html.
- Wells, David (1997). The Penguin Dictionary of Curious and Interesting Numbers (revised ed.). p. 182. ISBN 0-14-026149-4.
- Muir, Thomas (1882). A Treatise on the Theory of Determinants. Macmillan and Co.. Online
- Parameswaran, S. (1954). "Skew-Symmetric Determinants". The American Mathematical Monthly 61 (2): 116. JSTOR 2307800.
External links
- Pfaffian at PlanetMath.org
- T. Jones, The Pfaffian and the Wedge Product (a demonstration of the proof of the Pfaffian/determinant relationship)
- R. Kenyon and A. Okounkov, What is ... a dimer?
- (sequence A004003 in OEIS)