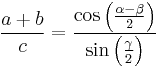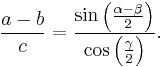Mollweide's formula
In trigonometry, Mollweide's formula, sometimes referred to in older texts as Mollweide's equations,[1] named after Karl Mollweide, is a set of two relationships between sides and angles in a triangle.[2] It can be used to check solutions of triangles.[3]
Let a, b, and c be the lengths of the three sides of a triangle. Let α, β, and γ be the measures of the angles opposite those three sides respectively. Mollweide's formula states that
and
Each of these identities uses all six parts of the triangle—the three angles and the lengths of the three sides.
See also
Notes
References
- H. Arthur De Kleine, "Proof Without Words: Mollweide's Equation", Mathematics Magazine, volume 61, number 5, page 281, December, 1988.