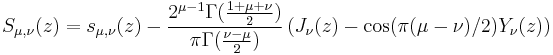Lommel function
The Lommel differential equation is an inhomogeneous form of the Bessel differential equation:
Two solutions are given by the Lommel functions sμ,ν(z) and Sμ,ν(z), introduced by Eugen von Lommel (1880),
where Jν(z) is a Bessel function of the first kind, and Yν(z) a Bessel function of the second kind.
See also
References
- Erdélyi, Arthur; Magnus, Wilhelm; Oberhettinger, Fritz; Tricomi, Francesco G. (1953), Higher transcendental functions. Vol II, McGraw-Hill Book Company, Inc., New York-Toronto-London, MR0058756, http://apps.nrbook.com/bateman/Vol2.pdf
- Lommel, E. (1875), "Ueber eine mit den Bessel'schen Functionen verwandte Function", Math. Ann. 9 (3): 425–444, doi:10.1007/BF01443342
- Lommel, E. (1880), "Zur Theorie der Bessel'schen Funktionen IV", Math. Ann. 16 (2): 183–208, doi:10.1007/BF01446386
- Paris, R. B. (2010), "Lommel function", in Olver, Frank W. J.; Lozier, Daniel M.; Boisvert, Ronald F. et al., NIST Handbook of Mathematical Functions, Cambridge University Press, ISBN 978-0521192255, MR2723248, http://dlmf.nist.gov/11.9
- Solomentsev, E.D. (2001), "Lommel function", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1556080104, http://www.encyclopediaofmath.org/index.php?title=l/l060800
External links
- Weisstein, Eric W. "Lommel Differential Equation." From MathWorld—A Wolfram Web Resource.
- Weisstein, Eric W. "Lommel Function." From MathWorld—A Wolfram Web Resource.
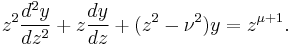
![s_{\mu,\nu}(z) = \frac{1}{2} \pi \left[ Y_\nu (z) \int_0^z z^\mu J_\nu (z)\, dz - J_\nu (z) \int_0^z z^\mu Y_\nu (z)\, dz\right]](/2012-wikipedia_en_all_nopic_01_2012/I/ca44e20fee4c3d3c4a8d41185b0ae3c4.png)