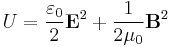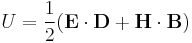Energy density
Energy density is a term used for the amount of energy stored in a given system or region of space per unit volume. Often only the useful or extractable energy is quantified, which is to say that chemically inaccessible energy such as rest mass energy is ignored.[1] Quantified energy is energy that has some sort of, as the name suggests, quantified magnitude with related units.
For fuels, the energy per unit volume is sometimes a useful parameter. Comparing, for example, the effectiveness of hydrogen fuel to gasoline, hydrogen has a higher specific energy (energy per unit mass) than gasoline does, but, even in liquid form, a much lower volumetric energy density.
Energy per unit volume has the same physical units as pressure, and in many circumstances is an exact synonym: for example, the energy density of the magnetic field may be expressed as (and behaves as) a physical pressure, and the energy required to compress a compressed gas a little more may be determined by multiplying the difference between the gas pressure and the pressure outside by the change in volume. In short, pressure is a measure of volumetric enthalpy of a system. A pressure gradient has a potential to perform work on the surroundings by converting enthalpy until equilibrium is reached.
Contents |
Energy density in energy storage and in fuel
In energy storage applications the energy density relates the mass of an energy store to the volume of the storage facility, e.g. the fuel tank. The higher the energy density of the fuel, the more energy may be stored or transported for the same amount of volume. The energy density of a fuel per unit mass is called the specific energy of that fuel. In general an engine using that fuel will generate less kinetic energy due to inefficiencies and thermodynamic considerations—hence the specific fuel consumption of an engine will always be greater than its rate of production of the kinetic energy of motion.
The greatest energy source by far consists of mass itself. This energy, E = mc2, where m = ρV, ρ is the mass per unit volume, V is the volume of the mass itself and c is the speed of light. This energy, however, can be released only by the processes of nuclear fission, nuclear fusion, or the annihilation of some or all of the matter in the volume V by matter-antimatter collisions. Nuclear reactions cannot be realized by chemical reactions such as combustion. Although greater matter densities can be achieved, the density of a neutron star would approximate the most dense system capable of matter-antimatter annihilation possible. A black hole, although denser than a neutron star, doesn't have an equivalent anti-particle form.
If we thus consider the volume of the sphere around a proton defined by the extent of its electric field, we can get an approximate energy density for the proton, as seen in the table below.
The highest density sources of energy outside of antimatter are fusion and fission. Fusion includes energy from the sun which will be available for billions of years (in the form of sunlight) but so far (2011), sustained fusion power production continues to be elusive. Fission of U-235 in nuclear power plants will be available for some decades even though the vast supply of the element on earth is being depleted (peak uranium) - it might, however be possible at some future time to extract uranium from seawater.[2][3] Coal, gas, and petroleum are the current primary energy sources in the U.S.[4] but have a much lower energy density. Burning local biomass fuels supplies household energy needs (cooking fires, oil lamps, etc.) worldwide.
Energy density (how much energy you can carry) does not tell you about energy conversion efficiency (net output per input) or embodied energy (what the energy output costs to provide, as harvesting, refining, distributing, and dealing with pollution all use energy). Like any process occurring on a large scale, intensive energy use impacts the world. For example, climate change, nuclear waste storage, and deforestation may be some of the consequences of supplying our growing energy demands from fossil fuels, nuclear fission, or biomass.
No single energy storage method boasts the best in specific power, specific energy, and energy density. Peukert's Law describes how the amount of useful energy that can be obtained (for a lead-acid cell) depends on how quickly we pull it out. To maximize both specific energy and energy density, one can compute the specific energy density of a substance by multiplying the two values together, where the higher the number, the better the substance is at storing energy efficiently.
Gravimetric and volumetric energy density of some fuels and storage technologies (modified from the Gasoline article):
- Note: Some values may not be precise because of isomers or other irregularities. See Heating value for a comprehensive table of specific energies of important fuels.
- Note: Also it is important to realise that generally the density values for chemical fuels do not include the weight of oxygen required for combustion. This is typically 2 oxygen atoms per carbon atom, and one per hydrogen atom. The atomic weight of carbon and oxygen are similar, while hydrogen is much lighter than oxygen. Figures are presented this way for those fuels where in practice air would only be drawn in locally to the burner. This explains the apparently lower energy density of materials that already include their own oxidiser (such as gunpowder and TNT), where the mass of the oxidiser in effect adds dead weight, and absorbs some of the energy of combustion to dissociate and liberate oxygen to continue the reaction. This also explains some apparent anomalies, such as the energy density of a sandwich appearing to be higher than that of a stick of dynamite.
Common energy densities
The following is a list of the energy densities of commonly used energy storage materials. Note that this list does not consider the mass of reactants commonly available such as the oxygen required for combustion or the matter necessary to annihilate with antimatter. The following unit conversions maybe helpful when considering the data in the table 1 MJ ≈ 0.28 kWh ≈ 0.37 HPh.
| Storage material | Energy type | Energy per kilogram | Energy per litre | Direct uses |
|---|---|---|---|---|
| Antimatter | Matter/Antimatter | 180 petajoules [5] | Theoretical | |
| Uranium 238 | Nuclear | 20 terajoules | Electric power plants (nuclear reactors) | |
| Hydrogen (compressed at 700 bar) | Chemical | 123 megajoules | 5.6 megajoules | Experimental automotive engines |
| Gasoline (petrol) | Chemical | 47.2 megajoules | 34 megajoules | Automotive engines |
| Diesel | Chemical | 45.4 megajoules | 38.6 megajoules | Automotive engines |
| Propane (including LPG) | Chemical | 46.4 megajoules | Cooking, home heating, automotive engines | |
| Jet fuel, Kerosene | Chemical | 43 megajoules | 33 megajoules | Jet engines |
| Fat (animal/vegetable) | Chemical | 37 megajoules | Human/animal nutrition | |
| Coal | Chemical | 24 megajoules | Electric power plants, home heating | |
| Carbohydrates (including sugars) | Chemical | 17 megajoules | Human/animal nutrition | |
| Protein | Chemical | 16.8 megajoules | Human/animal nutrition | |
| Wood | Chemical | 16.2 megajoules | Heating, outdoor cooking | |
| Lithium air battery | Electrochemical | 9 megajoules | Portable electronic devices, electric vehicles | |
| Carbon nanotube springs | Spring (device) | 7.2 megajoules [6] | Theoretical | |
| TNT | Chemical | 4.6 megajoules | Explosives | |
| Gunpowder | Chemical | 3 megajoules | Explosives | |
| Lithium battery | Electrochemical | 1.30 megajoules | Portable electronic devices, flashlights | |
| Lithium-ion battery | Electrochemical | 720 kilojoules | Laptop computers, mobile devices, some modern automotive engines | |
| Alkaline battery | Electrochemical | 590 kilojoules | Portable electronic devices, flashlights | |
| Supercapacitor (graphene/SWCNT electrode)[7] | Electrical | 560 kilojoules | Electric vehicles, DC power smoothing | |
| Sodium aqueous battery | Electrochemical | 367 kilojoules | Load levelling, power smoothing, grid storage | |
| Nickel-metal hydride battery | Electrochemical | 288 kilojoules | Portable electronic devices, flashlights | |
| Supercapacitor | Electrical | 100 kilojoules | DC Supply smoothing | |
| Lead-acid battery | Electrochemical | 100 kilojoules | Automotive engine igniton | |
| Electrostatic capacitor | Electrical | 360 joules | Electronic circuits |
| Storage device | Energy type | Energy content | Typical mass | W × H × D (mm) | Uses |
|---|---|---|---|---|---|
| Automotive battery (lead-acid) | Electrochemical | 2.6 megajoules | 15 kilograms | 230 × 180 × 185 | Automotive starter motor and accessories |
| Club sandwich (Subway 6 inch) | Chemical | 1.3 megajoules | 240 grams | 150 × ? × ? | Human nutrition |
| Alkaline "battery" (AA size) | Electrochemical | 15.4 kilojoules | 23 grams | 14.5 × 50.5 × 14.5 | Portable electronic equipment, flashlights |
| lithium-ion battery (Nokia BL-5C) | Electrochemical | 12.9 kilojoules | 18.5 grams | 54.2 × 33.8 × 5.8 | Mobile phones |
Energy densities ignoring external components
This table lists energy densities of systems that require external components, such as oxidisers or a heat sink or source. These figures do not take into account the mass and volume of the required components as they are assumed to be freely available and present in the atmosphere. Such systems cannot be compared with self-contained systems.
| Storage type | Specific energy (MJ/kg) | Energy density (MJ/L) | Peak recovery efficiency % | Practical recovery efficiency % |
|---|---|---|---|---|
| Planck energy density | 8.99 × 1010 | 4.633016 × 10104 | ||
| Hydrogen, liquid[8] | 141.86 | 8.491 | ||
| Hydrogen, at 690 bar and 15ºC[8] | 141.86 | 4.5 | ||
| Hydrogen, gas[8] | 141.86 | 0.01005 | ||
| Beryllium | 67.6 | 125.1 | ||
| Lithium borohydride | 65.2 | 43.4 | ||
| Boron[9] | 58.9 | 137.8 | ||
| Methane (1.013 bar, 15°C) | 55.6 | 0.0378 | ||
| Natural gas | 53.6[10] | 0.0364 | ||
| LNG (NG at −160°C) | 53.6[10] | 22.2 | ||
| CNG (NG compressed to 250 bar/~3,600 psi) | 53.6[10] | 9 | ||
| LPG propane[11] | 49.6 | 25.3 | ||
| LPG butane[11] | 49.1 | 27.7 | ||
| Gasoline (petrol)[11] | 46.4 | 34.2 | ||
| Polypropylene plastic | 46.4[12] | 41.7 | ||
| Polyethylene plastic | 46.3[12] | 42.6 | ||
| Crude oil (according to the definition of ton of oil equivalent) | 46.3 | 37[10] | ||
| Diesel fuel/residential heating oil [11] | 46.2 | 37.3 | ||
| 100LL Avgas | 44.0[13] | 31.59 | ||
| Gasohol E10 (10% ethanol 90% gasoline by volume) | 43.54 | 33.18 | ||
| Lithium | 43.1 | 23.0 | ||
| Jet A aviation fuel[14]/kerosene | 42.8 | 33 | ||
| Biodiesel oil (vegetable oil) | 42.20 | 33 | ||
| DMF (2,5-dimethylfuran) | 42[15] | 37.8 | ||
| Polystyrene plastic | 41.4[12] | 43.5 | ||
| Body fat metabolism | 38 | 35 | 22[16] | |
| Butanol | 36.6 | 29.2 | ||
| Gasohol E85 (85% ethanol 10% gasoline by volume) | 33.1 | 25.65 | ||
| Graphite | 32.7 | 72.9 | ||
| Coal, anthracite[17] | 32.5 | 72.4 | 36 | |
| Silicon [18] | 32.2 | 75.1 | ||
| Aluminum | 31.0 | 83.8 | ||
| Ethanol | 30 | 24 | ||
| Polyester plastic | 26.0 [12] | 35.6 | ||
| Magnesium | 24.7 | 43.0 | ||
| Coal, bituminous[17] | 24 | 20 | ||
| PET plastic | 23.5 (impure)[19] | |||
| Methanol | 19.7 | 15.6 | ||
| Hydrazine (toxic) combusted to N2+H2O | 19.5 | 19.3 | ||
| Liquid ammonia (combusted to N2+H2O) | 18.6 | 11.5 | ||
| PVC plastic (improper combustion toxic) | 18.0[12] | 25.2 | ||
| Wood[20] | 18.0 | |||
| Peat briquette [21] | 17.7 | |||
| Sugars, carbohydrates, and protein metabolism | 17 | 26.2(dextrose) | 22[22] | |
| Calcium | 15.9 | 24.6 | ||
| Glucose | 15.55 | 23.9 | ||
| Dry cowdung and cameldung | 15.5[23] | |||
| Coal, lignite | 14.0 | |||
| Sodium (burned to wet sodium hydroxide) | 13.3 | 12.8 | ||
| Sod peat | 12.8 | |||
| Nitromethane | 11.3 | |||
| Sulfur (burned to Sulfur Dioxide)[24] | 9.23 | 19.11 | ||
| Sodium (burned to dry sodium oxide) | 9.1 | 8.8 | ||
| Battery, lithium-air rechargeable | 9.0[25] | |||
| Household waste | 8.0[26] | |||
| Zinc | 5.3 | 38.0 | ||
| Iron (burned to iron(III) oxide) | 5.2 | 40.68 | ||
| Teflon plastic (combustion toxic, but flame retardant) | 5.1 | 11.2 | ||
| Iron (burned to iron(II) oxide) | 4.9 | 38.2 | ||
| ANFO | 3.7 | |||
| Battery, zinc-air[27] | 1.59 | 6.02 | ||
| Liquid nitrogen | 0.77[28] | 0.62 | ||
| Compressed air at 300 bar (potential energy) | 0.5 | 0.2 | >50% | |
| Latent heat of fusion of ice (thermal) | 0.335 | 0.335 | ||
| Water at 100 m dam height (potential energy) | 0.001 | 0.001 | 85-90% | |
| Storage type | Energy density by mass (MJ/kg) | Energy density by volume (MJ/L) | Peak recovery efficiency % | Practical recovery efficiency % |
Divide Joule meter−3 with 109 to get MJ L−1.
Energy density of electric and magnetic fields
Electric and magnetic fields store energy. In a vacuum, the (volumetric) energy density (in SI units) is given by
where E is the electric field and B is the magnetic field. In the context of magnetohydrodynamics, the physics of conductive fluids, the magnetic energy density behaves like an additional pressure that adds to the gas pressure of a plasma.
In normal (linear) substances, the energy density (in SI units) is
where D is the electric displacement field and H is the magnetizing field.
Energy density of empty space
In physics, "vacuum energy" or "zero-point energy" is the volumetric energy density of empty space. More recent developments have expounded on the concept of energy in empty space.
Modern physics is commonly classified into two fundamental theories: quantum field theory and general relativity. Quantum field theory takes quantum mechanics and special relativity into account, and it's a theory of all the forces and particles except gravity. General relativity is a theory of gravity, but it is incompatible with quantum mechanics. Currently these two theories have not yet been reconciled into one unified description, though research into "quantum gravity" and, more recently, stochastic electrodynamics, seeks to bridge this divide.
In general relativity, the cosmological constant is proportional to the energy density of empty space, and can be measured by the curvature of space.
Quantum field theory considers the vacuum ground state not to be completely empty, but to consist of a seething mass of virtual particles and fields. These fields are quantified as probabilities—that is, the likelihood of manifestation based on conditions. Since these fields do not have a permanent existence, they are called vacuum fluctuations. In the Casimir effect, two metal plates can cause a change in the vacuum energy density between them which generates a measurable force.
Some believe that vacuum energy might be the "dark energy" (also called Quintessence) associated with the cosmological constant in general relativity, thought to be similar to a negative force of gravity (or antigravity). Observations that the expanding universe appears to be accelerating seem to support the cosmic inflation theory—first proposed by Alan Guth in 1981—in which the nascent universe passed through a phase of exponential expansion driven by a negative vacuum energy density (positive vacuum pressure).
See also
- Power density and specifically
- Orders of magnitude (specific energy density)
- Figure of merit
- Energy content of biofuel
- Heat of combustion
- Heating value
- Rechargeable battery
- Specific impulse
- Vacuum energy
External references
Zero point energy
- Eric Weisstein's world of physics: energy density[29]
- Baez physics: Is there a nonzero cosmological constant?[30]
- Introductory review of cosmic inflation[31]
- An exposition to inflationary cosmology[32]
Density data
- ^ "Aircraft Fuels." Energy, Technology and the Environment Ed. Attilio Bisio. Vol. 1. New York: John Wiley and Sons, Inc., 1995. 257–259
- “Fuels of the Future for Cars and Trucks” - Dr. James J. Eberhardt - Energy Efficiency and Renewable Energy, U.S. Department of Energy - 2002 Diesel Engine Emissions Reduction (DEER) Workshop San Diego, California - August 25–29, 2002
Energy storage
Books
- The Inflationary Universe: The Quest for a New Theory of Cosmic Origins by Alan H. Guth (1998) ISBN 0-201-32840-2
- Cosmological Inflation and Large-Scale Structure by Andrew R. Liddle, David H. Lyth (2000) ISBN 0-521-57598-2
- Richard Becker, "Electromagnetic Fields and Interactions", Dover Publications Inc., 1964
Footnotes
- ^ http://physics.nist.gov/Pubs/SP811/sec04.html
- ^ stormsmith.nl: Factsheet 4: Energy security and uranium reserves Quote: "...After about 60 years the world nuclear power system will fall off the 'Energy Cliff' - meaning that the nuclear system will consume as much energy as can be generated from the uranium fuel. Whether large and rich new uranium ore deposits will be found or not is unknown...Graph 1: Depletion of world known recoverable resources, 2006 - 2076...Net energy and the 'Energy Cliff' Graph 2: the energy cliff..."
- ^ "Facts from Cohen". Formal.stanford.edu. 2007-01-26. http://www-formal.stanford.edu/jmc/progress/cohen.html. Retrieved 2010-05-07.
- ^ "U.S. Energy Information Administration (EIA) - Annual Energy Review". Eia.doe.gov. 2009-06-26. http://www.eia.doe.gov/emeu/aer/pecss_diagram.html. Retrieved 2010-05-07.
- ^ Ken Edwards. "Propulsion and Power with Positrons. NIAC Fellows Meeting." Air Force Research Laboratory, 2004. http://www.niac.usra.edu/files/library/meetings/fellows/mar04/Edwards_Kenneth.pdf Retrieved 2011-08-31.
- ^ Wikipedia: Carbon nanotube springs. http://en.wikipedia.org/wiki/Carbon_nanotube_springs Retrieved 2011-10-21.
- ^ Quiang Cheng et.al. "Graphene and carbon nanotube composite electrodes for supercapacitors with ultra-high energy density" Phys. Chem. Chem. Phys., 2011, DOI: 10.1039/C1CP21910C. http://pubs.rsc.org/en/content/articlelanding/2011/cp/c1cp21910c
- ^ a b c Hydrogen properties Hydrogen Properties. Retrieved 2011-11-30.
- ^ "Boron: A Better Energy Carrier than Hydrogen? (28 February 2009)". Eagle.ca. http://www.eagle.ca/~gcowan/boron_blast.html#TOC. Retrieved 2010-05-07.
- ^ a b c d Envestra Limited. Natural Gas. Retrieved 2008-10-05.
- ^ a b c d IOR Energy. List of common conversion factors (Engineering conversion factors). Retrieved 2008-10-05.
- ^ a b c d e http://www.aquafoam.com/papers/selection.pdf
- ^ http://www-static.shell.com/static/aus/downloads/aviation/avgas_100ll_pds.pdf
- ^ "Energy Density of Aviation Fuel". Hypertextbook.com. http://hypertextbook.com/facts/2003/EvelynGofman.shtml. Retrieved 2010-05-07.
- ^ Nature. "Production of dimethylfuran for liquid fuels from biomass-derived carbohydrates : Abstract". Nature. http://www.nature.com/nature/journal/v447/n7147/abs/nature05923.html. Retrieved 2010-05-07.
- ^ Justin Lemire-Elmore (2004-04-13). "The Energy Cost of Electric and Human-Powered Bicycles". p. 5. http://www.ebikes.ca/sustainability/Ebike_Energy.pdf. Retrieved 2009-02-26. "properly trained athlete will have efficiencies of 22 to 26%"
- ^ a b Fisher, Juliya (2003). "Energy Density of Coal". The Physics Factbook. http://hypertextbook.com/facts/2003/JuliyaFisher.shtml. Retrieved 2006-08-25.
- ^ http://dbresearch.com/PROD/DBR_INTERNET_EN-PROD/PROD0000000000079095.pdf
- ^ "Elite_bloc.indd" (PDF). http://www.payne-worldwide.com/documents/cms/Elite_bloc_msds.pdf. Retrieved 2010-05-07.
- ^ "Biomass Energy Foundation: Fuel Densities". Woodgas.com. http://www.woodgas.com/fuel_densities.htm. Retrieved 2010-05-07.
- ^ Bord na Mona, Peat for Energy
- ^ http://www.ebikes.ca/sustainability/Ebike_Energy.pdf
- ^ "energy buffers". Home.hccnet.nl. http://home.hccnet.nl/david.dirkse/math/energy.html. Retrieved 2010-05-07.
- ^ Anne Wignall and Terry Wales. Chemistry 12 Workbook, page 138. Pearson Education NZ ISBN 978 0 582 54974 6
- ^ Mitchell, Robert R.; Betar M. Gallant; Carl V. Thompson; Yang Shao-Horn (2011). "All-carbon-nanofiber electrodes for high-energy rechargeable Li–O2 batteries". Energy & Environmental Science 4: 2952–2958. doi:10.1039/C1EE01496J. http://pubs.rsc.org/en/content/articlelanding/2011/ee/c1ee01496j.
- ^ David E. Dirkse. energy buffers. "household waste 8..11 MJ/kg"
- ^ "Technical bulletin on Zinc-air batteries". Duracell. http://www.duracell.com/oem/primary/Zinc/zinc_air_tech.asp. Retrieved 2009-04-21.
- ^ C. Knowlen, A.T. Mattick, A.P. Bruckner and A. Hertzberg, "High Efficiency Conversion Systems for Liquid Nitrogen Automobiles", Society of Automotive Engineers Inc, 1988.
- ^ "Energy Density - from Eric Weisstein's World of Physics". Scienceworld.wolfram.com. http://scienceworld.wolfram.com/physics/EnergyDensity.html. Retrieved 2010-05-07.
- ^ What's the Energy Density of the Vacuum?
- ^ Shinji Tsujikawa (2003-04-28). "Introductory review of cosmic inflation". arXiv:hep-ph/0304257 [hep-ph].
- ^ Scott Watson (2000). "An Exposition on Inflationary Cosmology". arXiv:astro-ph/0005003 [astro-ph].