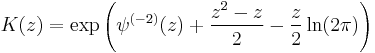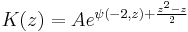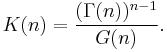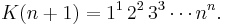K-function
For the k-function, see Bateman function.
In mathematics, the K-function, typically denoted K(z), is a generalization of the hyperfactorial to complex numbers, similar to the generalization of the factorial to the Gamma function.
Formally, the K-function is defined as
It can also be given in closed form as
where ζ'(z) denotes the derivative of the Riemann zeta function, ζ(a,z) denotes the Hurwitz zeta function and
Another expression using polygamma function is[1]
Or using alternative generalization of Polygamma function[2]:
- where A is Glaisher constant.
The K-function is closely related to the Gamma function and the Barnes G-function; for natural numbers n, we have
More prosaically, one may write
The first values are
- 1, 4, 108, 27648, 86400000, 4031078400000, 3319766398771200000, ... ((sequence A002109 in OEIS)).
References
- ^ Victor S. Adamchik. PolyGamma Functions of Negative Order
- ^ Olivier Espinosa Victor H. Moll. A Generalized polygamma function. Integral Transforms and Special Functions Vol. 15, No. 2, April 2004, pp. 101–115
External links
- Weisstein, Eric W., "K-Function" from MathWorld.
![K(z)=(2\pi)^{(-z%2B1)/2} \exp\left[\begin{pmatrix} z\\ 2\end{pmatrix}%2B\int_0^{z-1} \ln(t!)\,dt\right].](/2012-wikipedia_en_all_nopic_01_2012/I/116ac987e7de7c61173b4a09a7ecffa2.png)
![K(z)=\exp\left[\zeta^\prime(-1,z)-\zeta^\prime(-1)\right]](/2012-wikipedia_en_all_nopic_01_2012/I/8a540da338ac595b899a0d2015882ac3.png)
![\zeta^\prime(a,z)\ \stackrel{\mathrm{def}}{=}\ \left[\frac{d\zeta(s,z)}{ds}\right]_{s=a}.](/2012-wikipedia_en_all_nopic_01_2012/I/2b4d4c6cadc0a5e6a8e5c0ca6f2b0131.png)