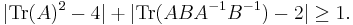Jørgensen's inequality

In the mathematical theory of Kleinian groups, Jørgensen's inequality is an inequality involving the traces of elements of a Kleinian group, proved by Troels Jørgensen (1976).

The inequality states that if A and B generate a non-elementary discrete subgroup of SL₂(C), then

$|\text{Tr}(A)^2 -4| %2B |\text{Tr}(ABA^{-1}B^{-1})-2|\ge 1. \,$

References

Jørgensen, Troels (1976), "On discrete groups of Möbius transformations", American Journal of Mathematics 98 (3): 739–749, ISSN 0002-9327, JSTOR 2373814, MR 0427627