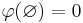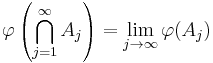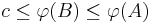Inner measure
In mathematics, in particular in measure theory, an inner measure is a function on the set of all subsets of members of a given σ-algebra with values in the extended real numbers satisfying some technical conditions. Intuitively, the inner measure of a set is a lower bound of the size of that set.
Contents |
Definition
An inner measure is a function
defined on all subsets of a set X, that satisfies the following conditions:
- Null empty set: The empty set has zero inner measure (see also: measure zero).
- Superadditive: For any disjoint sets A and B,
- Limits of decreasing towers: For any sequence {Aj} of sets such that
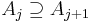 for each j and
for each j and 
- Infinity must be approached: If
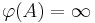 for a set A then for every positive number c, there exists a B which is a subset of A such that,
for a set A then for every positive number c, there exists a B which is a subset of A such that,
The inner measure induced by a measure
Let Σ be a σ-algebra over a set X and μ be a measure on Σ. Then the inner measure μ* induced by μ is defined by
Essentially μ* gives a lower bound of the size of any set by insuring it is at least as big as the μ-measure of any of its Σ-measurable subsets. Even though the set function μ* is usually not a measure, μ* shares the following properties with measures:
-
- μ*(∅)=0,
- μ* is non-negative,
- If E ⊆ F then μ*(E) ≤ μ*(F).
Measure completion
Induced inner measures are often used in combination with outer measures to extend a measure to a larger σ-algebra. If μ is a measure defined on σ-algebra Σ over X and μ* and μ* are corresponding induced outer and inner measures, then the sets T ∈ 2X such that μ*(T) = μ*(T) form a σ-algebra 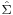 with
with 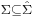 . The set function μ̂ defined by
. The set function μ̂ defined by
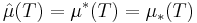 ,
,
for all T ∈ 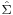 is a measure on
is a measure on 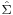 known as the completion of μ.
known as the completion of μ.
References
- Halmos, Paul R., Measure Theory, D. Van Nostrand Company, Inc., 1950, pp. 58.
- A. N. Kolmogorov & S. V. Fomin, translated by Richard A. Silverman, Introductory Real Analysis, Dover Publications, New York, 1970, ISBN 0-486-61226-0 (Chapter 7)
![\varphi: 2^X \rightarrow [0, \infty],](/2012-wikipedia_en_all_nopic_01_2012/I/08e8360cc5bff864099b7635f506ea2d.png)