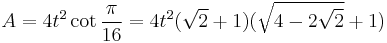Hexadecagon
| Regular hexadecagon | |
|---|---|
A regular hexadecagon |
|
| Type | general type of this shape |
| Edges and vertices | 16 |
| Schläfli symbol | {16} t{8} |
| Coxeter–Dynkin diagrams | |
| Symmetry group | Dihedral (D16) |
| Internal angle (degrees) | 157.5° |
| Properties | convex, cyclic, equilateral, isogonal, isotoxal |
In mathematics, a hexadecagon (sometimes called a hexakaidecagon) is a polygon with 16 sides and 16 vertices.
Contents |
Regular hexadecagon
A regular hexadecagon is constructible with a compass and straightedge.
Each angle of a regular hexadecagon is 157.5 degrees, and the total angle measure of any hexadecagon is 2520 degrees.
Construction
A regular hexadecagon is constructible using compass and straightedge:
Construction of a regular hexadecagon
Area
The area of a regular hexadecagon is: (with t = edge length)
Petrie polygons
The regular hexadecagon is the Petrie polygon for many higher dimensional polytopes, shown in these skew orthogonal projections, including:
External links
- Weisstein, Eric W., "Hexadecagon" from MathWorld.
|
|||||||||||||||||