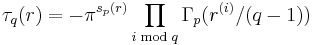Gross–Koblitz formula
In mathematics the Gross–Koblitz formula, introduced by Gross and Koblitz (1979) expresses a Gauss sum using a product of values of the p-adic gamma function. It is an analog of the Chowla–Selberg formula for the usual gamma function. It implies the Hasse–Davenport relation and generalizes the Stickelberger theorem. Boyarsky (1980) gave another proof of the Gross–Koblitz formula using Dwork's work, and Robert (2001) gave an elementary proof.
Statement
The Gross–Koblitz formula states that the Gauss sum τ can be given in terms of the p-adic gamma function Γp by
where
- q is a power pf of a prime p
- r is an integer with 0 ≤ r < q–1
- r(i) is the integer whose base p expansion is a cyclic permutation of the first f digits of r.
- sp(r) is the sum of the digits of r in base p
- τ is the Gauss sum
 where the sum is over roots of 1 in the extension Qp(π)
where the sum is over roots of 1 in the extension Qp(π)
- Γp is the p-adic gamma function.
- π satisfies πp–1 = –p
- ζπ is the pth root of 1 congruent to 1+π mod π2
References
- Boyarsky, Maurizio (1980), "p-adic gamma functions and Dwork cohomology", Transactions of the American Mathematical Society 257 (2): 359–369, doi:10.2307/1998301, ISSN 0002-9947, MR552263, http://dx.doi.org/10.2307/1998301
- Gross, Benedict H.; Koblitz, Neal (1979), "Gauss sums and the p-adic Γ-function", Annals of Mathematics. Second Series 109 (3): 569–581, doi:10.2307/1971226, ISSN 0003-486X, MR534763, http://dx.doi.org/10.2307/1971226
- Robert, Alain M. (2001), "The Gross-Koblitz formula revisited", Rendiconti del Seminario Matematico della Università di Padova. The Mathematical Journal of the University of Padova 105: 157–170, ISSN 0041-8994, MR1834987, http://www.numdam.org/item?id=RSMUP_2001__105__157_0