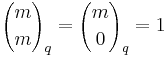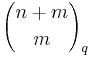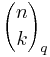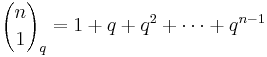Gaussian binomial coefficient
In mathematics, the Gaussian binomial coefficients (also called Gaussian coefficients, Gaussian polynomials, or q-binomial coefficients) are q-analogs of the binomial coefficients.
Contents |
Definition
The Gaussian binomial coefficients are defined by
where m and r are non-negative integers. For r = 0 the value is 1 since numerator and denominator are both empty products. Although the formula in the first clause appears to involve a rational function, it actually designates a polynomial, because the division is exact in Z[q]. Note that the formula can be applied for r = m + 1, and gives 0 due to a factor 1 − q0 = 0 in the numerator, in accordance with the second clause (for even larger r the factor 0 remains present in the numerator, but its further factors would involve negative powers of q, whence explicitly stating the second clause is preferable). All of the factors in numerator and denominator are divisible by 1 − q, with as quotient a q number:
dividing out these factors gives the equivalent formula
which makes evident the fact that substituting q = 1 into 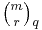 gives the ordinary binomial coefficient
gives the ordinary binomial coefficient 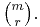 In terms of the q factorial
In terms of the q factorial ![[n]_q!=[1]_q[2]_q\cdots[n]_q](/2012-wikipedia_en_all_nopic_01_2012/I/41e9cca30f02fa0413aee6e28f0fc730.png) , the formula can be stated as
, the formula can be stated as
a compact form (often given as only definition), which however hides the presence of many common factors in numerator and denominator. This form does make obvious the symmetry 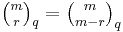 for r ≤ m.
for r ≤ m.
Unlike the ordinary binomial coefficient, the Gaussian binomial coefficient has finite values for 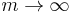 :
:
Examples
Properties
Like the ordinary binomial coefficients, the Gaussian binomial coefficients are center-symmetric i.e. invariant under the reflection 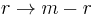 :
:
In particular,
The name Gaussian binomial coefficient stems from the fact that their evaluation at q = 1 is
for all m and r.
The analogs of Pascal identities for the Gaussian binomial coefficients are
and
There are analogs of the binomial formula, and of Newton's generalized version of it for negative integer exponents, although for the former the Gaussian binomial coefficients themselves do not appear as coefficients:
and
which, for  become:
become:
and
The first Pascal identity allows one to compute the Gaussian binomial coefficients recursively (with respect to m ) using the initial "boundary" values
and also incidentally shows that the Gaussian binomial coefficients are indeed polynomials (in q). The second Pascal identity follows from the first using the substitution 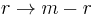 and the invariance of the Gaussian binomial coefficients under the reflection
and the invariance of the Gaussian binomial coefficients under the reflection 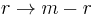 . Both Pascal identities together imply
. Both Pascal identities together imply
which leads (when applied iteratively for m, m − 1, m − 2,....) to an expression for the Gaussian binomial coefficient as given in the definition above.
Applications
Gaussian binomial coefficients occur in the counting of symmetric polynomials and in the theory of partitions. The coefficient of qr in
is the number of partitions of r with m or fewer parts each less than or equal to n. Equivalently, it is also the number of partitions of r with n or fewer parts each less than or equal to m.
Gaussian binomial coefficients also play an important role in the enumerative theory of symmetric spaces defined over a finite field. In particular, for every finite field Fq with q elements, the Gaussian binomial coefficient
counts the number vn,k;q of different k-dimensional vector subspaces of an n-dimensional vector space over Fq (a Grassmannian). For example, the Gaussian binomial coefficient
is the number of different lines in Fqn (a projective space).
In the conventions common in applications to quantum groups, a slightly different definition is used; the quantum binomial coefficient there is
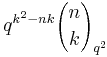 .
.
This version of the quantum binomial coefficient is symmetric under exchange of 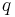 and
and 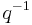 .
.
Triangles
The Gaussian binomial coefficients can be arranged in a triangle for each q, which is Pascal's triangle for q=1.
Read line by line these triangles form the following sequences in the OEIS:
- A022166 for q= 2
- A022167 for q= 3
- A022168 for q= 4
- A022169 for q= 5
- A022170 for q= 6
- A022171 for q= 7
- A022172 for q= 8
- A022173 for q= 9
- A022174 for q= 10
References
- Mukhin, Eugene. "Symmetric Polynomials and Partitions". http://mathcircle.berkeley.edu/BMC3/SymPol.pdf. (undated, 2004 or earlier).
- Ratnadha Kolhatkar, Zeta function of Grassmann Varieties (dated January 26, 2004)
- Weisstein, Eric W., "q-Binomial Coefficient" from MathWorld.
- Gould, Henry (1969). "The bracket function and Fontene-Ward generalized binomial coefficients with application to Fibonomial coefficients". Fib. Quart. 7: 23-40. MR0242691.
- Alexanderson, G. L. (1974). "A Fibonacci analogue of Gaussian binomial coefficients". Fib. Quart. 12: 129-132. MR0354537.
- Andrews, George E. (1974). "Applications of basic hypergeometric functions". SIAM Rev. 16 (4). doi:10.1137/1016081. JSTOR 2028690. MR0352557.
- Borwein, Peter B. (1988). "Pade approximants for the q-elementary functions". Construct. Approx. 4 (1): 391-402. doi:10.1007/BF02075469. MR0956175.
- Konvalina, John (1998). "Generalized binomial coefficients and the subset-subspace problem". Adv. Appl. Math. 21: 228-240. doi:10.1006/aama.1998.0598. MR1634713.
- Di Bucchianico, A. (1999). "Combinatorics, computer algebra and the Wilcoxon-Mann-Whitney test". J. Stat. Plann. Inf. 79: 349-364. doi:10.1016/S0378-3758(98)00261-4.
- Konvalina, John (2000). "A unified interpretation of the Binomial Coefficients, the Stirling numbers, and the Gaussian coefficients". Am. Math. Monthly 107 (10): 901-910. JSTOR 2695583. MR1806919.
- Kupershmidt, Boris A. (2000). "q-Newton binomial: from Euler to Gauss". J. Nonlin. Math. Phys. 7 (2): 244-262. Bibcode 2000JNMP....7..244K. MR1763640.
- Cohn, Henry (2004). "Projective geometry over F1 and the Gaussian Binomial Coefficients". Am. Math. Monthly 111 (6): 487-495. JSTOR 4145067. MR2076581.
- Kim, T. (2007). "q-Extension of the Euler formula and trigonometric functions". Russ. J. Math. Phys. 14 (3): -275-278. doi:10.1134/S1061920807030041. MR2341775.
- Kim, T. (2008). "q-Bernoulli numbers and polynomials associated with Gaussian binomial coefficients". Russ. J. Math. Physics 15 (1): 51-57. doi:10.1134/S1061920808010068. MR2390694.
- Corcino, Roberto B. (2008). "On p,q-binomial coefficients". Integers 8: #A29. MR2425627.
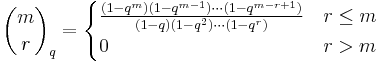
![[k]_q=\frac{1-q^k}{1-q}=\sum_{0\leq i<k}q^i=1%2Bq%2Bq^2%2B\cdots%2Bq^{k-1};](/2012-wikipedia_en_all_nopic_01_2012/I/7ffc6645362b2e1d6d1507198d4eb91f.png)
![{m \choose r}_q=\frac{[m]_q[m-1]_q\cdots[m-r%2B1]_q}{[1]_q[2]_q\cdots[r]_q}\quad(r\leq m),](/2012-wikipedia_en_all_nopic_01_2012/I/0415a83b5ca3dfc6540b3e59fca014c1.png)
![{m \choose r}_q=\frac{[m]_q!}{[r]_q!\,[m-r]_q!}\quad(r\leq m),](/2012-wikipedia_en_all_nopic_01_2012/I/5ebe1ab4d7a3120df0c239b67076294f.png)
![{\infty \choose r}_q = \lim_{m\rightarrow \infty} {m \choose r}_q = \frac{1}{[r]_q!\,(1-q)^r}](/2012-wikipedia_en_all_nopic_01_2012/I/50d773b0dce53b4b5b62c066f6ff40f2.png)
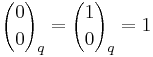
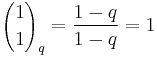
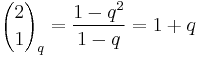
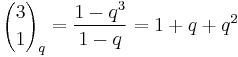
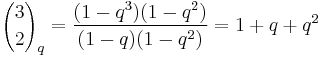
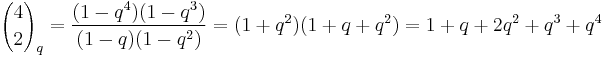
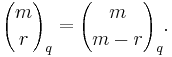
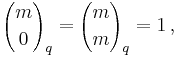
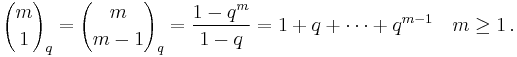
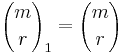
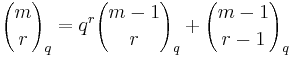
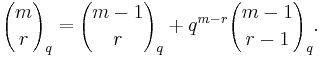
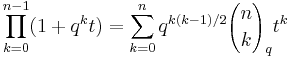

![\prod_{k=0}^{\infty} (1%2Bq^kt)=\sum_{k=0}^\infty \frac{q^{k(k-1)/2}t^k}{[k]_q!\,(1-q)^k}](/2012-wikipedia_en_all_nopic_01_2012/I/4d7e7734bc1f7bfd984104f84202c30c.png)
![\prod_{k=0}^\infty \frac{1}{(1-q^kt)}=\sum_{k=0}^\infty
\frac{t^k}{[k]_q!\,(1-q)^k} .](/2012-wikipedia_en_all_nopic_01_2012/I/189db612fdf7e24c420b57b8eeecc896.png)