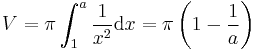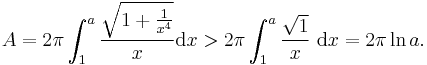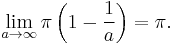Gabriel's Horn
Gabriel's Horn (also called Torricelli's trumpet) is a geometric figure which has infinite surface area but encloses a finite volume. The name refers to the tradition identifying the Archangel Gabriel as the angel who blows the horn to announce Judgment Day, associating the divine, or infinite, with the finite. The properties of this figure were first studied by Italian physicist and mathematician Evangelista Torricelli.
Contents |
Mathematical definition
Gabriel's horn is formed by taking the graph of 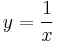 , with the domain
, with the domain 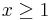 (thus avoiding the asymptote at x = 0) and rotating it in three dimensions about the x-axis. The discovery was made using Cavalieri's principle before the invention of calculus, but today calculus can be used to calculate the volume and surface area of the horn between x = 1 and x = a, where a > 1. Using integration (see Solid of revolution and Surface of revolution for details), it is possible to find the volume
(thus avoiding the asymptote at x = 0) and rotating it in three dimensions about the x-axis. The discovery was made using Cavalieri's principle before the invention of calculus, but today calculus can be used to calculate the volume and surface area of the horn between x = 1 and x = a, where a > 1. Using integration (see Solid of revolution and Surface of revolution for details), it is possible to find the volume  and the surface area
and the surface area  :
:
 can be as large as required, but it can be seen from the equation that the volume of the part of the horn between
can be as large as required, but it can be seen from the equation that the volume of the part of the horn between 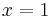 and
and  will never exceed
will never exceed  ; however, it will get closer and closer to
; however, it will get closer and closer to  as
as  becomes larger. Mathematically, the volume approaches
becomes larger. Mathematically, the volume approaches  as
as  approaches infinity. Using the limit notation of calculus, the volume may be expressed as:
approaches infinity. Using the limit notation of calculus, the volume may be expressed as:
This is so because as  approaches infinity,
approaches infinity, 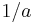 approaches zero. This means the volume approaches
approaches zero. This means the volume approaches  (1 - 0) which equals
(1 - 0) which equals  .
.
As for the area, the above shows that the area is greater than  times the natural logarithm of
times the natural logarithm of  . There is no upper bound for the natural logarithm of
. There is no upper bound for the natural logarithm of  as it approaches infinity. That means, in this case, that the horn has an infinite surface area. That is to say;
as it approaches infinity. That means, in this case, that the horn has an infinite surface area. That is to say;
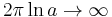 as
as 
or
Apparent paradox
When the properties of Gabriel's Horn were discovered, the fact that the rotation of an infinite curve about the x-axis generates an object of finite volume was considered paradoxical. However, the explanation is that the bounding curve, 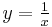 , is simply a special case–just like the simple harmonic series (Σx-1)–for which the successive area 'segments' do not decrease rapidly enough to allow for convergence to a limit. For volume segments (Σ1/x2) however, and in fact for any generally constructed higher degree curve (eg y = 1/x1.001), the same is not true and the rate of decrease in the associated series is sufficiently rapid for convergence to a (finite) limiting sum.
, is simply a special case–just like the simple harmonic series (Σx-1)–for which the successive area 'segments' do not decrease rapidly enough to allow for convergence to a limit. For volume segments (Σ1/x2) however, and in fact for any generally constructed higher degree curve (eg y = 1/x1.001), the same is not true and the rate of decrease in the associated series is sufficiently rapid for convergence to a (finite) limiting sum.
The apparent paradox formed part of a great dispute over the nature of infinity involving many of the key thinkers of the time including Thomas Hobbes, John Wallis and Galileo.[1]
The paradox can also be considered from a non-mathematical perspective. If Gabriel’s Horn existed in reality, it could be filled with a finite amount of paint since it has a finite volume. But then too, it has an infinite area; so an infinite amount of paint would therefore be required to cover its inner surface. It seems nonsensical to be able to completely fill a space with paint, yet fail to cover all that space's surface. The real-world solution to the paradox is that paint is not infinitely divisible; at some point, the throat of the Horn will become too small to allow even a single paint molecule to pass. When considering the Horn to this limited length, the practicably paintable surface of the inner surface is less than the amount of paint needed to fill it to that point.[2]
See also
References
External links
- Information and diagrams about Gabriel's Horn
- Torricelli's Trumpet at PlanetMath
- Weisstein, Eric W., "Gabriel's Horn" from MathWorld.
- "Gabriel's Horn" by John Snyder, the Wolfram Demonstrations Project, 2007.