Equinumerosity
"Equipollence" redirects here. For the concept in geometry, see Equipollence (geometry).
In mathematics, two sets are equinumerous if they have the same cardinality, i.e., if there exists a bijection f : A → B for sets A and B. This is usually denoted 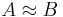 or
or 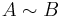 .
.
The study of cardinality is often called equinumerosity (equalness-of-number). The terms equipollence (equalness-of-strength) and equipotence (equalness-of-power) are sometimes used instead.
In Set, the category of all sets with functions as morphisms, an isomorphism between two sets is precisely a bijection, and two sets are equinumerous precisely if they are isomorphic in this category.