Demand (economics)

In economics, demand is the desire to own anything, the ability to pay for it, and the willingness to pay[1] (see also supply and demand). The term demand signifies the ability or the willingness to buy a particular commodity at a given point of time.

Economists record demand on a demand schedule and plot it on a graph as a demand curve that is usually downward sloping. The downward slope reflects the relationship between price and quantity demanded: as price decreases, quantity demanded increases. In principle, each consumer has a demand curve for any product that he or she would consider buying, and the consumer's demand curve is equal to the marginal utility (benefit) curve. When the demand curves of all consumers are added up, the result is the market demand curve for that product. If there are no externalities, the market demand curve is also equal to the social utility (benefit) curve.

Elements of the Law of Demand As Melvin and Boyes note the law of demand is defined as:

  1. The quantity of a well-defined good or service that:
  2. People are willing and able to buy.
  3. During a particular period of time.
  4. Decreases/increases as the price of that good or service rises/falls
  5. All other factors remain constant.

Melvin and Boyes (2010)

Demand is a relationship between two variables, price and quantity demanded, with all other factors that could affect demand being held constant.

well defined: The key phrase in the first element is “well defined”. The purpose of the phrase is to ensure that we are examining the relationship between price and quantity demanded for the same good. If we are interested in demand for a particular good there is no reason to compare the relationship between the price of the good and the change in quantity demanded of a different goods. Goods are well defined if they share the same characteristics—brand, model, age, quality and performance to name a few. For example a Cadillac CTS-V is a high performance car manufactured by General Motors. The defining feature of the car is its engine—a supercharged OHV 6.2 liter L V-8. The engine produces 556 horsepower and 551 lb·ft of torque. The engine enables the vehicle to go from zero to 60 in 3.9 seconds. The car cost about 65,000.00. If we are interested in the demand for the CTS-V we need to compare the price of a CTS-V to the quantity demanded for a CTS-V and not a Ford Festiva.
willing and able: to participate in the market a consumer must not only be willing to buy a good she must be able to buy as well. For example, John may want to buy a Cadillac CTS. However unless he has the cash or credit to consummate the purchase his unrealized desires are irrelevant.[2]
particular time period: demand measures the rate at which goods are being purchased during a specified period of time. For example to say that four thousand units are sold at a price of 65,000 does not tell us the level of demand unless we specify the time period per day per week per month.
nature of the relationship: this portion of the definition establishes that the price and quantity demanded have a negative or inverse relationship along the demand curve.[3]
held constant: there are innumerable factors other than price than can affect the level of demand. Some of the more important are income, price of related goods,[4] number of buyers, expectations and tastes and preferences.[5] To focus on the cause and effect relationship between the good's own price and the quantity of the good demanded all these other factors must be held constant. To hold a variable constant means to freeze its value and not allow it to change.

Contents

Factors affecting demand

Innumerable factors and circumstances could affect a buyer's willingness or ability to buy a good. Some of the more common factors are:

Good's own price: The basic demand relationship is between potential prices of a good and the quantities that would be purchased at those prices.[6] Generally the relationship is negative meaning that an increase in price will induce a decrease in the quantity demanded. This negative relationship is embodied in the downward slope of the consumer demand curve. The assumption of a negative relationship is reasonable and intuitive. If the price of a new novel is high, a person might decide to borrow the book from the public library rather than buy it.[7] Or if the price of a new piece of equipment is high a firm may decide to repair existing equipment rather than replacing it.
Price of related goods: The principal related goods are complements and substitutes. A complement is a good that is used with the primary good.[8] Examples include hotdogs and mustard, beer and pretzels, automobiles and gasoline. (Perfect complements behave as a single good.) If the price of the complement goes up the quantity demanded of the other good goes down.[9] Mathematically, the variable representing the price of the complementary good would have a negative coefficient in the demand function. For example, Qd = a - P - Pg where Q is the quantity of automobiles demanded, P is the price of automobiles and Pg is the price of gasoline. The other main category of related goods are substitutes. Substitutes are goods that can be used in place of the primary good. The mathematical relationship between the price of the substitute and the demand for the good in question is positive. If the price of the substitute goes down the demand for the good in question goes down.[10]
Personal Disposable Income: In most cases, the more disposable income (income after tax and receipt of benefits) you have the more likely you buy.[11][12]
Tastes or preferences: The greater the desire to own a good the more likely you are to buy the good.[13] There is a basic distinction between desire and demand. Desire is a measure of the willingness to buy a good based on its intrinsic qualities. Demand is the willingness and ability to put one's desires into effect. It is assumed that tastes and preferences are relatively constant.
Consumer expectations about future prices and income: If a consumer believes that the price of the good will be higher in the future he is more likely to purchase the good now. If the consumer expects that her income will be higher in the future the consumer may buy the good now. In other words positive expectations about future income may encourage present consumption.[14]

Demand function and demand equation

The demand equation is the mathematical expression of the relationship between the quantity of a good demanded and those factors that affect the willingness and ability of a consumer to buy the good. For example, Qd = f(P; Prg, Y) is a demand equation where Qd is the quantity of a good demanded, P is the price of the good, Prg is the price of a related good, and Y is income; the function on the right side of the equation is called the demand function. The semi-colon in the list of arguments in the demand function means that the variables to the right are being held constant as we plot the demand curve in (quantity, price) space. A simple example of a demand equation is Qd = 325 - P - 30Prg + 1.4Y. Here 325 is the repository of all relevant non-specified factors that affect demand for the product. P is the price of the good. The coefficient is negative in accordance with the law of demand. The related good may be either a complement or a substitute. If a complement, the coefficient of its price would be negative as in this example. If a substitute, the coefficient of its price would be positive. Income, Y, has a positive coefficient indicating that the good is a normal good. If the coefficient was negative the good in question would be an inferior good meaning that the demand for the good would fall as the consumer's income increased. Specifying values for the non price determinants, Prg = 4.00 and Y = 50, results in the demand equation Q = 325 - P - 30(4) +1.4(50) or Q = 275 - P. If income were to increase to 55 the new demand equation would be Q = 282 - P. Graphically this change in a non price determinant of demand would be reflected in an outward shift of the demand function caused by a change in the x intercept.

Demand curve

Main article: Demand curve

The relationship of price and quantity demanded can be exhibited graphically as the demand curve. The curve is generally negatively sloped. The curve is two-dimensional and depicts the relationship between two variables only: price and quantity demanded. All other factors affecting demand are held constant. However, these factors are part of the demand curve and influence the location of the curve. In many economics graphs, such as that of the demand curve, the independent variable is plotted on the vertical axis and the dependent variable on the horizontal axis. Consequently, the graphical presentation is technically that of the equation P = f(Q) where f(Q) is the inverse demand function, although the graph is referred to simply as the demand curve.

Income and Substitution Effects

The negative slope of the demand curve is due to the substitution and income effects. If the relative price of a good falls consumers will substitute that good for more expensive goods -that will buy more of the good whose relative price has fallen and less of the other goods.[15] This is the substitution effect. When the relative price of a good falls the consumer can buy the same bundle of goods as before the price decline and have some money left over.[16] This money can be used to purchase more of all his consumption goods. In other words his purchasing power is called the income effect.[17]

Discrete goods

In some cases it is impractical to represent the relationship between price and demand with a continuous curve because of small quantities demanded. Goods and services measured in small units are best represented with a smooth curve. Examples include food measured in calories and leisure measured in minutes. However, when the price of a good is very high in proportion to a consumer's budget there is a need to incorporate this limitation in both the mathematical analysis and the graph representing the relationship. While cars and houses are discrete goods for most people, cheaper goods such as glasses and bicycles are discrete goods only for the very poor. On the national level, nuclear power plants or space stations may be considered discrete goods. The concept is more useful at the individual consumer's level than at the consumers' aggregate level, because for example the difference between 3,000,000 cars demanded and 3,000,001 cars demanded is so little that the market demand for cars can be viewed as essentially continuous.

===The demand pinus

in the discrete case===

The price where the consumer is indifferent between buying an extra unit and not buying an extra unit is called the reservation price (r) after the same term used in auctions. If p is the price of the good and n units of the good are demanded, then rn>=p>=rn+1. For example, John is considering whether to buy a car or not (n=0 or n=1). The price of the car is $15,000 (p=15,000). The determining factor in John's consumption choice is his reservation price, r, simply the maximum price he is willing to pay for the car, reflecting his preferences. If John purchases this car and only this car then r1>=15,000>=r2 but if he does not purchase the car then r0>=15,000>=r1.

As with other demand curves, discrete demand curves are usually downward sloping, but in the case of discrete goods the curve is shaped like a staircase, reflecting the properties of goods which can only be consumed in quantities of integers. The horizontal line segments represent prices at which the consumer is indifferent between buying an extra unit or not. The vertical line segments represent ranges of prices where the quantity demanded does not vary. Nevertheless, as prices change within these ranges, the consumer surplus may change.

Movements versus shifts

The demand curve is a two-dimensional depiction of the relationship between price and quantity demanded. Movements along the curve occur only if there is a change in quantity demanded caused by a change in the good's own price. A shift in the demand curve, referred to as a change in demand, occurs only if a non-price determinant of demand changes. For example, if the price of a complement were to increase, the demand curve would shift leftward reflecting a decrease in demand. The shifted demand curve represents a new demand equation.

Movement along a demand curve due to a change in the good's price results in a change in the quantity demanded, not a change in demand. A change in demand refers to a shift in the position of the demand curve in two-dimensional space resulting from a change in one of the other arguments of the demand function.

From individual to market demand curve

The market demand curve is the horizontal summation of individual consumer demand curves.[18][19] Aggregation introduces three additional non-price determinants of demand: (1) the number of consumers; (2) "the distribution of tastes among the consumers"; and (3) "the distribution of incomes among consumers of different taste."[20] Thus if the population of consumers increases, ceteris paribus the market demand curve will shift outward (to the right).[21] If the proportion of consumers with a strong preference for a good increases, ceteris paribus the demand for the good will increase.[22] Finally if the distribution of income changes is favor of those consumers with a strong preference for the good in question the demand will shift out.[23] Factors that affect individual demand can also affect market demand. However, net effects must be considered. For example, a good that is a complement for one person is not necessarily a complement for another;[24] Further, the strength of the relationship would vary among persons. So in the aggregate the goods might be substitutes or complements. Finally the demand for a firm's product or services will often depend on such factors as competitors prices and marketing strategies.[25]

Horizontal versus vertical summation

When adding individual demand curves it is critical that the summation be horizontal rather than vertical. The derivation of the market demand function involves adding quantities.[26] The conventional graphical representation is of the inverse demand function. Adding inverse demand equations involves adding prices. In order to add the demand functions algebraically one must first convert the inverse equation to the standard demand function where quantity demanded is a function of price.[27] For example, assume that there are two consumers in a given market and their respective demand functions are P = 30 - 2Q and P = 30 - 6Q. To sum these functions to obtain the market demand curve we must first convert to standard form, that is Q = 15 - (P/2) and Q = 5 - (P/6). Then, adding Q1 and Q2 yields 15 - (P/2) + 5 - (P/6) = 20 - (4P/6)[28]= 20 - 2P/3.

Coefficient Addition

Note that in aggregating individual demand curves to determine market demand we added the coefficients for the goods own price. The unexpressed assumption that is the basis for the addition is the law of one price. With some variables addition of coefficients is more problematic. For example income is an important determinant of demand. However, the percentage of additional income that a person would spend for a particular good or service varies widely making simple coefficient addition less tenable. To borrow an example from Nicholson[29] assume that we have two consumers and that individuals 1’s demand for oranges is

X1 = 10 - 2Px + .1I1 + .5Py and individual 2’s demand for oranges is X2 = 17 - Px + 0.05I2 + .5Py

The market demand functions would be X1 + X2 = 27 - 3Px + .1I1 + 0.05I2 + Py.

Specifying values for I1, I2 and Px and substituting them into the demand equation we have:

*X1 + X2 = 39 - 3Px. Graphically this change in the price of a non price determinant of demand would be reflected in a shift out of the demand curve as the x intercept changed from 38 to 39.

'*X1 + X2 = 27 - 3Px + .1I1 + 0.05I2 + Py.

The redistribution of income has reduce demand and would be reflected in a shift inward of the demand curve.[30] Note that the assumption that each person's demand for a good is independent of everyone else's demand for the same good is a necessary assumption for aggregation.[31] Finally one must be aware that simple addition of coefficients can produce non-sensical results over certain range of prices. That is individual demand curves may not be valid at certain prices.[32]

As Frank notes, "Horizontal summation works as a way of generating market demand curves from individual demand curves because all consumers in the market face the same market price for the product. But when incomes differ widely from one consumer to another, it makes no sense to hold income constant and add quantities across consumers."[33]

Price elasticity of demand (PED)

PED is a measure of the sensitivity of the quantity variable, Q, to changes in the price variable, P. Elasticity answers the question of the percent by which the quantity demanded will change relative to (divided by) a given percentage change in the price. For infinitessimal changes the formula for calculating PED is the absolute value of (∂Q/∂P)×(P/Q).

Determinants of PED

The overriding factor in determining PED is the willingness and ability of consumers after a price changes to postpone immediate consumption decisions concerning the good and to search for substitutes (wait and look). The greater the incentive the consumer has to delay consumption and search for substitutes and the more readily available substitutes are the more elastic the demand will be. Specific factors are:

Availability of substitutes: The more choices that are available, the more elastic is the demand for a good. If the price of Pepsi goes up by 20%, one can always purchase Coke, 7-Up, Dr. Pepper and so forth. One's willingness and ability to postpone the consumption of Pepsi and get by with a "lesser brand" makes the PED of Pepsi relatively elastic.
Necessity: With a true necessity a consumer has neither the willingness nor the ability to postpone consumption. There are few or no satisfactory substitutes. Insulin is the ultimate necessity, so the demand for it is inelastic.
Importance in terms of proportion of income spent on a good: Most consumers have both the willingness and ability to postpone the purchase of big ticket items. If an item constitutes a significant portion of one's income, it is worth one's time to search for substitutes. A consumer will give more time and thought to the purchase of a $3000 television than a $1 candy bar, so demand for the former will be more elastic than demand for the latter.
Duration: The more time a consumer has to search for substitute goods, the more elastic the demand.[34]
Breadth of definition: how specifically the good is defined. For example, the demand for automobiles is less elastic than the demand for Toyotas, which is in turn less elastic than the demand for Red Toyota Priuses.
Availability of information concerning substitute goods: The easier it is for a consumer to locate the substitute goods, the more willing he will be to undertake the search, and the more elastic demand will be.

Elasticity along linear demand curve

The slope of a linear demand curve is constant. The elasticity of demand changes continuously as one moves down the demand curve because the ratio of price to quantity continuously falls. At the point the demand curve intersects the y-axis PED is infinitely elastic, because the variable Q apperaing in the demominator of the elasticity formula is zero there.[35] At the point the demand curve intersects the x-axis PED is zero, because the variable P appearing in the numerator of the elasticity formula is zero there.[36] At one point on the demand curve PED is unitary elastic: PED equals one. Above the point of unitary elasticity is the elastic range of the demand curve (meaning that the elasticity is greater than one). Below is the inelastic range, in which the elasticity is less than one. The decline in elasticity as one moves down the curve is due to the falling P/Q ratio.

Constant price elasticity demand

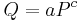Q=aP^{c} where a and c are parameters, and the constant price elasticity is c and c\le 0.

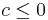Market structure and the demand curve

In perfectly competitive markets the demand curve, the average revenue curve, and the marginal revenue curve all coincide and are horizontal at the market-given price.[37] The demand curve is perfectly elastic and coincides with the average and marginal revenue curves. Economic actors are price-takers. Perfectly competitive firms have zero market power; that is, they have no ability to affect the terms and conditions of exchange. A perfectly competitive firm's decisions are limited to whether to produce and if so, how much. In less than perfectly competitive markets the demand curve is negatively sloped and there is a separate marginal revenue curve. A firm in a less than perfectly competitive market is a price-setter. The firm can decide how much to produce or what price to charge. In deciding one variable the firm is necessarily determining the other variable

Inverse demand function

In its standard form a linear demand equation is Q = a - bP. That is, quantity demanded is a function of price. The inverse demand equation, or price equation, treats price as a function g of quantity demanded: P = f(Q). To compute the inverse demand equation, simply solve for P from the demand equation.[38] For example, if the demand equation is Q = 240 - 2P then the inverse demand equation would be P = 120 - .5Q, the right side of which is the inverse demand function.[39]

The inverse demand function is useful in deriving the total and marginal revenue functions. Total revenue equals price, P, times quantity, Q, or TR = P×Q. Multiply the inverse demand function by Q to derive the total revenue function: TR = (120 - .5Q) × Q = 120Q - 0.5Q². The marginal revenue function is the first derivative of the total revenue function; here MR = 120 - Q. Note that the MR function has the same y-intercept as the inverse demand function in this linear example; the x-intercept of the MR function is one-half the value of that of the demand function, and the slope of the MR function is twice that of the inverse demand function. This relationship holds true for all linear demand equations. The importance of being able to quickly calculate MR is that the profit-maximizing condition for firms regardless of market structure is to produce where marginal revenue equals marginal cost (MC). To derive MC the first derivative of the total cost function is taken. For example assume cost, C, equals 420 + 60Q + Q2. Then MC = 60 + 2Q. Equating MR to MC and solving for Q gives Q = 20. So 20 is the profit maximizing quantity: to find the profit-maximizing price simply plug the value of Q into the inverse demand equation and solve for P.

Residual demand curve

The demand curve facing a particular firm is called the residual demand curve. The residual demand curve is the market demand that is not met by other firms in the industry at a given price. The residual demand curve is the market demand curve D(p), minus the supply of other organizations, So(p): Dr(p) = D(p) - So(p )[40]

Is the demand curve for PC firm really flat?

Practically every introductory microeconomics text describes the demand curve facing a perfectly competitive firm as being flat or horizontal. A horizontal demand curve is perfectly elastic. If there are n identical firms in the market then the elasticity of demand PED facing any one firm is

PEDmi = nPEDm - (n - 1) PES [41]

where PEDm is the market elasticity of demand, PES is the elasticity of supply of each of the other firms, and (n -1) is the number of other firms.[42] This formula suggests two things. The demand curve is not perfectly elastic and if there are a large number of firms in the industry the elasticity of demand for any individual firm will be extremely high and the demand curve facing the firm will be nearly flat.[43]

For example assume that there are 80 firms in the industry and that the demand elasticity for industry is -1.0 and the price elasticity of supply is 3. Then

PEDmi = nPEDm - (n - 1) PES,
PEDmi = (-1) - (80 - 1) 3,
PEDmi = -1(80) - (79 x 3)
PEDmi = -80 - 237 = - 317

That is the firm PED is 317 times as elastic as the market PED. If a firm raised its price "by one tenth of one percent demand would drop by nearly one third."[44] if the firm raised its price by three tenths of one percent the quantity demanded would drop by nearly 100%. Three tenths of one percent marks the effective range of pricing power the firm has because any attempt to raise prices by a higher percentage will effectively reduce quantity demanded to zero.

See also

Notes

  1. ^ Sullivan, Arthur; Steven M. Sheffrin (2003). Economics: Principles in action. Upper Saddle River, New Jersey 07458: Pearson Prentice Hall. pp. 79. ISBN 0-13-063085-3. http://www.pearsonschool.com/index.cfm?locator=PSZ3R9&PMDbSiteId=2781&PMDbSolutionId=6724&PMDbCategoryId=&PMDbProgramId=12881&level=4. 
  2. ^ Goodwin (2009) p. 85.
  3. ^ Goodwin (2009)
  4. ^ Related goods include complements and substitutes. A complement is a good used with another good. A substitute is a good used in place of another good.
  5. ^ Goodwin, (2009) p.89.
  6. ^ O’Sullivan, A. & Sheffrin, S, Microeconomics 4th ed. p. 62. Pearson 2005.
  7. ^ Goodwin, N, Nelson, J; Ackerman, F & Weissskopf, T: Microeconomics in Context 2d ed. Sharpe 2009
  8. ^ Colander, David C. Microeconomics 7th ed. p. 84. McGraw-Hill 2008.
  9. ^ Colander, David C. Microeconomics 7th ed. p. 84. McGraw-Hill 2008.
  10. ^ Colander, David C. Microeconomics 7th ed. p. 84. McGraw-Hill 2008.
  11. ^ Goodwin, Nelson, Ackerman, & Weissskopf, Microeconomics in Context 2d ed. (Sharpe 2009), p. 88.
  12. ^ Lovell (2004) p.71.
  13. ^ O’Sullivan, A. & Sheffrin, S, Microeconomics 4th ed. p. 74–75. Pearson 2005.
  14. ^ Goodwin, Nelson, Ackerman, & Weissskopf, Microeconomics in Context 2d ed. (Sharpe 2009) at 89.
  15. ^ Besanko and Braeutigam (2005) p. 146-47.
  16. ^ Besanko and Braeutigam (2005) p. 146-47.
  17. ^ Besanko and Braeutigam (2005) p. 146-47.
  18. ^ Nicholson & Snyder, Intermediate Microeconomics (Thomson 2007) p. 114–115.
  19. ^ A firm will not know the individual demand functions. So a firm could not derive its market demand curve by aggregating individual demand curves. There are empirical studies that estimate demand. As a rule of thumb the firm's demand curve is 5 to 6 times more elastic than the market demand curve. Thus a manager could take the market coefficient and multiply it by 6 to obtain the firm's PED. d
  20. ^ Binger & Hoffman, Microeconomics with Calculus, 2nd ed. (Addison-Wesley 1998) at 154-55
  21. ^ Binger & Hoffman, Microeconomics with Calculus, 2nd ed. (Addison-Wesley 1998) at 154-55
  22. ^ Binger & Hoffman, Microeconomics with Calculus, 2nd ed. (Addison-Wesley 1998) at 154-55
  23. ^ Binger & Hoffman, Microeconomics with Calculus, 2nd ed. (Addison-Wesley 1998) at 154-55.
  24. ^ Nicholson & Snyder, Intermediate Microeconomics (Thomson 2007) at 116.
  25. ^ Hirschey, Mark Managerial Economics Rev. Ed. (2000) 80-81.
  26. ^ Besanko and Braeutigam (2005) p 169.
  27. ^ Besanko p. 169.
  28. ^ Frank, R., Microeconomics and Behavior 7th ed. (Mc-Graw-Hill) ISBN 978-007-126349-8.
  29. ^ Nicholson (1998)186-89.
  30. ^ A more difficult problem with integration occurs when the system exhibits feedback. For example, consumer 1's taste and preferences may influence consumer 2's which in turn affect consumer 1's tastes and preferences.
  31. ^ Besanko and Braeutigam (2005) p. 169.
  32. ^ Besanko and Braeutigam (2005) p. 169.
  33. ^ Frank p. 121.
  34. ^ O’Sullivan, A. & Sheffrin, S, Microeconomics 4th ed. p. 99. Pearson 2005.
  35. ^ Colander, David C. Microeconomics 7th ed. pp. 132-33 McGraw-Hill 2008.
  36. ^ Colander, David C. Microeconomics 7th ed. pp. 132-33. McGraw-Hill 2008.
  37. ^ The perfectly competitive firm's demand curve is not in fact flat. However, if there are numerous firms in the industry the demand curve of an individual firm is likely to be extremely elastic, for a discussion of residual demand curves see Perloff (2008) at pp. 245–246.
  38. ^ The form of the inverse linear demand equation is P = a/b - 1/bQ.
  39. ^ Samuelson, W & Marks, S. Managerial Economics 4th ed. p. 37. Wiley 2003.
  40. ^ Perloff (2008) p. 243.
  41. ^ Perloff (2008) p. 243.
  42. ^ Perloff (2008) p. 245–246
  43. ^ Perloff (2008) p. 244.
  44. ^ Prloff (2008) p. 243.