Cumulative elevation gain
In running, cycling, and mountaineering, cumulative elevation gain refers to the sum of every gain in elevation throughout an entire trip. It is sometimes also known as cumulative gain or elevation gain, or often in the context of mountain travel, simply gain. Elevation losses are not counted in this measure. Cumulative elevation gain, along with round-trip distance, is arguably the most important value used in quantifying the strenuousness of a trip. This is because hiking 10 miles (16 km) on flat land (zero elevation gain) is significantly easier than hiking up a large mountain with a round-trip distance of 10 miles (16 km). It is much harder to ascend vertically, or to increase elevation, than to walk on flat land because doing so also requires that the hiker increase his/her gravitational potential energy.
Contents |
Computation (simple explanation)
In the simplest case of a trip where hikers only travel up on their way to a single summit, the cumulative elevation gain is simply given by the difference in the summit elevation and the starting elevation. For example, if one were to start hiking at a trailhead with elevation 1,000 feet (300 m), and hike up to a summit of 5,000 feet (1,500 m), the cumulative elevation gain would just be 5000 ft - 1000 ft = 4000 ft. The loss of elevation on the descent is not relevant, because only increases in elevation are considered in this measure.
However, when climbing a mountain with some "ups-and-downs", or traversing several mountains, you must take into account every "up" along the whole route. This even means that the (usually small) uphills on the descent must be counted. For example, consider a mountain whose summit was 5,000 feet (1,500 m) in elevation, but somewhere on the way up, the trail went back down 250 feet (76 m). If starting at an elevation of 1,000 feet (300 m), one would gain 4,250 feet (1,300 m) on the way up (not 4000, because 250 is lost and has to be "regained") and 250 more feet on the way down, leading to a cumulative elevation gain of 4,500 feet (1,400 m) on the trip.
If one were to hike over five hills of 100 vertical feet each, and back, the cumulative elevation gain would be 5 x (100 ft) x 2 = 1000 ft.
This concept makes travel on mountains which have more "ups-and-downs", or are generally more rugged, significantly more strenuous.
Computation (mathematical explanation)
More generally, suppose a hiker's elevation 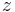 at a linear distance from the starting point
at a linear distance from the starting point 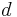 can be expressed as an elevation profile function
can be expressed as an elevation profile function  . For instance, the hiker's elevation 4 miles (6.4 km) into the hike would be given by
. For instance, the hiker's elevation 4 miles (6.4 km) into the hike would be given by  .
.
The derivative of the elevation profile  is related to the steepness at a distance
is related to the steepness at a distance 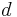 into the trip. We can then define a gain function as the elevation gained cumulatively up to the point
into the trip. We can then define a gain function as the elevation gained cumulatively up to the point 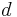 in a trip. The gain function can be expressed as
in a trip. The gain function can be expressed as
 ,
,
where  is the Heaviside step function and the prime mark is Lagrange's notation for a derivative. The purpose of the step function is to zero-out all downhills. That is, any downhill along the way would result in a negative value of
is the Heaviside step function and the prime mark is Lagrange's notation for a derivative. The purpose of the step function is to zero-out all downhills. That is, any downhill along the way would result in a negative value of  , which would make the step function, and consequently the integrand, zero (so that downhills do not get added). A cumulative elevation loss function could easily be constructed by the insertion of a negative sign inside the step function, so that only downhills are factored in.
, which would make the step function, and consequently the integrand, zero (so that downhills do not get added). A cumulative elevation loss function could easily be constructed by the insertion of a negative sign inside the step function, so that only downhills are factored in.
If the hiker traveled a total distance of  , the cumulative gain on the trip would be given by
, the cumulative gain on the trip would be given by
 .
.