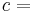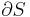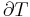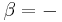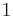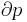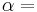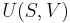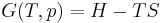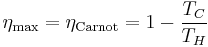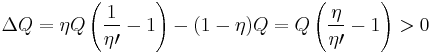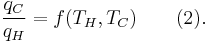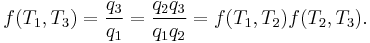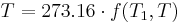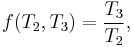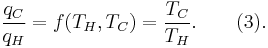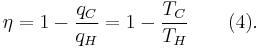Carnot's theorem (thermodynamics)

Thermodynamics
Branches Classical · Statistical · Chemical Equilibrium / Non-equilibrium Thermofluids
Laws Zeroth · First · Second · Third
Systems State: Equation of state Ideal gas · Real gas Phase of matter · Equilibrium Control volume · Instruments Processes: Isobaric · Isochoric · Isothermal Adiabatic · Isentropic · Isenthalpic Quasistatic · Polytropic Free expansion Reversibility · Irreversibility Endoreversibility Cycles: Heat engines · Heat pumps Thermal efficiency
System properties Property diagrams Intensive and extensive properties State functions: Temperature / Entropy (intro.) † Pressure / Volume † Chemical potential / Particle no. † († Conjugate variables) Vapor quality Reduced properties Process functions: Work · Heat
Material properties Specific heat capacity  Compressibility  Thermal expansion  Property database
Equations Carnot's theorem Clausius theorem Fundamental relation Ideal gas law Maxwell relations Table of thermodynamic equations
Potentials Free energy · Free entropy Internal energy Enthalpy Helmholtz free energy Gibbs free energy
History and culture Philosophy: Entropy and time · Entropy and life Brownian ratchet Maxwell's demon Heat death paradox Loschmidt's paradox Synergetics History: General · Heat · Entropy · Gas laws Perpetual motion Theories: Caloric theory · Vis viva Theory of heat Mechanical equivalent of heat Motive power Publications: "An Experimental Enquiry Concerning ... Heat" "On the Equilibrium of Heterogeneous Substances" "Reflections on the Motive Power of Fire" Timelines of: Thermodynamics · Heat engines Art: Maxwell's thermodynamic surface Education: Entropy as energy dispersal
Scientists Daniel Bernoulli Sadi Carnot Benoît Paul Émile Clapeyron Rudolf Clausius Hermann von Helmholtz Constantin Carathéodory Pierre Duhem Josiah Willard Gibbs James Prescott Joule James Clerk Maxwell Julius Robert von Mayer William Rankine John Smeaton Georg Ernst Stahl Benjamin Thompson William Thomson, 1st Baron Kelvin John James Waterston

Carnot's theorem, developed in 1824, also called Carnot's rule is a principle that specifies limits on the maximum efficiency any heat engine can obtain, which thus solely depends on the difference between the hot and cold temperature reservoirs.

Carnot's theorem states:

• All irreversible heat engines between two heat reservoirs are less efficient than a Carnot engine operating between the same reservoirs.
• All reversible heat engines between two heat reservoirs are equally efficient with a Carnot engine operating between the same reservoirs.

The formula for this maximum efficiency is

where T_C is the absolute temperature of the cold reservoir, T_H is the absolute temperature of the hot reservoir, and the efficiency 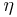is the ratio of the work done by the engine to the heat drawn out of the hot reservoir.

Based on modern thermodynamics, Carnot's theorem is a result of the second law of thermodynamics. Historically, however, it was based on contemporary caloric theory and preceded the establishment of the second law.^[1]

Contents 1 Proof 1.1 Irreversible engine 1.2 Reversible engine 2 Definition of thermodynamic temperature 3 Applicability to fuel cells and batteries 4 References

Proof

The theorem may be proved in the following way for the cases of the irreversible and the reversible heat engines.^[2]

Irreversible engine

The derivation assumes an irreversible heat engine that has an efficiency 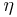, operating between reservoirs 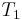and 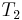. It is combined with a reversed Carnot engine that has efficiency 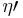as shown by the diagram to the right.

If , then the combined engine does nothing in a cycle, which contradicts the irreversibility.

If 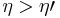, then the net effect of the combined engine is draining heat

from the colder reservoir 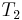and releases the same amount to the hotter reservoir 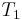without affecting any other changes. This is clearly in violation of the Clausius statement of the second law.

The conclusion is that the only possibility remaining is .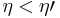

Reversible engine

In the case of a reversible heat engine, the proof may be conducted similarly, in that .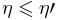

The said engine is reversed and combined with a regular Carnot engine. In a similar manner the conclusion is obtained: 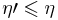. Thus,

.

Definition of thermodynamic temperature

The efficiency of the engine is the work divided by the heat introduced to the system or

,

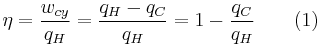where w_cy is the work done per cycle. Thus, the efficiency depends only on q_C/q_H.

Because all reversible engines operating between the same heat reservoirs are equally efficient, any reversible heat engine operating between temperatures T₁ and T₂ must have the same efficiency, meaning, the efficiency is the function of the temperatures only:

In addition, a reversible heat engine operating between temperatures T₁ and T₃ must have the same efficiency as one consisting of two cycles, one between T₁ and another (intermediate) temperature T₂, and the second between T₂andT₃. This can only be the case if

Specializing to the case that 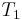is a fixed reference temperature: the temperature of the triple point of water. Then for anyT₂and T₃,

Therefore, if thermodynamic temperature is defined by

then the function f, viewed as a function of thermodynamic temperature, is

and the reference temperature T₁ has the value 273.16. (Of course any reference temperature and any positive numerical value could be used—the choice here corresponds to the Kelvin scale.)

It follows immediately that

Substituting Equation 3 back into Equation 1 gives a relationship for the efficiency in terms of temperature:

Applicability to fuel cells and batteries

Since fuel cells and batteries can generate useful power when all components of the system are at the same temperature (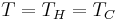), they are clearly not limited by Carnot's theorem, which states that no power can be generated when 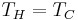. This is because Carnot's theorem applies to engines converting thermal energy to work, whereas fuel cells and batteries instead convert chemical energy to work.^[3] Nevertheless, the second law of thermodynamics still provides restrictions on fuel cell and battery energy conversion.^[4]

References