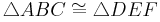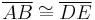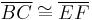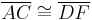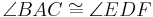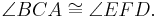CPCTC

In geometry, CPCTC is the abbreviation of a theorem involving congruent triangles. CPCTC stands for Corresponding Parts of Congruent Triangles are Congruent. CPCTC states that if two or more triangles are proven congruent by: ASA, AAS, SSS, HL, or SAS, then all of their corresponding parts are congruent as well. CPCTC is especially useful in proving various geometrical problems and theorems.

If

\triangle ABC \cong \triangle DEF\,

then the following statements are true:

\overline{AB} \cong \overline{DE}\,
\overline{BC} \cong \overline{EF}\,
\overline{AC} \cong \overline{DF}
\angle BAC \cong \angle EDF\,
\angle ABC \cong \angle DEF\,
\angle BCA \cong \angle EFD.\,

A related theorem is CPCtC, in which triangles is replaced with figures so that the theorem applies to any polygon or polyhedron.

External links