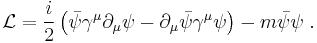Bispinor

In physics, bispinor is a four-component object which transforms under the (½,0)⊕(0,½) representation of the covariance group of special relativity[1] (see, e.g., [2]). Bispinors are used to describe relativistic spin-½ quantum fields.

In the Weyl basis a bispinor

\psi=\left(\begin{array}{c}\phi\\ \chi\end{array}\right)

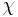consists of two (two-component) Weyl spinors \phi and \chi which transform, correspondingly, under (½,0) and (0,½) representations of the SO(1,3) group (the Lorentz group without parity transformations). Under parity transformation the Weyl spinors transform into each other.

The Dirac bispinor is connected with the Weyl bispinor by a unitary transformation to the Dirac basis,

\psi\rightarrow{1\over\sqrt2}\left[ \begin{array}{cc}1&1\\1&-1 \end{array} \right]\psi= {1\over\sqrt2}\left(\begin{array}{c}\phi%2B\chi\\ \phi-\chi \end{array}\right) .

The Dirac basis is the one most widely used in the literature.

A bilinear form of bispinors 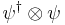\psi^\dagger\otimes\psi can be reduced to five irreducible (under the Lorentz group) objects:

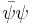  1. scalar, \bar{\psi}\psi ;
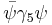  2. pseudo-scalar, \bar{\psi}\gamma_5\psi ;
  3. vector, \bar{\psi}\gamma^\mu\psi ;
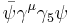  4. pseudo-vector, \bar{\psi}\gamma^\mu\gamma_5\psi ;
  5. antisymmetric tensor, \bar{\psi}(\gamma^\mu\gamma^\nu-\gamma^\nu\gamma^\mu)\psi ,

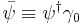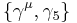where \bar{\psi}\equiv\psi^\dagger\gamma_0 and \{\gamma^\mu,\gamma_5\} are the gamma matrices.

A suitable Lagrangian (the Euler-Lagrange equation of which is the Dirac equation) for the relativistic spin-½ field is given as

\mathcal{L}={i\over2}\left( \bar{\psi}\gamma^\mu\partial_\mu\psi-\partial_\mu\bar{\psi}\gamma^\mu\psi\right)-m\bar{\psi}\psi\;.

Notes

  1. ^ covariance group of special relativity is 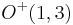O^%2B(1,3) or the Lorentz group.
  2. ^ Caban and Rembielinski 2005, p. 2.

References