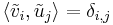Biorthogonal system

In mathematics, a biorthogonal system is a pair of topological vector spaces E and F that are in duality, with a pair of indexed subsets

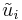$\tilde v_i$ in E and $\tilde u_i$ in F

such that

$\langle\tilde v_i , \tilde u_j\rangle = \delta_{i,j}$

with the Kronecker delta. This applies, for example, with E = F = H a Hilbert space; in which case a biorthogonal system reduces to an orthonormal system. In L²[0,2π] the functions cos nx and sin nx form a biorthogonal system.

1 Projection
2 Construction
3 See also
4 References

Projection

Related to a biorthogonal system is the projection

$P:= \sum_{i \in I} \tilde u_i \otimes \tilde v_i$ ,

where $\left( u \otimes v\right) (x):= u \langle v, x\rangle$ ; its image is the linear span of $\{\tilde u_i: i \in I\}$ , and the kernel is $\{\langle\tilde v_i, \cdot\rangle = 0: i \in I \}$ .

Construction

Given a possibly non-orthogonal set of vectors $\mathbf{u}= (u_i)$ and $\mathbf{v}= (v_i)$ the projection related is

$P= \sum_{i,j} u_i \left( \langle\mathbf{v}, \mathbf{u}\rangle^{-1}\right)_{j,i} \otimes v_j$ ,

where $\langle\mathbf{v},\mathbf{u}\rangle$ is the matrix with entries $\left(\langle\mathbf{v},\mathbf{u}\rangle\right)_{i,j}= \langle v_i, u_j\rangle$ .

$\tilde u_i:= (I-P) u_i$ , and $\tilde v_i:= \left(I-P \right)^* v_i$ then is an orthogonal system.

References

Jean Dieudonné, On biorthogonal systems Michigan Math. J. 2 (1953), no. 1, 7–20 [1]

Biorthogonal system

Contents

Projection

Construction

See also

References