Amagat

An amagat is a practical unit of number density. Although it can be applied to any substance at any conditions, it is defined as the number of ideal gas molecules per unit volume at 1 atm (= 101.325 kPa) and 0 °C (= 273.15 K).^[1] It is named after Emile Amagat, who also has Amagat's law named after him. The abbreviated form of amagat is "amg."

Definition

Number density in amg, denoted here by 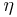$\eta$ , is defined as

$\eta= \frac{n}{n_0}$ ,

where n₀ = 1 amg = 2.686 7774×10²⁵ m⁻³ = 44.614 981 mol/m³ is the Loschmidt constant.

In practice, number density in amagat of an ideal gas at pressure P and temperature T can be calculated as^[2]

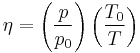$\eta= \left(\frac{p}{p_0}\right)\left(\frac{T_0}{T}\right)$ ,

where T₀ = 273.15 K and p₀ = 101.325 kPa.

Example

Number density of an ideal gas (such as air) at room temperature (20 °C) and 1 atm (101.325 kPa) is

$\eta= \left(\frac{1\, atm}{p_0}\right)\left(\frac{273.15 K}{(273.15%2B20) K}\right)=0.932 {\rm amg}$ .

References

^ Hirschfelder, Joseph O.; Curtiss, Charles F.; Bird, R. Byron (1967), Molecular Theory of Gases and Liquids (Corrected printing ed.), John Wiley & Sons, Inc.
^ In this formula, absolute units of pressure and temperature, relative to vacuum and absolute zero, must be used.