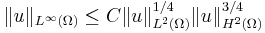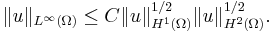Agmon's inequality
In mathematical analysis, Agmon's inequalities, named after Shmuel Agmon,[1] consist of a few closely related inequalities between the Lebesgue space 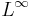 and the Sobolev spaces
and the Sobolev spaces  . It is useful in the study of partial differential equations.
. It is useful in the study of partial differential equations.
The result is stated in 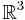 only. Let
only. Let  be a vector-valued function,
be a vector-valued function, 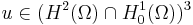 where
where 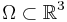 . Then Agmon's inequalities state that there exists a constant
. Then Agmon's inequalities state that there exists a constant 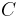 such that
such that
and
References
- Agmon, Shmuel (2010). Lectures on elliptic boundary value problems. , Providence, RI: AMS Chelsea Publishing. ISBN 978-0-8218-4910-1.
- Foias, Ciprian; Manley, O.; Rosa, R.; Temam, R. (2001). Navier-Stokes Equations and Turbulence. Cambridge: Cambridge University Press. ISBN 0-521-36032-3.
Notes
- ^ Lemma 13.2, in: Agmon, Shmuel, Lectures on Elliptic Boundary Value Problems, AMS Chelsea Publishing, Providence, RI, 2010. ISBN 978-0-8218-4910-1.