4 (number)

4
−1 0 1 2 3 4 5 6 7 8 9 → List of numbers — Integers 0 10 20 30 40 50 60 70 80 90 →
Cardinal	4 four
Ordinal	4th fourth
Numeral system	quaternary
Factorization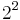
Divisors	1, 2, 4
Roman numeral	IV or IIII
Roman numeral (Unicode)	Ⅳ, ⅳ
Greek	δ (or Δ)
Arabic	٤,4
Arabic (Persian, Urdu)	۴
Ge'ez	፬
Bengali	৪
Chinese numeral	四，亖，肆
Devanagari	४
Telugu	౪
Malayalam	൪
Tamil	௪
Hebrew	ארבע (Arba, pronounced AR-bah) or ד (Dalet, 4th letter of the Hebrew alphabet)
Khmer	៤
Thai	๔
prefixes	tetra- (from Greek) quadri-/quadr- (from Latin)
Binary	100
Octal	4
Duodecimal	4
Hexadecimal	4
Vigesimal	4

4 (four; /ˈfɔər/) is a number, numeral, and glyph. It is the natural number following 3 and preceding 5.

Contents 1 In mathematics 1.1 List of basic calculations 2 Evolution of the glyph 3 In religion 4 In science 4.1 In astronomy 4.2 In biology 4.3 In chemistry 4.4 In physics 5 In logic and philosophy 6 In technology 7 In transport 8 In sports 9 In other fields 10 In music 11 Groups of four 12 References 13 External links

In mathematics

Four is the smallest composite number, its proper divisors being 1 and 2. Four is also a highly composite number. The next highly composite number is 6.

Four is the second square number, the second centered triangular number.

4 is the smallest squared prime (p²) and the only even number in this form. It has an aliquot sum of 3 which is itself prime. The aliquot sequence of 4 has 4 members (4, 3, 1, 0) and is accordingly the first member of the 3-aliquot tree.

Only one number has an aliquot sum of 4 and that is squared prime 9.

The prime factorization of four is two times two.

Four is the smallest composite number that is equal to the sum of its prime factors. (As a consequence of this, it is the smallest Smith number). However, it is the largest (and only) composite number n for which 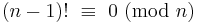is false.

It is also a Motzkin number.

In bases 6 and 12, 4 is a 1-automorphic number.

In addition, 2 + 2 = 2 × 2 = 2² = 4. Continuing the pattern in Knuth's up-arrow notation, 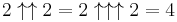, and so on, for any number of up arrows.

A four-sided plane figure is a quadrilateral (quadrangle) or square, sometimes also called a tetragon. A circle divided by 4 makes right angles. Because of it, four (4) is the base number of plane (mathematics). Four cardinal directions, four seasons, duodecimal system, and vigesimal system are based on four.

A solid figure with four faces is a tetrahedron. The regular tetrahedron is the simplest Platonic solid. A tetrahedron, which can also be called a 3-simplex, has four triangular faces and four vertices. It is the only self-dual regular polyhedron.

Four-dimensional space is the highest-dimensional space featuring more than three convex regular figures:

• Two-dimensional: infinitely many convex regular polygons.
• Three-dimensional: five convex regular polyhedra (the five Platonic Solids).
• Four-dimensional: six convex regular polychora.
• Five-dimensional and every higher-dimensional: three regular convex polytopes (regular simplexes, hypercubes, cross-polytopes).

Four-dimensional differential manifolds have some unique properties. There is only one differential structure on 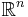except when n = 4, in which case there are uncountably many.

The smallest non-cyclic group has four elements; it is the Klein four-group. Four is also the order of the smallest non-trivial groups that are not simple.

Four is the maximum number of dimensions of a real division algebra (the quaternions), by a theorem of Ferdinand Georg Frobenius.

The four-color theorem states that a planar graph (or, equivalently, a flat map of two-dimensional regions such as countries) can be colored using four colors, so that adjacent vertices (or regions) are always different colors.^[1] Three colors are not, in general, sufficient to guarantee this. The largest planar complete graph has four vertices.

Lagrange's four-square theorem states that every positive integer can be written as the sum of at most four square numbers. Three are not always sufficient; 7 for instance cannot be written as the sum of three squares.

Four is the first positive non-Fibonacci number.

Each natural number divisible by 4 is a difference of squares of two natural numbers, i.e. 4x = y² − z².

Four is an all-Harshad number and a semi-meandric number.

List of basic calculations

Multiplication	1	2	3	4	5	6	7	8	9	10		11	12	13	14	15	16	17	18	19	20		21	22	23	24	25		50	100	1000
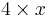	4	8	12	16	20	24	28	32	36	40		44	48	52	56	60	64	68	72	76	80		84	88	92	96	100		200	400	4000

Division	1	2	3	4	5	6	7	8	9	10		11	12	13	14	15	16
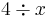	4	2		1	0.8		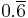	0.5		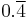0.4				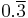			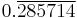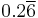0.25
	0.25	0.5	0.75	1	1.25	1.5	1.75	2	2.25	2.5		2.75	3	3.25	3.5	3.75	4

Exponentiation	1	2	3	4	5	6	7	8	9	10		11	12	13
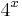	4	16	64	256	1024	4096	16384	65536	262144	1048576		4194304	16777216	67108864
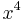	1	16	81	256	625	1296	2401	4096	6561	10000		14641	20736	28561

Evolution of the glyph

Representing 1, 2 and 3 in as many lines as the number represented worked well. The Brahmin Indians simplified 4 by joining its four lines into a cross that looks like our modern plus sign. The Sunga would add a horizontal line on top of the numeral, and the Kshatrapa and Pallava evolved the numeral to a point where speed of writing was a secondary concern. The Arabs' 4 still had the early concept of the cross, but for the sake of efficiency, was made in one stroke by connecting the "western" end to the "northern" end; the "eastern" end was finished off with a curve. The Europeans dropped the finishing curve and gradually made the numeral less cursive, ending up with a glyph very close to the original Brahmin cross.^[2]

While the shape of the 4 character has an ascender in most modern typefaces, in typefaces with text figures the character usually has a descender, as, for example, in .

On the seven-segment displays of pocket calculators and digital watches, as well as certain optical character recognition fonts, 4 is seen with an open top. This is because this was the original way the glyph was drawn (before the illustration above came into usage).

Television stations that operate on channel 4 have occasionally made use of another variation of the "open 4", with the open portion being on the side, rather than the top. This version resembles the Canadian Aboriginal syllabics letter ᔦ. The magnetic ink character recognition "CMC-7" font also uses this variety of "4". Another form of the 4 glyph that was invented for television was the arrow 4, which combines the 4 glyph with an arrow.

In religion

Buddhism

• Four Noble Truths
  • Dukkha – The Noble Truth of Suffering
  • Samudaya – The Noble Truth of the Cause of Suffering
  • Nirodha – The Noble Truth of the Cessation of Suffering
  • Magga – The Noble Truth of the Path leading to the Cessation of Suffering
• Four sights – observations which affected Prince Siddhartha deeply and made him realize the sufferings of all beings, and compelled him to begin his spiritual journey—an old man, a sick man, a dead man, and an ascetic
• Four Great Elements – earth, water, fire, and wind
• Four Heavenly Kings
• Four Foundations of Mindfulness – contemplation of the body, contemplation of feelings, contemplation of mind, contemplation of mental objects
• Four Right Exertions
• Four Bases of Power
• Four jhānas
• Four arūpajhānas
• Four Divine Abidings – loving-kindness, compassion, sympathetic joy, and equanimity
• Four stages of enlightenment – stream-enterer, once-returner, non-returner, and arahant
• Four main pilgrimage sites – Lumbini, Bodh Gaya, Sarnath, and Kusinara

Judeo-Christian symbolism

• The Tetragrammaton is the four-letter name of God.
• The four Matriarchs (foremothers) of Judaism are Sarah, Rebeccah, Leah, and Rachel.
• The Four Species (lulav, hadass, aravah and etrog) are taken as one of the mitzvot on the Jewish holiday of Sukkot. (Judaism)
• The Four Cups of Wine to drink on the Jewish holiday of Passover. (Judaism)
• The Four Questions to be asked on the Jewish holiday of Passover. (Judaism)
• The Four Sons to be dealt with on the Jewish holiday of Passover. (Judaism)
• The Four Expressions of Redemption to be said on the Jewish holiday of Passover. (Judaism)
• The Four Horsemen of the Apocalypse ride in the Book of Revelation.
• The four Gospels: Matthew, Mark, Luke, and John.

Hinduism

• In Puruṣārtha there are 4 aims of human life Dharma, Artha, Kāma, Moksha
• The four stages of life Brahmacharya (student life), Grihastha (household life), Vanaprastha (retired life) and Sannyasa (renunciation).
• The four primary castes or strata of society Brahmana (priest/teacher), Kshatriya (warrior/politician), Vaishya (landowner/entrepreneur) and Shudra (servant/manual laborer)

Islam

• Eid al-Adha lasts for four days, from the 10th to the 14th of Dhul Hijja.
• There are four The Rightly Guided Caliphs or Rashidun: Abu Bakr, Umar ibn al-Khattab, Uthman ibn Affan and Ali ibn Abi Talib.
• The Four Arch Angels in Islam are: Jibraeel (Gabriel), Mikaeel (Michael), Izraeel (Azrael), and Israfil (Raphael)
• There are four Sacred Months in Islam: Muharram, Rajab, Dhu al-Qi'dah and Dhu al-Hijjah.
• There are four Sunni schools of fiqh: Hanafi, Shafi`i, Maliki and Hanbali.
• There are four major sunni Imams: Abū Ḥanīfa, Muhammad ibn Idris ash-Shafi`i, Malik ibn Anas and Ahmad ibn Hanbal.
• There are four books in Islam: Torah, Zaboor, Injeel, Quran.
• Waiting for four months is ordained for those who take an oath for abstention from their wives.
• The waiting period of the woman whose husband dies, is four months and ten days.
• When Abraham said: “My Lord, show me how You give life to the dead,” Allah said: “Why! Do you have no faith?” Abraham replied: “Yes, but in order that my heart be at rest.” He said: “Then take four birds, and tame them to yourself, then put a part of them on every hill, and summon them; they will come to you flying.
• The respite of four months was granted to give time to the mushriks in Surah Taubah so that they should consider their position carefully and decide whether to make preparation for war or to emigrate from the country or to accept Islam.
• Those who accuse honourable women (of unchastity) but do not produce four witnesses, flog them with eighty lashes, and do not admit their testimony ever after. They are indeed transgressors. Surah Noor

Other

• Four is the sacred number of the Zia, an indigenous tribe located in the U.S. State of New Mexico.
• The Chinese, Vietnamese, the Korean and the Japanese are superstitious about the number four because it is a homonym for "Death" in their languages

In science

• A tetramer is a thing formed out of four sub-units.

In astronomy

• Four terrestrial (or rocky) planets in the Solar system: Mercury, Venus, Earth, Mars.
• Four giant gas planets in the Solar system: Jupiter, Saturn, Uranus, Neptune.
• Four of Jupiter's moons (the Galilean moons) are readily visible from Earth.
• Messier object M4, a magnitude 7.5 globular cluster in the constellation Scorpius.
• The New General Catalogue object NGC 4, a faint galaxy in the constellation Pisces
• The Roman numeral IV stands for subgiant in the Yerkes spectral classification scheme.
• The Roman numeral IV (usually) stands for the fourth-discovered satellite of a planet or minor planet (e.g. Jupiter IV)

In biology

• Four is the number of nucleobase types in DNA and RNA – adenine, guanine, cytosine, thymine (uracil in RNA).
• Many chordates have four feet, legs or leglike appendages (Tetrapods).
• The mammalian heart consists of four chambers.
• Many mammals (Carnivora, Ungulata) use four fingers for movement.
• The fourth finger of a human hand (on the left hand – ring-finger) is moved when the little finger moves.
• All insects with wings except flies have four wings.
• Insects of the superorder Endopterygota, also known as Holometabola, such as butterflies, ants, bees, beetles, fleas, flies, moths, and wasps, undergo holometabolism – complete metamorphism in four stages – from (1) embryo (ovum, egg), to (2) larva (such as grub, caterpillar), then (3) pupa (such as the chrysalis), and finally (4) the imago.
• There are four human blood groups (A, B, O, AB).
• Humans have four canines, four incisors and four wisdom teeth.
• The cow's stomach is divided in four digestive compartments: reticulum, rumen, omasum and abomasum.

In chemistry

• Valency of carbon (that is basis of life on the Earth) is four. Also because of its tetrahedral crystal bond structure, diamond (one of the natural allotropes of carbon) is the hardest known naturally occurring material. It is also the valence of silicon, whose compounds form the majority of the mass of the Earth's crust.
• The atomic number of beryllium
• There are four basic states of matter: solid, liquid, gas, and plasma.
• The four elements of alchemy are earth, water, air and fire.

In physics

• Special relativity and general relativity treat nature as four-dimensional – time and three-dimensional space are treated together and called spacetime. Also, any event E has a light cone composed of four zones of possible communication and cause and effect (outside the light cone is strictly incommunicado).
• An alpha particle (helium nucleus, also called a helion) consists of four hadrons.
• There are four fundamental forces (electromagnetism, gravitation, the weak nuclear force, and the strong nuclear force).
• In statistical mechanics, the four functions inequality is an inequality for four functions on a finite distributive lattice.

In logic and philosophy

• The symbolic meanings of the number four are linked to those of the cross and the square. "Almost from prehistoric times, the number four was employed to signify what was solid, what could be touched and felt. Its relationship to the cross (four points) made it an outstanding symbol of wholeness and universality, a symbol which drew all to itself". Where lines of latitude and longitude intersect, they divide the earth into four proportions. Throughout the world kings and chieftains have been called "lord of the four suns"..."lord of the four quarters of the earth"...^[3] by which is understood to the extent of their powers both territorially and in terms of total control of their subjects' doings.
• The Square of Opposition, in both its Aristotelian version and its Boolean version, consists of four forms: A ("All S is R"), I ("Some S is R"), E ("No S is R"), and O ("Some S is not R").
• In regard to whether two given propositions can have the same truth value, there are four separate logical possibilities: the propositions are subalterns (possibly both are true, and possibly both are false); subcontraries (it is possible that both are true, but not that both are false); contraries (it is possible that both are false, but not that both are true); or contradictories (it is not possible that both are true, and it is not possible that both are false).
• Aristotle held that there are basically four causes in nature: the efficient cause, the matter, the end, and the form.
• The Stoics held with four basic categories, all viewed as bodies (substantial and insubstantial): (1) substance in the sense of substrate, primary formless matter; (2) quality, matter's organization to differentiate and individualize something, and coming down to a physical ingredient such as pneuma, breath; (3) somehow holding (or disposed), as in a posture, state, shape, size, action, and (4) somehow holding (or disposed) toward something, as in relative location, familial relation, and so forth.
• Immanuel Kant expounded a table of judgments involving four three-way alternatives, in regard to (1) Quantity, (2) Quality, (3) Relation, (4) Modality, and, based thereupon, a table of four categories, named by the terms just listed, and each with three subcategories.
• Arthur Schopenhauer's doctoral thesis was On the Fourfold Root of the Principle of Sufficient Reason.
• Franz Brentano held that any major philosophical period has four phases: (1) Creative and rapidly progressing with scientific interest and results; then declining through the remaining phases, (2) practical, (3) increasingly skeptical, and (4) literary, mystical, and scientifically worthless – until philosophy is renewed through a new period's first phase. (See Brentano's essay "The Four Phases of Philosophy and Its Current State" 1895, tr. by Mezei and Smith 1998.)
• C. S. Peirce, usually a trichotomist, discussed four methods for overcoming troublesome uncertainties and achieving secure beliefs: (1) the method of tenacity (policy of sticking to initial belief), (2) the method of authority, (3) the method of congruity (following a fashionable paradigm), and (4) the fallibilistic, self-correcting method of science (see "The Fixation of Belief", 1877); and four barriers to inquiry, barriers refused by the fallibilist: (1) assertion of absolute certainty; (2) maintaining that something is absolutely unknowable; (3) maintaining that something is absolutely inexplicable because absolutely basic or ultimate; (4) holding that perfect exactitude is possible, especially such as to quite preclude unusual and anomalous phenomena (see "F.R.L." [First Rule of Logic], 1899).
• Paul Weiss built a system involving four modes of being: Actualities (substances in the sense of substantial, spatio-temporally finite beings), Ideality or Possibility (pure normative form), Existence (the dynamic field), and God (unity). (See Weiss's Modes of Being, 1958).
• Karl Popper outlined a tetradic schema to describe the growth of theories and, via generalization, also the emergence of new behaviors and living organisms: (1) problem, (2) tentative theory, (3) (attempted) error-elimination (especially by way of critical discussion), and (4) new problem(s). (See Popper's Objective Knowledge, 1972, revised 1979.)
• John Boyd (military strategist) made his key concept the decision cycle or OODA loop, consisting of four stages: (1) observation (data intake through the senses), (2) orientation (analysis and synthesis of data), (3) decision, and (4) action. Boyd held that his decision cycle has philosophical generality, though for strategists the point remains that, through swift decisions, one can disrupt an opponent's decision cycle.
• Richard McKeon outlined four classes (each with four subclasses) of modes of philosophical inquiry: (1) Modes of Being (Being); (2) Modes of Thought (That which is); (3) Modes of Fact (Existence); (4) Modes of Simplicity (Experience) – and, corresponding to them, four classes (each with four subclasses) of philosophical semantics: Principles, Methods, Interpretations, and Selections. (See McKeon's "Philosophic Semantics and Philosophic Inquiry" in Freedom and History and Other Essays, 1989.)
• Jonathan Lowe (E.J. Lowe) argues in The Four-Category Ontology, 2006, for four categories: kinds (substantial universals), attributes (relational universals and property-universals), objects (substantial particulars), and modes (relational particulars and property-particulars, also known as "tropes"). (See Lowe's "Recent Advances in Metaphysics," 2001, Eprint)

In technology

• The resin identification code used in recycling to identify low-density polyethylene.
• Most furniture has four legs – tables, chairs, etc.
• Four horses (quadriga) is the maximal number of horses in one row for carriage.
• The four color process (CMYK) is used for printing.
• Wide use of rectangles (with four angles and four sides) because they have effective form and capability for close adjacency to each other (houses, rooms, tables, bricks, sheets of paper, screens, film frames).
• In the Rich Text Format specification, language code 4 is for the Chinese language. Codes for regional variants of Chinese are congruent to 4 mod 256.
• Credit card machines have four-twelve function keys.
• On most phones, the 4 key is associated with the letters G, H, and I, but on the BlackBerry cellular phone, it is the key for D and F.
• On many computer keyboards, the "4" key may also be used to type the dollar sign ($) if the shift key is held down.
• It is the number of bits in a nibble, equivalent to half a byte
• In internet slang, "4" can replace the word "for" (as "four" and "for" are pronounced similarly). For example, typing "4u" instead of "for you".
• In Leetspeak, "4" may be used to replace the letter "A".

In transport

• Most vehicles, including motor vehicles, and particularly cars/automobiles and light commercial vehicles have four road wheels.
• "quattro", meaning four in the Italian language, is used by Audi as a trademark to indicate that four-wheel drive (4WD) technologies are used on Audi-branded cars. The word "Quattro" was initially used by Audi in 1980 in its original 4WD coupé, the Audi Quattro. Audi also has a privately held subsidiary company called quattro GmbH.
• The designation of Interstate 4, a freeway in Florida, the lowest unqualified number assigned and signed in the Interstate Highway System of the United States. (There is an Interstate H-1, Interstate H-2 and Interstate H-3 in Hawaii.)

In sports

• In cricket, a four is a specific type of scoring event, whereby the ball crosses the boundary after touching the ground at least one time, scoring four runs. Taking four wickets in four consecutive balls is typically referred to as a double hat trick (two consecutive, overlapping hat tricks).
• In basketball, the number four is used to designate the Power Forward position, often referred to as "The four spot".
• The basketball term Final Four refers to:
  • in the United States, the last four teams remaining in the NCAA playoff tournament, each of which is the winner of its respective region (for collegiate hockey the term Frozen Four is used).
  • In Europe, the last four teams remaining in many national and transnational club competitions, most notably the Euroleague.
• In rowing, a four refers to a boat for four rowers, with or without coxswain. In rowing nomenclature 4− represents a coxless four and 4+ represents a coxed four.
• There are four bases in the game of baseball: first base, second base, third base, and home plate; to score a run, an offensive player must complete, in the sequence shown, a circuit of those four bases.
• In baseball scoring, number 4 is assigned to the second baseman. Also, four is the most number of runs that can be scored on any single at bat, whereby all three baserunners and the batter score (the most common being via a grand slam).
• In gridiron football codes,
  • four points are awarded in a handful of leagues for rarely attempted types of field goals; an example is in six-man football. Because of the difficulties of getting a successful kick due to the few players on the field, a field goal is worth four points. Also, in Arena Football, a successful dropkicked field goal attempt scores four points.
  • the "four hole" in offense terminology is the space between the right guard and the right tackle on the offensive line
  • the "four back" is an extra running back (outside the fullback and halfback, often referred to as an H-back) in the backfield; e.g. a play call for a "44 lead" indicates the H-back will follow the fullback into the hole between the right guard and the right tackle.
• In rugby union:
  • One of the two starting locks wears the jersey number 4.
  • At several points in the history of the sport, 4 points were awarded for the following scores:
    • A goal from mark from 1891 to 1904 (3 points after that time; abolished in 1977).
    • A drop goal from 1891 to 1947 (now 3 points).
    • A try from 1971 to 1991 (now 5 points).
  • In most league competitions, as well as the pool phases of the Rugby World Cup and many other worldwide championships, 4 competition points are awarded for a win (see rugby union bonus points system).
• In rugby league:
  • A try is worth 4 points.
  • In most competitions (though not the European Super League, which uses static squad numbering), the starting left centre wears jersey number 4.
• The jersey number 4 has been retired by several North American sports teams in honor of past playing greats or other key figures:
  • In Major League Baseball:
    • The Baltimore Orioles, for Hall of Fame manager Earl Weaver.
    • The Boston Red Sox, for Hall of Famer Joe Cronin.
    • The Chicago White Sox, for Hall of Famer Luke Appling.
    • The Los Angeles Dodgers, for Hall of Famer Duke Snider.
    • The Milwaukee Brewers, for Hall of Famer Paul Molitor.
    • The New York Giants, for Hall of Famer Mel Ott; the team has continued to honor the number in its current home of San Francisco.
    • The New York Yankees, for Hall of Famer Lou Gehrig. Notably, he was the first player in major North American sports to be so honored by a U.S.-based team (he was preceded in this honor by Ace Bailey, whose #6 was retired by the Toronto Maple Leafs five years earlier).
    • The Pittsburgh Pirates, for Hall of Famer Ralph Kiner.
  • In the NBA:
    • The Chicago Bulls, for Jerry Sloan, who later served as the team's head coach and went on to a Hall of Fame career in that role (mainly with the Utah Jazz).
    • The Detroit Pistons, for Hall of Fame player Joe Dumars, who later served as team president.
    • The Milwaukee Bucks, for Sidney Moncrief.
    • The New York Nets, for Wendell Ladner, who died in a 1975 plane crash. The team continues to honor the number in its current home of New Jersey.
    • The Sacramento Kings, for Chris Webber.
    • The Utah Jazz, for Hall of Fame player Adrian Dantley.
  • In the NHL:
    • The Boston Bruins, for Hall of Famer Bobby Orr.
    • The Montreal Canadiens, for Hall of Famer Jean Beliveau.
    • The New Jersey Devils, for Hall of Famer Scott Stevens.
    • The Philadelphia Flyers, for Barry Ashbee.
    • The Toronto Maple Leafs have a policy of not retiring numbers unless the player honoured either died or suffered a career-ending incident while a member of the team. Other players whose numbers would otherwise be retired instead have their numbers enshrined by the team as "Honoured Numbers", which remain in circulation for future players. The number 4 is currently honoured for Hall of Famer Red Kelly.
  • In the NFL:
    • The New York Giants, for Hall of Famer Tuffy Leemans.

In other fields

See also 4 (disambiguation).

• The phrase "four-letter word" is used to describe most swear words in the English language, as most swear words do indeed possess four letters.
• Four (四, formal writing: 肆, pinyin sì) is considered an unlucky number in Chinese, Korean, Vietnamese and Japanese cultures because it sounds like the word "death" (死, pinyin sǐ). Due to that, many numbered product lines skip the "four": e.g. Nokia cell phones (there is no series beginning with a 4), Microsoft Windows (which switched to "Windows 95" for version 4^[4]), Palm PDAs, the Leisure Suit Larry games, etc. Some buildings skip floor 4 or replace the number with the letter "F", particularly in heavily Asian areas. See tetraphobia and Numbers in Chinese culture.
• The number of characters in a canonical four-character idiom.
• In the NATO phonetic alphabet, the digit 4 is called "fower".
• In Astrology, Cancer is the 4th astrological sign of the Zodiac.
• In Tarot, The Emperor is the fourth trump or Major Arcana card.
• In Tetris, a game named for the Greek word for 4, every shape in the game is formed of 4 blocks each.
• 4 is one of The Numbers – 4, 8, 15, 16, 23, and 42 – featured in Lost.
• 4 represents the number of Justices on the Supreme Court of the United States necessary to grant a writ of certiorari (i.e., agree to hear a case; it is one less than the number necessary to render a majority decision).

In music

• In classical music, common time is constructed of four beats.
• The number of movements in a symphony.
• The number of completed, numbered symphonies by Johannes Brahms.
• The number of strings on a violin, a viola, a cello, double bass, a cuatro and a ukulele, and the number of string pairs on a mandolin.
• Four, an album by Blues Traveler
• 4, an album by Foreigner
• Miles Davis has a song entitled "Four."
• 4, an album by Beyoncé Knowles
• "Four colly birds" is the gift on the fourth day of Christmas in the carol "The Twelve Days of Christmas"

Groups of four

• Four rules: addition, subtraction, multiplication, division.
• Greek classical elements (fire, air, water, earth).
• Four seasons: spring, summer, autumn, winter.
• Four parts of a day: night, morning, afternoon, evening.
• Approximately Four weeks (4 times 7 days) to a lunar month (synodic month = 29,53 days). Thus the number four is universally an integral part of primitive sacred calenders.
• Four cardinal directions: north, south, east, west.
• Four Temperaments: sanguine, choleric, melancholic, phlegmatic.
• Four Humors: blood, yellow bile, black bile, phlegm.
• Four Great Ancient Capitals of China
• Four corner method
• Cardinal principles
• Four cardinal virtues: justice, prudence, temperance, and fortitude
• Four canonical Christian Gospels, attributed to the Four Evangelists (Matthew, Mark, Luke, and John)
• Four horsemen of the Apocalypse: war, famine, pestilence, death.
• Four suits of playing cards: hearts, diamonds, clubs, spades.
• Four nations of the United Kingdom: England, Wales, Scotland, Northern Ireland.
• Four Noble Truths in the Buddhist religion.
• Four estates: politics, administration, judiciary, journalism. Especially in the expression "Fourth Estate", which means journalism.
• Four Corners is the only location in the United States where four states come together at a single point: Colorado, Utah, New Mexico, and Arizona.
• The Fantastic Four: Mr. Fantastic, The Invisible Woman, The Human Torch, and The Thing.
• The Beatles were also known as the "Fab Four": John Lennon, Ringo Starr, George Harrison, and Paul McCartney.
• Gang of Four is a British post-punk rock band formed in the late 1970s.
• Typically there are four string players in a classical string quartet, usually two violinists, a violist, and a cellist.
• Four rivers in the Garden of Eden (Genesis 2:10–14): Pishon (perhaps the Jaxartes or Syr Darya), Gihon (perhaps the Oxus or Amu Darya), Hiddekel (Tigris), and P'rat (Euphrates)
• There are also four years in a single olympiad (duration between the Olympic Games). The World Cup is held at every four years.

References

• Wells, D. The Penguin Dictionary of Curious and Interesting Numbers London: Penguin Group. (1987): 55 - 58

External links

• Marijn.Org on Why is everything four?
• A few thoughts on the number four, by Penelope Merritt at samuel-beckett.net