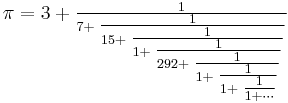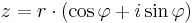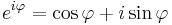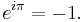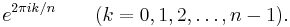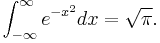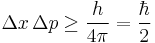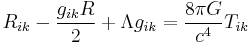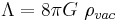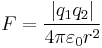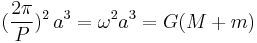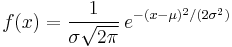Pi

| Part of a series of articles on |
the mathematical constant 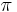 |
|---|
| Uses |
| Area of disk · Circumference Use in other formulae |
| Properties |
| Irrationality · Transcendence Less than 22/7 |
| Value |
| Approximations · Memorization |
| People |
| Archimedes · Zu Chongzhi Madhava of Sangamagrama William Jones · John Machin John Wrench |
| History |
| Chronology · Book |
| In culture |
| Legislation · Holiday |
| Related topics |
| Squaring the circle · Basel problem Other topics related to π |
π (sometimes written pi) is a mathematical constant whose value is the ratio of any circle's circumference to its diameter in Euclidean space; this is the same value as the ratio of a circle's area to the square of its radius. It is approximately equal to 3.141593 in the usual decimal notation. Many formulae from mathematics, science, and engineering involve π, which is one of the most important mathematical and physical constants. Unlike many physical constants, pi is a dimensionless quantity, meaning that it is simply a number without physical units.
π is an irrational number, which means that its value cannot be expressed exactly as a fraction m/n, where m and n are integers. Consequently, its decimal representation never ends or repeats. It is also a transcendental number, which implies, among other things, that no finite sequence of algebraic operations on integers (powers, roots, sums, etc.) can be equal to its value; proving this was a late achievement in mathematical history and a significant result of 19th century German mathematics. Throughout the history of mathematics, there has been much effort to determine π more accurately and to understand its nature; fascination with the number has even carried over into non-mathematical culture.
Probably because of the simplicity of its definition, the concept of π has become entrenched in popular culture to a degree far greater than almost any other mathematical construct.[1] It is, perhaps, the most common ground between mathematicians and non-mathematicians.[2] Reports on the latest, most-precise calculation of π (and related stunts) are common news items.[3][4][5] The current record for the decimal expansion of π, if verified, stands at 5 trillion digits.[6]
The Greek letter π, often spelled out pi in text, was first adopted for the number as an abbreviation of the Greek word for perimeter "περίμετρος" (or as an abbreviation for "perimeter/diameter") by William Jones in 1707. The constant is also known as Archimedes' Constant, after Archimedes of Syracuse, although this name is uncommon in modern English-speaking contexts.
Contents |
Fundamentals
The letter π

The name of the Greek letter π is pi.[7] The name pi is commonly used as an alternative to using the Greek letter. As a mathematical symbol, the Greek letter is not capitalized (Π) even at the beginning of a sentence, and instead the lower case (π) is used at the beginning of a sentence. When referring to this constant, the symbol π is always pronounced "pie" in English, which is the conventional English pronunciation of the Greek letter. The constant is named "π" because "π" is the first letter of the Greek word περίμετρος (perimeter), probably referring to its use in the formula perimeter/diameter which is constant for all circles, the word "perimeter" being synonymous here with "circumference."[8] William Jones was the first to use the Greek letter in this way, in 1707,[9] and it was later popularized by Leonhard Euler in 1737.[10][11] William Jones wrote:
There are various other ways of finding the Lengths or Areas of particular Curve Lines, or Planes, which may very much facilitate the Practice; as for instance, in the Circle, the Diameter is to the Circumference as 1 to ... 3.14159, &c. = π[12]
The capital letter pi (Π) has a completely different mathematical meaning; it is used for expressing products (notice that the word "product" begins with the letter "p" just like "perimeter/diameter" does).
Geometric definition
In Euclidean plane geometry, π is defined as the ratio of a circle's circumference C to its diameter d:[8]
The ratio C/d is constant, regardless of a circle's size. For example, if a circle has twice the diameter d of another circle it will also have twice the circumference C, preserving the ratio C/d.
Alternatively π can be defined as the ratio of a circle's area A to the area of a square whose side is equal to the radius r of the circle:[8][13]
These definitions depend on results of Euclidean geometry, such as the fact that all circles are similar, and the fact that the right-hand-sides of these two equations are equal to each other (i.e. the area of a disk is Cr/2). These two geometric definitions can be considered a problem when π occurs in areas of mathematics that otherwise do not involve geometry. For this reason, mathematicians often prefer to define π without reference to geometry, instead selecting one of its analytic properties as a definition. A common choice is to define π as twice the smallest positive x for which the trigonometric function cos(x) equals zero.[14]



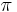 is a transcendental number, squaring the circle is not possible in a finite number of steps using the classical tools of compass and straightedge.
is a transcendental number, squaring the circle is not possible in a finite number of steps using the classical tools of compass and straightedge.Irrationality and transcendence
π is an irrational number, meaning that it cannot be written as the ratio of two integers. π is also a transcendental number, meaning that there is no polynomial with rational coefficients for which π is a root.[15] An important consequence of the transcendence of π is the fact that it is not constructible. Because the coordinates of all points that can be constructed with compass and straightedge are constructible numbers, it is impossible to square the circle: that is, it is impossible to construct, using compass and straightedge alone, a square whose area is equal to the area of a given circle.[16] This is historically significant, for squaring a circle is one of the easily understood elementary geometry problems left to us from antiquity; many amateurs in modern times have attempted to solve each of these problems, and their efforts are sometimes ingenious, but in this case, doomed to failure: a fact not always understood by the amateur involved.
Decimal representation
The decimal representation of π truncated to 50 decimal places is:[17]
Various online web sites provide π to many more digits.[18] While the decimal representation of π has been computed to more than a trillion (1012) digits,[19] elementary applications, such as estimating the circumference of a circle, will rarely require more than a dozen decimal places. For example, the decimal representation of π truncated to 11 decimal places is good enough to estimate the circumference of any circle that fits inside the Earth with an error of less than one millimetre, and the decimal representation of π truncated to 39 decimal places is sufficient to estimate the circumference of any circle that fits in the observable universe with precision comparable to the radius of a hydrogen atom.[20][21]
Because π is an irrational number, its decimal representation does not repeat, and therefore does not terminate. This sequence of non-repeating digits has fascinated mathematicians and laymen alike, and much effort over the last few centuries has been put into computing ever more of these digits and investigating π's properties.[22] Despite much analytical work, and supercomputer calculations that have determined over 1 trillion digits of the decimal representation of π, no simple base-10 pattern in the digits has ever been found.[23] Digits of the decimal representation of π are available on many web pages, and there is software for calculating the decimal representation of π to billions of digits on any personal computer.
Estimating π
| Number system | Approximation of 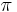 |
|---|---|
| Binary | 11.00100100001111110110...[24] |
| Decimal | 3.14159265358979323846264338327950288... |
| Hexadecimal | 3.243F6A8885A308D31319...[25] |
| Rational approximations | 3, 22⁄7, 333⁄106, 355⁄113, 103993/33102, ...[26]
(listed in order of increasing accuracy) |
| Continued fraction | [3;7,15,1,292,1,1,1,2,1,3,1,14,2,1,1...][27]
(This fraction is not periodic. Shown in linear notation) |
| 3.1415926535 8979323846 2643383279 5028841971 6939937510
5820974944 5923078164 0628620899 8628034825 3421170679 8214808651 3282306647 0938446095 5058223172 5359408128 4811174502 8410270193 8521105559 6446229489 5493038196 4428810975 6659334461 2847564823 3786783165 2712019091 4564856692 3460348610 4543266482 1339360726 0249141273 7245870066 0631558817 4881520920 9628292540 9171536436 7892590360 0113305305 4882046652 1384146951 9415116094 3305727036 5759591953 0921861173 8193261179 3105118548 0744623799 6274956735 1885752724 8912279381 8301194912 9833673362 4406566430 8602139494 6395224737 1907021798 6094370277 0539217176 2931767523 8467481846 7669405132 0005681271 4526356082 7785771342 7577896091 7363717872 1468440901 2249534301 4654958537 1050792279 6892589235 4201995611 2129021960 8640344181 5981362977 4771309960 5187072113 4999999837 2978049951 0597317328 1609631859 5024459455 3469083026 4252230825 3344685035 2619311881 7101000313 7838752886 5875332083 8142061717 7669147303 5982534904 2875546873 1159562863 8823537875 9375195778 1857780532 1712268066 1300192787 6611195909 2164201989 3809525720 1065485863 2788659361 5338182796 8230301952 0353018529 6899577362 2599413891 2497217752 8347913151 5574857242 4541506959 |
|
An estimate of π accurate to 1120 decimal digits was obtained using a gear-driven calculator in 1948, by John Wrench and Levi Smith. This was the most accurate estimate of π before electronic computers came into use.[28]
|
The earliest numerical approximation of π is almost certainly the value 3.[29] In cases where little precision is required, it may be an acceptable substitute. That 3 is an underestimate follows from the fact that it is the ratio of the perimeter of an inscribed regular hexagon to the diameter of the circle.
π can be empirically estimated by drawing a large circle, then measuring its diameter and circumference and dividing the circumference by the diameter. Another geometry-based approach, attributed to Archimedes,[30] is to calculate the perimeter, Pn , of a regular polygon with n sides circumscribed around a circle with diameter d. Then compute the limit of a sequence as n increases to infinity:
This limit converges because the more sides the polygon has, the closer the ratio approaches π. Archimedes determined the accuracy of this approach by comparing the perimeter of the circumscribed polygon with the perimeter of a regular polygon with the same number of sides inscribed inside the circle. Using a polygon with 96 sides, he computed the fractional range:[31]
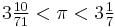 .
.
π can also be calculated using purely mathematical methods. Due to the transcendental nature of π, there are no closed form expressions for the number in terms of algebraic numbers and functions.[15] Formulas for calculating π using elementary arithmetic typically include series or summation notation (such as "..."), which indicates that the formula is really a formula for an infinite sequence of approximations to π.[32] The more terms included in a calculation, the closer to π the result will get.
Most formulae used for calculating the value of π have desirable mathematical properties, but are difficult to understand without a background in trigonometry and calculus. However, some are quite simple, such as this form of the Gregory-Leibniz series:[33]
While that series is easy to write and calculate, it is not immediately obvious why it yields π. In addition, this series converges so slowly that nearly 300 terms are needed to calculate π correctly to 2 decimal places.[34] However, by computing this series in a somewhat more clever way by taking the midpoints of partial sums, it can be made to converge much faster. Let the sequence
and then define
then computing  will take similar computation time to computing 150 terms of the original series in a brute-force manner, and
will take similar computation time to computing 150 terms of the original series in a brute-force manner, and 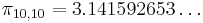 , correct to 9 decimal places. This computation is an example of the van Wijngaarden transformation.[35]
, correct to 9 decimal places. This computation is an example of the van Wijngaarden transformation.[35]
For many purposes, 3.14 or 22/7 is close enough, although engineers often use 3.1416 (5 significant figures) or 3.14159 (6 significant figures) for more precision. The approximations 22/7 and 355/113, with 3 and 7 significant figures respectively, are obtained from the simple continued fraction expansion of π. The approximation 355/113 (3.1415929...) is the best one that may be expressed with a three-digit or four-digit numerator and denominator; the next good approximation 52163/16604 (3.141592387...) requires much bigger numbers, due to the large number 292 in the continued fraction expansion of π.[26]
History

The earliest evidenced conscious use of an accurate approximation for the length of a circumference with respect to its radius is of 3 + 1/7 in the designs of the Old Kingdom pyramids in Egypt. The Great Pyramid at Giza, constructed c.2550-2500 BC, was built with a perimeter of 1760 cubits and a height of 280 cubits; the ratio 1760/280 ≈ 2π. Egyptologists such as Professors Flinders Petrie[36] and I.E.S Edwards[37] have shown that these circular proportions were deliberately chosen for symbolic reasons by the Old Kingdom scribes and architects.[38][39] The same apotropaic proportions were used earlier at the Pyramid of Meidum c.2600 BC. This application is archaeologically evidenced, whereas textual evidence does not survive from this early period.
The early history of π from textual sources roughly parallels the development of mathematics as a whole.[40] Some authors divide progress into three periods: the ancient period during which π was studied geometrically, the classical era following the development of calculus in Europe around the 17th century, and the age of digital computers.[41]
Antiquity
The earliest known textually evidenced approximations date from around 1900 BC; they are 256/81 (Egypt) and 25/8 (Babylonia), both within 1% of the true value.[8] The Indian text Shatapatha Brahmana gives π as 339/108 ≈ 3.139. Additionally, the Old Testament discusses a ceremonial pool in the temple of King Solomon, having a diameter of ten cubits, and a circumference of thirty cubits, implying an approximate value of three for pi;[42][29] though perhaps King Solomon knew better.

Archimedes (287–212 BC) was the first to estimate π rigorously. He realized that its magnitude can be bounded from below and above by inscribing circles in regular polygons and calculating the outer and inner polygons' respective perimeters:[29] By using the equivalent of 96-sided polygons, he proved that 310/71 < π < 31/7.[29] The average of these values is about 3.14185.
Ptolemy, in his Almagest, gives a value of 3.1416, which he may have obtained from Apollonius of Perga.[43]
Around AD 265, the Wei Kingdom mathematician Liu Hui provided a simple and rigorous iterative algorithm to calculate π to any degree of accuracy. He himself carried through the calculation to a 3072-gon (i.e. a 3072-sided polygon) and obtained an approximate value for π of 3.1416.[44] Later, Liu Hui invented a quick method of calculating π and obtained an approximate value of 3.14 with only a 96-gon,[44] by taking advantage of the fact that the difference in area of successive polygons forms a geometric series with a factor of 4.
Around 480, the Chinese mathematician Zu Chongzhi demonstrated that π ≈ 355/113, and showed that 3.1415926 < π < 3.1415927[44] using Liu Hui's algorithm applied to a 12288-gon. This value would remain the most accurate approximation of π available for the next 900 years.
Maimonides mentions with certainty the irrationality of π in the 12th century.[45] This was proved in 1768 by Johann Heinrich Lambert.[46] In the 20th century, proofs were found that require no prerequisite knowledge beyond integral calculus. One of those, due to Ivan Niven, is widely known.[47][48] A somewhat earlier similar proof is by Mary Cartwright.[49]
Second Millennium AD


Until the second millennium AD, estimations of π were accurate to fewer than 10 decimal digits. The next major advances in the study of π came with the development of infinite series and subsequently with the discovery of calculus, which permit the estimation of π to any desired accuracy by considering sufficiently many terms of a relevant series. Around 1400, Madhava of Sangamagrama found the first known such series:
This is now known as the Madhava–Leibniz series[50][51] or Gregory-Leibniz series since it was rediscovered by James Gregory and Gottfried Leibniz in the 17th century. Unfortunately, the rate of convergence is too slow to calculate many digits in practice; about 4,000 terms must be summed to improve upon Archimedes' estimate. However, by transforming the series into
Madhava was able to estimate π as 3.14159265359, which is correct to 11 decimal places. The record was beaten in 1424 by the Persian mathematician, Jamshīd al-Kāshī, who gave an estimate π that is correct to 16 decimal digits.
The first major European contribution since Archimedes was made by the German mathematician Ludolph van Ceulen (1540–1610), who used a geometric method to give an estimate of π that is correct to 35 decimal digits. He was so proud of the calculation, which required the greater part of his life, that he had the digits engraved into his tombstone.[52] Pi is sometimes called "Ludolph's Constant" though not as often as it is called "Archimedes' Constant."[53]
Around the same time, the methods of calculus and determination of infinite series and products for geometrical quantities began to emerge in Europe. The first such representation was the Viète's formula,
found by François Viète in 1593. Another famous result is Wallis' product,
by John Wallis in 1655. Isaac Newton himself derived a series for π and calculated 15 digits, although he later confessed: "I am ashamed to tell you to how many figures I carried these computations, having no other business at the time."[54]
In 1706 John Machin was the first to compute 100 decimals of π, using the inverse trigonometric function arctan in the formula
with
Formulas of this type, now known as Machin-like formulas, were used to set several successive records and remained the best known method for calculating π well into the age of computers. A remarkable record was set by the calculating prodigy Zacharias Dase, who in 1844 employed a Machin-like formula to calculate 200 decimals of π in his head at the behest of Gauss. The best value at the end of the 19th century was due to William Shanks, who took 15 years to calculate π with 707 digits, although due to a mistake only the first 527 were correct. (To avoid such errors, modern record calculations of any kind are often performed twice, with two different formulas. If the results are the same, they are likely to be correct.)
Theoretical advances in the 18th century led to insights about π's nature that could not be achieved through numerical calculation alone. Johann Heinrich Lambert proved the irrationality of π in 1761, and Adrien-Marie Legendre also proved in 1794 π2 to be irrational. When Leonhard Euler in 1735 solved the famous Basel problem, finding the exact value of the Riemann zeta function of 2,
which is π2/6, he established a deep connection between π and the prime numbers. Both Legendre and Euler speculated that π might be transcendental, which was finally proved in 1882 by Ferdinand von Lindemann.
Computation in the computer age

Although practically a physicist needs only 39 digits of Pi to make a circle the size of the observable universe accurate to one atom of hydrogen, the number itself as a mathematical curiosity has created many challenges in different fields.
The advent of digital computers in the 20th century led to an increased rate of new π calculation records. John von Neumann et al. used ENIAC to compute 2037 digits of π in 1949, a calculation that took 70 hours.[55][56] Additional thousands of decimal places were obtained in the following decades, with the million-digit milestone passed in 1973. Progress was not only due to faster hardware, but also new algorithms. One of the most significant developments was the discovery of the fast Fourier transform (FFT) in the 1960s, which allows computers to perform arithmetic on extremely large numbers quickly.
In the beginning of the 20th century, the Indian mathematician Srinivasa Ramanujan found many new formulas for π, some remarkable for their elegance, mathematical depth and rapid convergence.[57] One of his formulas is the series,
where k! is the factorial of k.
A collection of some others are in the table below: [58][59]
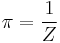 |
 |
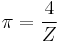 |
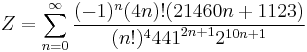 |
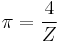 |
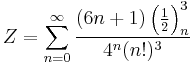 |
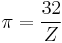 |
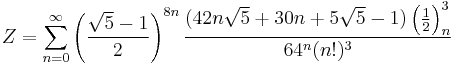 |
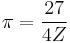 |
 |
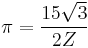 |
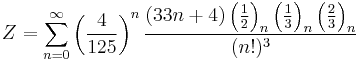 |
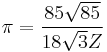 |
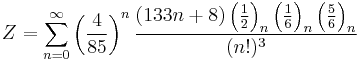 |
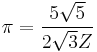 |
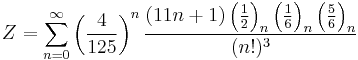 |
 |
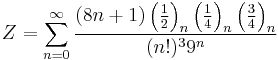 |
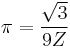 |
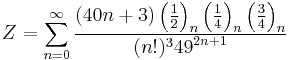 |
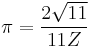 |
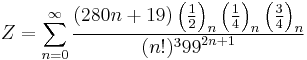 |
 |
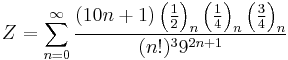 |
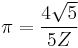 |
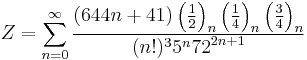 |
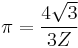 |
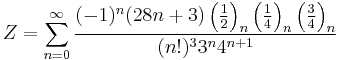 |
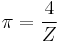 |
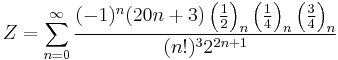 |
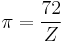 |
 |
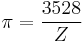 |
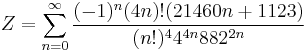 |
where
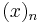
is the pochhammer symbol for the falling factorial.
The related one found by the Chudnovsky brothers in 1987 is
which deliver 14 digits per term.[57] The Chudnovskys used this formula to set several π computing records in the end of the 1980s, including the first calculation of over one billion (1,011,196,691) decimals in 1989. It remains the formula of choice for π calculating software that runs on personal computers, as opposed to the supercomputers used to set modern records. On August 5, 2010, PhysOrg.com reported that Japanese and American computer experts Shigeru Kondo and Alexander Yee said they've calculated the value of π to 5 trillion decimal places on a personal computer, double the previous record.[60]
Whereas series typically increase the accuracy with a fixed amount for each added term, there exist iterative algorithms that multiply the number of correct digits at each step, with the downside that each step generally requires an expensive calculation. A breakthrough was made in 1975, when Richard Brent and Eugene Salamin independently discovered the Brent–Salamin algorithm, which uses only arithmetic to double the number of correct digits at each step.[61] The algorithm consists of setting
and iterating
until an and bn are close enough. Then the estimate for π is given by
Using this scheme, 25 iterations suffice to reach 45 million correct decimals. A similar algorithm that quadruples the accuracy in each step has been found by Jonathan and Peter Borwein.[62] The methods have been used by Yasumasa Kanada and team to set most of the π calculation records since 1980, up to a calculation of 206,158,430,000 decimals of π in 1999. As of January 2010, the record was almost 2.7 trillion digits.[63] This beats the previous record of 2,576,980,370,000 decimals, set by Daisuke Takahashi on the T2K-Tsukuba System, a supercomputer at the University of Tsukuba northeast of Tokyo.[64]
An important recent development was the Bailey–Borwein–Plouffe formula (BBP formula), discovered by Simon Plouffe and named after the authors of the paper in which the formula was first published, David H. Bailey, Peter Borwein, and Simon Plouffe.[65] The formula,
is remarkable because it allows extracting any individual hexadecimal or binary digit of π without calculating all the preceding ones.[65] Between 1998 and 2000, the distributed computing project PiHex used a modification of the BBP formula due to Fabrice Bellard to compute the quadrillionth (1,000,000,000,000,000:th) bit of π, which turned out to be 0.[66]
If a formula of the form
were found where b and c are positive integers and p and q are polynomials with fixed degree and integer coefficients (as in the BPP formula above), this would be one the most efficient ways of computing any digit of π at any position in base bc without computing all the preceding digits in that base, in a time just depending on the size of the integer k and on the fixed degree of the polynomials. Plouffe also describes such formulas as the interesting ones for computing numbers of class SC*, in a logarithmically polynomial space and almost linear time, depending only on the size (order of magnitude) of the integer k, and requiring modest computing resources. The previous formula (found by Plouffe for π with b=2 and c=4, but also found for log(9/10) and for a few other irrational constants), implies that π is a SC* number.
In 2006, Simon Plouffe, using the integer relation algorithm PSLQ, found a series of formulas.[67] Let q = eπ (Gelfond's constant), then
and others of form,
where k is an odd number, and a, b, c are rational numbers.
In the previous formula, if k is of the form 4m + 3, then the formula has the particularly simple form,
for some rational number p where the denominator is a highly factorable number, though no rigorous proof has yet been given.
Pi and continued fraction
The sequence of partial denominators of the simple continued fraction of π does not show any obvious pattern:[27]
or
However, there are generalized continued fractions for π with a perfectly regular structure, such as:[68]
Memorizing digits

Well before computers were used in calculating π, memorizing a record number of digits had become an obsession for some people. In 2006, Akira Haraguchi, a retired Japanese engineer, claimed to have recited 100,000 decimal places.[69] This, however, has yet to be verified by Guinness World Records. The Guinness-recognized record for remembered digits of π is 67,890 digits, held by Lu Chao, a 24-year-old graduate student from China.[70] It took him 24 hours and 4 minutes to recite to the 67,890th decimal place of π without an error.[71]
On June, 17th, 2009 Andriy Slyusarchuk, a Ukrainian neurosurgeon, medical doctor and professor claimed to have memorized 30 million digits of pi, which were printed in 20 volumes of text.[72][73] Although he did not recite the entire 30 million digits that he claims to have memorized, media claim that he was able to recite any randomly selected sequences from the printed text of the 30 million digits[74].
There are many ways to memorize π, including the use of "piems", which are poems that represent π in a way such that the length of each word (in letters) represents a digit. Here is an example of a piem, originally devised by Sir James Jeans: How I want a drink, alcoholic of course, after the heavy lectures involving quantum mechanics.[75][76] Notice how the first word has 3 letters, the second word has 1, the third has 4, the fourth has 1, the fifth has 5, and so on. The Cadaeic Cadenza contains the first 3835 digits of π in this manner.[77] Piems are related to the entire field of humorous yet serious study that involves the use of mnemonic techniques to remember the digits of π, known as piphilology. In other languages there are similar methods of memorization. However, this method proves inefficient for large memorizations of π. Other methods include remembering patterns in the numbers and the method of loci.[78][79]
Open questions
The most pressing open question about π is whether it is a normal number—whether any digit block occurs in the expansion of π just as often as one would statistically expect if the digits had been produced completely "randomly", and that this is true in every integer base, not just base 10.[80] Current knowledge on this point is very weak; e.g., it is not even known which of the digits 0,...,9 occur infinitely often in the decimal expansion of π,[81] although it is clear that at least two such digits must occur infinitely often, since otherwise π would be rational, which it is not.
Bailey and Crandall showed in 2000 that the existence of the above mentioned Bailey-Borwein-Plouffe formula and similar formulas imply that the normality in base 2 of π and various other constants can be reduced to a plausible conjecture of chaos theory.[82]
It is also unknown whether π and e are algebraically independent, although Yuri Nesterenko proved the algebraic independence of {π, eπ, Γ(1/4)} in 1996.[83]
Use in mathematics and science
π is ubiquitous in mathematics, science, and engineering.[84] It appears even in places that lack an obvious connection to the circles of Euclidean geometry.[85]
Geometry and trigonometry
For any circle with radius r and diameter d = 2r, the circumference is πd and the area is πr2. Further, π appears in formulas for areas and volumes of many other geometrical shapes based on circles, such as ellipses, spheres, cones, and tori.[86] Accordingly, π appears in definite integrals that describe circumference, area or volume of shapes generated by circles. In the basic case, half the area of the unit disk is given by the integral:[87] 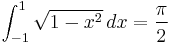 and
and
gives half the circumference of the unit circle.[86] More complicated shapes can be integrated as solids of revolution.[88]
From the unit-circle definition of the trigonometric functions also follows that the sine and cosine have period 2π. That is, for all x and integers n, sin(x) = sin(x + 2πn) and cos(x) = cos(x + 2πn). Because sin(0) = 0, sin(2πn) = 0 for all integers n. Also, the angle measure of 180° is equal to π radians. In other words, 1° = (π/180) radians.
In modern mathematics, π is often defined using trigonometric functions, for example as the smallest positive x for which sin x = 0, to avoid unnecessary dependence on the subtleties of Euclidean geometry and integration. Equivalently, π can be defined using the inverse trigonometric functions, for example as π = 2 arccos(0) or π = 4 arctan(1). Expanding inverse trigonometric functions as power series is the easiest way to derive infinite series for π.
Complex numbers and calculus

A complex number  can be expressed in polar coordinates as follows:
can be expressed in polar coordinates as follows:
The frequent appearance of π in complex analysis can be related to the behavior of the exponential function of a complex variable, described by Euler's formula
where i is the imaginary unit satisfying i2 = −1 and e ≈ 2.71828 is Euler's number. This formula implies that imaginary powers of e describe rotations on the unit circle in the complex plane; these rotations have a period of 360° = 2π. In particular, the 180° rotation φ = π results in the remarkable Euler's identity
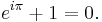
There are n different n-th roots of unity
The Gaussian integral
A consequence is that the gamma function of a half-integer is a rational multiple of √π.
Physics
Although not a physical constant, π appears routinely in equations describing fundamental principles of the Universe, due in no small part to its relationship to the nature of the circle and, correspondingly, spherical coordinate systems. Using units such as Planck units can sometimes eliminate π from formulae.
- Heisenberg's uncertainty principle, which shows that the uncertainty in the measurement of a particle's position (Δx) and momentum (Δp) can not both be arbitrarily small at the same time:[89]
- The cosmological constant Λ from Einstein's field equation is related to the intrinsic energy density of the vacuum ρvac via the gravitational constant G as follows:[91]
- Coulomb's law for the electric force, describing the force between two electric charges (q1 and q2) separated by distance r:[92]
- Magnetic permeability of free space relates the production of a magnetic field in a vacuum by an electric current in units of Newtons (N) and Amperes (A):[93]
- Kepler's third law constant, relating the orbital period (P) and the semimajor axis (a) to the masses (M and m) of two co-orbiting bodies:
Probability and statistics
In probability and statistics, there are many distributions whose formulas contain π, including:
- the probability density function for the normal distribution with mean μ and standard deviation σ, due to the Gaussian integral:[94]
- the probability density function for the (standard) Cauchy distribution:[95]
Note that since 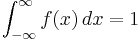 for any probability density function f(x), the above formulas can be used to produce other integral formulas for π.[96]
for any probability density function f(x), the above formulas can be used to produce other integral formulas for π.[96]
Buffon's needle problem is sometimes quoted as an empirical approximation of π in "popular mathematics" works. Consider dropping a needle of length L repeatedly on a surface containing parallel lines drawn S units apart (with S > L). If the needle is dropped n times and x of those times it comes to rest crossing a line (x > 0), then one may approximate π using the Monte Carlo method:[97][98][99][100] 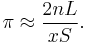 Though this result is mathematically impeccable, it cannot be used to determine more than very few digits of π by experiment. Reliably getting just three digits (including the initial "3") right requires millions of throws,[97] and the number of throws grows exponentially with the number of digits desired. Furthermore, any error in the measurement of the lengths L and S will transfer directly to an error in the approximated π. For example, a difference of a single atom in the length of a 10-centimeter needle would show up around the 9th digit of the result. In practice, uncertainties in determining whether the needle actually crosses a line when it appears to exactly touch it will limit the attainable accuracy to much less than 9 digits.
Though this result is mathematically impeccable, it cannot be used to determine more than very few digits of π by experiment. Reliably getting just three digits (including the initial "3") right requires millions of throws,[97] and the number of throws grows exponentially with the number of digits desired. Furthermore, any error in the measurement of the lengths L and S will transfer directly to an error in the approximated π. For example, a difference of a single atom in the length of a 10-centimeter needle would show up around the 9th digit of the result. In practice, uncertainties in determining whether the needle actually crosses a line when it appears to exactly touch it will limit the attainable accuracy to much less than 9 digits.
Geomorphology and chaos theory
Under ideal conditions (uniform gentle slope on an homogeneously erodible substrate), the ratio between the actual length of a river and its straight-line from source to mouth length tends to approach π.[101] Albert Einstein was the first to suggest that rivers have a tendency towards an ever more loopy path because the slightest curve will lead to faster currents on the outer side, which in turn will result in more erosion and a sharper bend. The sharper the bend, the faster the currents on the outer edge, the more the erosion, the more the river will twist and so on. However, increasing loopiness will result in rivers doubling back on themselves and effectively short-circuiting, creating an ox-bow lake. The balance between these two opposing factors leads to an average ratio of π between the actual length and the direct distance between source and mouth.[102]
In popular culture

Nobel prize winning poet Wisława Szymborska wrote a poem about π, and here is an excerpt:[103]
The caravan of digits that is pi
does not stop at the edge of the page,
but runs off the table and into the air,
over the wall, a leaf, a bird's nest, the clouds, straight into the sky,
through all the bloatedness and bottomlessness.
Oh how short, all but mouse-like is the comet's tail!
Many schools around the world observe Pi Day (March 14, from 3.14).[104] At least one cheer at the Massachusetts Institute of Technology includes "3.14159!"[105]
On November 7, 2005, alternative musician Kate Bush released the album Aerial. The album contains the song "π" whose lyrics consist principally of Bush singing the digits of π to music, beginning with "3.14".[106]
In Carl Sagan's novel Contact, π played a key role in the story. The novel suggested that there was a message buried deep within the digits of π placed there by the creator of the universe. This part of the story was omitted from the film adaptation of the novel.
Darren Aronofsky's film Pi deals with a number theorist.
The Wheel of Dublin Ferris wheel has been nicknamed "the pi in the sky".[107]
See also
| List of numbers – Irrational and suspected irrational numbers γ – ζ(3) – √2 – √3 – √5 – φ – ρ – δS – α – e – π – δ |
- The Feynman point, a sequence of six 9s that appears at the 762nd through 767th decimal places of π
- Indiana Pi Bill
- List of topics related to π
- Proof that 22/7 exceeds π
References
- ↑ See, e.g, Lennart Berggren, Jonathan M. Borwein, and Peter B. Borwein (eds.), Pi: A Source Book. Springer, 1999 (2nd ed.). ISBN 978-0-387-98946-4.
- ↑ See Alfred S. Posamentier and Ingmar Lehmann, Pi: A Biography of the World's Most Mysterious Number. Prometheus Books, 2004. ISBN 978-1-59102-200-8.
- ↑ E.g., MSNBC, Man recites pi from memory to 83,431 places July 3, 2005; Matt Schudel, Obituaries: "John W. Wrench, Jr.: Mathematician Had a Taste for Pi" The Washington Post, March 25, 2009, p. B5.
- ↑ The Big Question: How close have we come to knowing the precise value of pi? The Independent, 8 January 2010
- ↑ Pi, a mathematical story that would take 49,000 years to tell
- ↑ Japanese and US whizzes claim new record for pi calculation http://www.physorg.com/news200209829.html
- ↑ Holton, David; Mackridge, Peter A.; Philippaki-Warburton, Irene (2004). Greek: an essential grammar of the modern language. Routledge essential grammars. Routledge. p. xi. ISBN 0415232104.
- ↑ 8.0 8.1 8.2 8.3 "About Pi". Ask Dr. Math FAQ. http://mathforum.org/dr.math/faq/faq.pi.html. Retrieved 2007-10-29.
- ↑ "About: William Jones". Famous Welsh. http://www.famousWelsh.com/cgibin/getmoreinf.cgi?pers_id=737. Retrieved 2007-10-27.
- ↑ Comanor, Milton; Ralph P. Boas (1976). "Pi". In William D. Halsey. Collier's Encyclopedia. 19. New York: Macmillan Educational Corporation. pp. 21–22.
- ↑ Ben-Menahem, Ari. Historical Encyclopedia of Natural and Mathematical Sciences (2009): "Jones was first to use π for the ratio (perimeter/diameter) of a circle, in 1706."
- ↑ Smith, David Eugene. A source book in mathematics, Volume I, pp. 346-347.
- ↑ Richmond, Bettina (1999-01-12). "Area of a Circle". Western Kentucky University. http://www.wku.edu/~tom.richmond/Pir2.html. Retrieved 2007-11-04.
- ↑ Rudin, Walter (1976) [1953]. Principles of Mathematical Analysis (3e ed.). McGraw-Hill. p. 183. ISBN 0-07-054235-X.
- ↑ 15.0 15.1 Mayer, Steve. "The Transcendence of π". http://dialspace.dial.pipex.com/town/way/po28/maths/docs/pi.html. Retrieved 2007-11-04.
- ↑ "Squaring the Circle". cut-the-knot. http://www.cut-the-knot.org/impossible/sq_circle.shtml. Retrieved 2007-11-04.
- ↑ A000796: Decimal expansion of Pi, On-Line Encyclopedia of Integer Sequences
- ↑ E.g. see “Pi to More Decimal Places Than You Will Ever Need”, University of Exeter, School of Physics, Quantum Physics and Nanomaterials Group (provides pi to one million digits).
- ↑ "Current publicized world record of pi". http://www.super-computing.org/pi_current.html. Retrieved 2007-10-14.
- ↑ Young, Robert M. (1992). Excursions in Calculus. Washington: Mathematical Association of America (MAA). p. 417. ISBN 0883853175. http://books.google.com/?id=iEMmV9RWZ4MC&pg=PA238&dq=intitle:Excursions+intitle:in+intitle:Calculus+39+digits.
- ↑ "Statistical estimation of pi using random vectors". http://scitation.aip.org/getabs/servlet/GetabsServlet?prog=normal&id=AJPIAS000067000004000298000001&idtype=cvips&gifs=yes. Retrieved 2007-08-12.
- ↑ Weisstein, Eric W., "Pi Digits" from MathWorld.
- ↑ Boutin, Chad (2005-04-26). "Pi seems a good random number generator - but not always the best". Purdue University. http://www.purdue.edu/UNS/html4ever/2005/050426.Fischbach.pi.html. Retrieved 2007-11-04.
- ↑ Alexander D. Poularikas (1999). The handbook of formulas and tables for signal processing. CRC Press. p. 9.8. ISBN 9780849385797. http://books.google.com/?id=aaStYSe6WVcC&pg=PT165&dq=11.001001+different-number-bases&q=11.001001%20different-number-bases.
- ↑ "Sample digits for hexa decimal digits of pi". December 6, 2002. http://www.super-computing.org/pi-hexa_current.html.
- ↑ 26.0 26.1 Gourdon, Xavier; Pascal Sebah. "Collection of approximations for π". Numbers, constants and computation. http://numbers.computation.free.fr/Constants/Pi/piApprox.html. Retrieved 2007-11-08.
- ↑ 27.0 27.1 A001203: Continued fraction for Pi, On-Line Encyclopedia of Integer Sequences
- ↑ Wrench, John. "The evolution of extended decimal approximations to π", The Mathematics Teacher, volume 53, pages 644–650 (1960).
- ↑ 29.0 29.1 29.2 29.3 O'Connor, J J; E F Robertson (2001-08). "A history of Pi". http://www-groups.dcs.st-and.ac.uk/~history/HistTopics/Pi_through_the_ages.html. Retrieved 2007-10-30.
- ↑ Groleau, Rick (09-2003). "Infinite Secrets: Approximating Pi". NOVA. http://www.pbs.org/wgbh/nova/archimedes/pi.html. Retrieved 2007-11-04.
- ↑ Beckmann, Petr (1989). A History of Pi. Barnes & Noble Publishing. ISBN 0880294183.
- ↑ Weisstein, Eric W (2007-09-27). "Pi Formulas". MathWorld. http://mathworld.wolfram.com/PiFormulas.html. Retrieved 2007-11-10.
- ↑ Eymard, Pierre; Jean-Pierre Lafon (02 2004). "2.6". The Number π. Stephen S. Wilson (translator). American Mathematical Society. p. 53. ISBN 0821832468. http://books.google.com/?id=qZcCSskdtwcC&pg=PA53&dq=leibniz+pi. Retrieved 2007-11-04.
- ↑ Lampret, Spanish, Vito (2006). "Even from Gregory-Leibniz series π could be computed: an example of how convergence of series can be accelerated" (PDF). Lecturas Mathematicas 27: 21–25. http://www.scm.org.co/Articulos/832.pdf. Retrieved 2007-11-04.
- ↑ A. van Wijngaarden, in: Cursus: Wetenschappelijk Rekenen B, Process Analyse, Stichting Mathematisch Centrum, (Amsterdam, 1965) pp. 51–60.
- ↑ Petrie, W.M.F. 1940 Wisdom of the Egyptians: pp 27
- ↑ Edwards. I.E.S. 1979: The Pyramids of Egypt: pp269
- ↑ Jackson and Stamp (2002) Pyramid: Beyond Imagination. pp153
- ↑ Lightbody, D.I. 2008. Egyptian Tomb Architecture: The Archaeological Facts of Pharaonic Circular Symbolism. British Archaeological Reports. pp36
- ↑ Beckmann, Petr (1976). A History of π. St. Martin's Griffin. ISBN 0-312-38185-9.
- ↑ "Archimedes' constant π". http://numbers.computation.free.fr/Constants/Pi/pi.html. Retrieved 2007-11-04.
- ↑ Kenda, Margaret et al. Math wizardry for kids, page 44 (1995).
- ↑ C. Boyer, A History of Mathematics, Wiley, p. 168.
- ↑ 44.0 44.1 44.2 C. Boyer, A History of Mathematics, Wiley, p. 202.
- ↑ Commentary to Mishneh Torah, beginning of Eruvin
- ↑ Lambert, Johann Heinrich (1761). "Mémoire sur quelques propriétés remarquables des quantités transcendentes circulaires et logarithmiques". Histoire de l'Académie, (Berlin) XVII: pp. 265–322. 1768.
- ↑ Niven, Ivan (1947). "A simple proof that π is irrational" (PDF). Bulletin of the American Mathematical Society 53 (6): 509. doi:10.1090/S0002-9904-1947-08821-2. http://www.ams.org/bull/1947-53-06/S0002-9904-1947-08821-2/S0002-9904-1947-08821-2.pdf. Retrieved 2007-11-04.
- ↑ Richter, Helmut (1999-07-28). "Pi Is Irrational". Leibniz Rechenzentrum. http://www.lrz-muenchen.de/~hr/numb/pi-irr.html. Retrieved 2007-11-04.
- ↑ Jeffreys, Harold (1973). Scientific Inference (3rd ed.). Cambridge University Press.
- ↑ George E. Andrews, Richard Askey, Ranjan Roy (1999). Special Functions. Cambridge University Press. p. 58. ISBN 0521789885.
- ↑ Gupta, R. C. (1992). "On the remainder term in the Madhava-Leibniz's series". Ganita Bharati 14 (1-4): 68–71.
- ↑ Charles Hutton (1811). Mathematical Tables; Containing the Common, Hyperbolic, and Logistic Logarithms.... London: Rivington. p. 13. http://books.google.com/?id=zDMAAAAAQAAJ&pg=PA13&dq=snell+descartes+date:0-1837.
- ↑ Cuyt, Annie et al. Handbook of continued fractions for special functions, page 176 (2008).
- ↑ The New York Times: Even Mathematicians Can Get Carried Away
- ↑ "An {ENIAC} Determination of pi and e to more than 2000 Decimal Places", Mathematical Tables and Other Aids to Computation, 4 (29), pp. 11–15. (January,1950)
- ↑ "Statistical Treatment of Values of First 2,000 Decimal Digits of e and of pi Calculated on the ENIAC", Mathematical Tables and Other Aids to Computation, 4 (30), pp. 109–111. (April,1950)
- ↑ 57.0 57.1 "The constant π: Ramanujan type formulas". http://numbers.computation.free.fr/Constants/Pi/piramanujan.html. Retrieved 2007-11-04.
- ↑ http://www.pi314.net/eng/ramanujan.php
- ↑ http://numbers.computation.free.fr/Constants/Pi/piSeries.html
- ↑ 5 Trillion Digits of Pi - New World Record http://www.numberworld.org/misc_runs/pi-5t/details.html
- ↑ Brent, Richard (1975). "Multiple-precision zero-finding methods and the complexity of elementary function evaluation". Analytic Computational Complexity (New York: Academic Press): pp. 151–176. http://wwwmaths.anu.edu.au/~brent/pub/pub028.html. Retrieved 2007-09-08.
- ↑ Borwein, Jonathan M; Borwein, Peter; Berggren, Lennart (2004). Pi: A Source Book. Springer. ISBN 0387205713.
- ↑ "Pi calculated to 'record number' of digits". bbc.co.uk. 2010-01-06. http://news.bbc.co.uk/1/hi/technology/8442255.stm. Retrieved 2010-01-06.
- ↑ Pi-obsessed Japanese reach 2.5 trillion digits 2009-08-20
- ↑ 65.0 65.1 Bailey, David H.; Borwein, Peter B.; and Plouffe, Simon (April 1997). "On the Rapid Computation of Various Polylogarithmic Constants" (PDF). Mathematics of Computation 66 (218): 903–913. doi:10.1090/S0025-5718-97-00856-9. http://crd.lbl.gov/~dhbailey/dhbpapers/digits.pdf.
- ↑ Bellard, Fabrice. "A new formula to compute the nth binary digit of pi". http://fabrice.bellard.free.fr/pi/pi_bin/pi_bin.html. Retrieved 2007-10-27.
- ↑ Plouffe, Simon. "Indentities inspired by Ramanujan's Notebooks (part 2)". http://www.lacim.uqam.ca/~plouffe/inspired2.pdf. Retrieved 2009-04-10.
- ↑ Lange, L. J. (May 1999). "An Elegant Continued Fraction for π". The American Mathematical Monthly 106 (5): 456–458. doi:10.2307/2589152. http://www.jstor.org/stable/2589152.
- ↑ Otake, Tomoko (2006-12-17). "How can anyone remember 100,000 numbers?". The Japan Times. http://search.japantimes.co.jp/print/fl20061217x1.html. Retrieved 2007-10-27.
- ↑ "Pi World Ranking List". http://www.pi-world-ranking-list.com/news/index.htm. Retrieved 2007-10-27.
- ↑ "Chinese student breaks Guiness record by reciting 67,890 digits of pi". News Guangdong. 2006-11-28. http://www.newsgd.com/culture/peopleandlife/200611280032.htm. Retrieved 2007-10-27.
- ↑ http://vlasti.net/news/49643 Украинец Андрей Слюсарчук установил мировой рекорд — запомнил 30 миллионов цифр
- ↑ http://video.bizua.com.ua/753/index.html Андрей Слюсарчук запоминает 30 млн. цифр после числа Пи, видео
- ↑ http://www.gzt.ru/topnews/science/243760.html Мозг человека может запомнить 30 млн цифр
- ↑ Weisstein, Eric W. "Pi Wordplay." From MathWorld—A Wolfram Web Resource. Retrieved on 2009-03-12.
- ↑ Borwein, Jonathan M (2005-09-25). "The Life of Pi: From Archimedes to Eniac and Beyond" (PDF). Dalhousie University Computer Science. http://users.cs.dal.ca/~jborwein/pi-culture.pdf. Retrieved 2007-10-29.
- ↑ Keith, Mike. "Cadaeic Cadenza Notes & Commentary". http://www.cadaeic.net/comments.htm. Retrieved 2009-07-29.
- ↑ Liu, Yicong (2004-05-19). "Oh my, memorizing so many digits of pi.". Silver Chips Online. http://silverchips.mbhs.edu/inside.php?sid=3577. Retrieved 2007-11-04.
- ↑ Raz A, Packard MG, Alexander GM, Buhle JT, Zhu H, Yu S, Peterson BS. (2009). A slice of pi: An exploratory neuroimaging study of digit encoding and retrieval in a superior memorist. Neurocase. 6:1-12. doi:10.1080/13554790902776896 PMID 19585350
- ↑ Weisstein, Eric W (2005-12-22). "Normal Number". MathWorld. http://mathworld.wolfram.com/NormalNumber.html. Retrieved 2007-11-10.
- ↑ Preuss, Paul (2001-07-23). "Are The Digits of Pi Random? Lab Researcher May Hold The Key". Lawrence Berkeley National Laboratory. http://www.lbl.gov/Science-Articles/Archive/pi-random.html. Retrieved 2007-11-10.
- ↑ Peterson, Ivars (2001-09-01). "Pi à la Mode: Mathematicians tackle the seeming randomness of pi's digits". Science News Online. http://www.sciencenews.org/articles/20010901/bob9.asp. Retrieved 2007-11-10.
- ↑ Nesterenko, Yuri V (1996). "Modular Functions and Transcendence Problems". Comptes rendus de l'Académie des sciences Série 1 322 (10): 909–914.
- ↑ Howard Whitley Eves (1969). An Introduction to the History of Mathematics. Holt, Rinehart & Winston. http://books.google.com/?id=LIsuAAAAIAAJ&q=%22important+numbers+in+mathematics%22&dq=%22important+numbers+in+mathematics%22.
- ↑ "Japanese breaks pi memory record". BBC News. 2005-07-02. http://news.bbc.co.uk/1/hi/world/asia-pacific/4644103.stm. Retrieved 2007-10-30.
- ↑ 86.0 86.1 "Area and Circumference of a Circle by Archimedes". Penn State. http://www.math.psu.edu/courses/maserick/circle/circleapplet.html. Retrieved 2007-11-08.
- ↑ Weisstein, Eric W (2006-01-28). "Unit Disk Integral". MathWorld. http://mathworld.wolfram.com/UnitDiskIntegral.html. Retrieved 2007-11-08.
- ↑ Weisstein, Eric W (2006-05-04). "Solid of Revolution". MathWorld. http://mathworld.wolfram.com/SolidofRevolution.html. Retrieved 2007-11-08.
- ↑ Imamura, James M (2005-08-17). "Heisenberg Uncertainty Principle". University of Oregon. http://zebu.uoregon.edu/~imamura/208/jan27/hup.html. Retrieved 2007-11-09.
- ↑ Einstein, Albert (1916). "The Foundation of the General Theory of Relativity" (PDF). Annalen der Physik. http://www.alberteinstein.info/gallery/gtext3.html. Retrieved 2007-11-09.
- ↑ Perkins, Donald H. (2009). Particle astrophysics. Oxford master series in particle physics, astrophysics, and cosmology. 10 (2nd ed.). Oxford University Press. p. 184. ISBN 0199545456.
- ↑ Nave, C. Rod (2005-06-28). "Coulomb's Constant". HyperPhysics. Georgia State University. http://hyperphysics.phy-astr.gsu.edu/hbase/electric/elefor.html#c3. Retrieved 2007-11-09.
- ↑ "Magnetic constant". NIST. 2006 CODATA recommended values. http://physics.nist.gov/cgi-bin/cuu/Value?mu0. Retrieved 2007-11-09.
- ↑ Weisstein, Eric W (2004-10-07). "Gaussian Integral". MathWorld. http://mathworld.wolfram.com/GaussianIntegral.html. Retrieved 2007-11-08.
- ↑ Weisstein, Eric W (2005-10-11). "Cauchy Distribution". MathWorld. http://mathworld.wolfram.com/CauchyDistribution.html. Retrieved 2007-11-08.
- ↑ Weisstein, Eric W (2003-07-02). "Probability Function". MathWorld. http://mathworld.wolfram.com/ProbabilityFunction.html. Retrieved 2007-11-08.
- ↑ 97.0 97.1 Weisstein, Eric W (2005-12-12). "Buffon's Needle Problem". MathWorld. http://mathworld.wolfram.com/BuffonsNeedleProblem.html. Retrieved 2007-11-10.
- ↑ Bogomolny, Alex (2001-08). "Math Surprises: An Example". cut-the-knot. http://www.cut-the-knot.org/ctk/August2001.shtml. Retrieved 2007-10-28.
- ↑ Ramaley, J. F. (October 1969). "Buffon's Noodle Problem". The American Mathematical Monthly 76 (8): 916–918. doi:10.2307/2317945. http://jstor.org/stable/2317945.
- ↑ "The Monte Carlo algorithm/method". datastructures. 2007-01-09. http://www.datastructures.info/the-monte-carlo-algorithmmethod/. Retrieved 2007-11-07.
- ↑ Hans-Henrik Stølum (1996-03-22). "River Meandering as a Self-Organization Process". Science 271 (5256): 1710–1713. doi:10.1126/science.271.5256.1710.
- ↑ Kaleem Omar (2002-12-10). "Poetic Licence: Pi calculated to 1.24 trillion digits". Daily Times. http://www.dailytimes.com.pk/default.asp?page=story_10-12-2002_pg3_8. Retrieved 2009-12-11.
- ↑ Szymborska, Wislawa. Poems New and Collected, page 174 (2001).
- ↑ Pi Day activities.
- ↑ MIT, E to the U.
- ↑ Blatner, David (2008-03-14). "UK | Magazine | 3.14 and the rest". BBC News. http://news.bbc.co.uk/1/hi/magazine/7296224.stm. Retrieved 2010-01-02.
- ↑ Irish Times, 4 Aug 2010, p.15.
External links
- http://numbers.computation.free.fr/Constants/Pi/pi.html
- Digits of Pi at the Open Directory Project
- Formulas for π at MathWorld
- Representations of Pi at Wolfram Alpha
- Pi at PlanetMath
- Determination of π at Cut-the-knot
- Statistical Distribution Information on PI based on 1.2 trillion digits of PI
- A000796 Decimal expansions of Pi and related links at the On-Line Encyclopedia of Integer Sequences
- Pi Search Engine (2 billion digits)
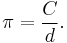
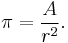
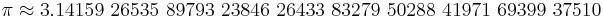
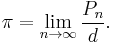
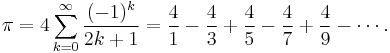
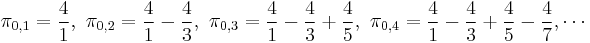

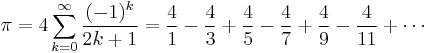


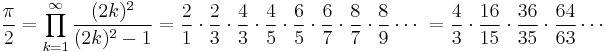
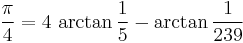
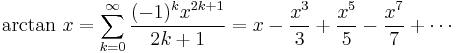
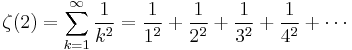
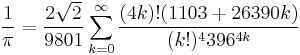
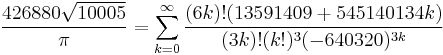
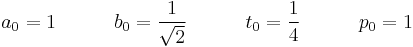

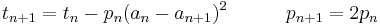
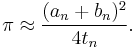
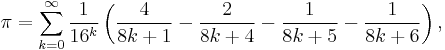
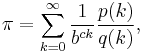
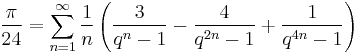
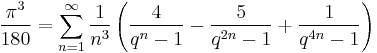

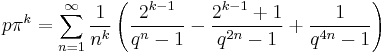
![\pi=[3;7,15,1,292,1,1,1,2,1,3,1,14,2,1,1,2,2,2,2,1,84,\cdots]](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/108f4286d5ad19cd2d6aa6910dbe7431.png)