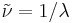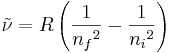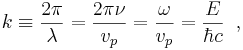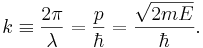Wavenumber

Wavenumber in most physical sciences is a wave property inversely related to wavelength, having SI units of reciprocal meters (m−1). Wavenumber is the spatial analog of frequency, that is, it is the measurement of the number of repeating units of a propagating wave (the number of times a wave has the same phase) per unit of space. Application of a Fourier transformation on data as a function of time yields a frequency spectrum; application on data as a function of position yields a wavenumber spectrum. The exact definition varies depending on the field of study.

Contents

In spectroscopy

In spectroscopy, the wavenumber 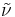\tilde{\nu} of electromagnetic radiation is defined as

\tilde{\nu} = 1/\lambda

where \lambda is the wavelength of the radiation in a vacuum. The wavenumber has dimensions of inverse length and SI units of reciprocal meters (m−1). Commonly, the quantity is expressed in the cgs unit cm−1, pronounced as reciprocal centimeter or inverse centimeter, also formerly called the kayser, after Heinrich Kayser. The historical reason for using this quantity is that it proved to be convenient in the analysis of atomic spectra. Wavenumbers were first used in the calculations of Janne Rydberg in the 1880s. The Rydberg-Ritz combination principle of 1908 was also formulated in terms of wavenumbers. A few years later spectral lines could be understood in quantum theory as differences between energy levels, energy being proportional to wavenumber, or frequency. However, spectroscopic data kept being tabulated in terms of wavenumber rather than frequency or energy, since spectroscopic instruments are typically calibrated in terms of wavelength, independent of the value for the speed of light or Planck's constant.

A wavenumber can be converted into quantum-mechanical energy 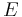E in J or regular frequency \nu in Hz according to

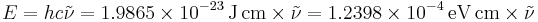E = hc\tilde{\nu} = 1.9865\times 10^{-23} \, \mathrm{J\,cm} \times \tilde{\nu} = 1.2398\times 10^{-4} \,\mathrm{eV\,cm} \times \tilde{\nu},
\nu = c \tilde{\nu} = 29.978\times10^{9} \, \mathrm{Hz\,cm} \times \tilde{\nu}.

Note that here wavenumber and the speed of light are in cgs units, so care must be taken when doing these calculations.

For example, the wavenumbers of the emissions lines of hydrogen atoms are given by

\tilde{\nu} = R\left(\frac{1}{{n_f}^2} - \frac{1}{{n_i}^2}\right)

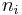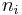where R is the Rydberg constant and n_i and n_f are the principal quantum numbers of the initial and final levels, respectively (n_i is greater than n_f for emission).

In colloquial usage, the unit cm−1 is sometimes referred to as a "wavenumber",[1] which confuses the name of a quantity with that of a unit. Furthermore, spectroscopists often express a quantity proportional to the wavenumber, such as frequency or energy, in cm−1 and leave the appropriate conversion factor as implied. Consequently, an incorrect phrase such as "The energy is 300 wavenumbers" should be interpreted or restated as "The energy corresponds to a wavenumber of 300 reciprocal centimeters (or inverse centimeters or per centimeter)" The analogous statements hold true for the unit m−1.

In wave equations

The angular wavenumber or circular wavenumber, k, often misleadingly abbreviated as "wavenumber", is defined as

k \equiv \frac{2\pi}{\lambda}

for a wave of wavelength \lambda.

For the special case of an electromagnetic wave,

k \equiv \frac{2\pi}{\lambda} = \frac{2\pi\nu}{v_p}=\frac{\omega}{v_p}=\frac{E}{\hbar c}\;\;,

where \nu (Greek letter nu) is the frequency of the wave, vp is the phase velocity of the wave, ω is the angular frequency of the wave, E is the energy of the wave, ħ is the reduced Planck constant, and c is the speed of light in vacuum. If the electromagnetic wave travels in vacuum, its phase velocity vp = c. The wavenumber is the magnitude of the wave vector.

For the special case of a matter wave, for example an electron wave, in the non-relativistic approximation:

k \equiv \frac{2\pi}{\lambda} = \frac{p}{\hbar}= \frac{\sqrt{2 m E }}{\hbar}.

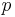Here p is the momentum of the particle, 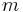m is the mass of the particle, 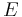E is the kinetic energy of the particle, and 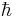\hbar is the reduced Planck's constant.

In atmospheric science

Wavenumber in atmospheric science is defined as length of the spatial domain divided by the wavelength, or equivalently the number of times a wave has the same phase over the spatial domain. The domain might be 2π for the non-dimensional case, or

2\pi R \cos\left(\phi\right)

for an atmospheric wave, where R is Earth's radius and φ is latitude. Wavenumber-frequency diagrams are a common way of visualizing atmospheric waves.

See also

References

  1. ↑ For example J. Quantitative Spectroscopy and Radiative Transfer 68, 543 (2001); US patent 5046846: Method and apparatus for spectroscopic comparison of compositions; an editorial comment in Science 308, 1221 (2005).