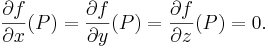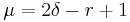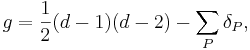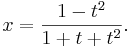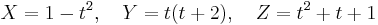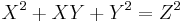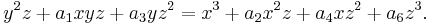Algebraic curve

In algebraic geometry, an algebraic curve is an algebraic variety of dimension one. The theory of these curves in general was quite fully developed in the nineteenth century, after many particular examples had been considered, starting with circles and other conic sections.

Contents

Plane algebraic curves

An algebraic curve defined over a field F may be considered as the locus of points in Fn determined by at least n−1 independent polynomial functions in n variables with coefficients in F, gi(x1, …, xn), where the curve is defined by setting each gi = 0.

Using the resultant, we can eliminate all but two of the variables and reduce the curve to a birationally equivalent plane curve, f(x,y) = 0, still with coefficients in F, but usually of higher degree, and often possessing additional singularities. For example, eliminating z between the two equations x2+y2−z2 = 0 and x+2y+3z−1 = 0, which defines an intersection of a cone and a plane in three dimensions, we obtain the conic section 8x2+5y2−4xy+2x+4y−1 = 0, which in this case is an ellipse. If we eliminate z between 4x2+y2−z2 = 1 and z = x2, we obtain y2 = x4−4x2+1, which is the equation of a hyperelliptic curve.

Projective curves

It is often desirable to consider that curves are a locus of points in projective space. In the set of equations gi = 0, we can replace each xk with xk/x0, and multiply by x0n, where n is the degree of gi. In this way we obtain homogeneous polynomial functions, which define the corresponding curve in projective space. For a plane algebraic curve we have a single equation f(x,y,z) = 0, where f is homogeneous; for example, the Fermat curve xn+yn+zn = 0 is a projective curve.

Algebraic function fields

The study of algebraic curves can be reduced to the study of irreducible algebraic curves. Up to birational equivalence, these are categorically equivalent to algebraic function fields. An algebraic function field is a field of algebraic functions in one variable K defined over a given field F. This means there exists an element x of K which is transcendental over F, and such that K is a finite algebraic extension of F(x), which is the field of rational functions in the indeterminate x over F.

For example, consider the field C of complex numbers, over which we may define the field C(x) of rational functions in C. If y2 = x3−x−1, then the field C(x,y) is an elliptic function field. The element x is not uniquely determined; the field can also be regarded, for instance, as an extension of C(y). The algebraic curve corresponding to the function field is simply the set of points (x,y) in C2 satisfying y2 = x3−x−1.

If the field F is not algebraically closed, the point of view of function fields is a little more general than that of considering the locus of points, since we include, for instance, "curves" with no points on them. If the base field F is the field R of real numbers, then x2+y2 = −1 defines an algebraic extension field of R(x), but the corresponding curve considered as a locus has no points in R. However, it does have points defined over the algebraic closure C of R.

Complex curves and real surfaces

A complex projective algebraic curve resides in n-dimensional complex projective space CPn. This has complex dimension n, but topological dimension, as a real manifold, 2n, and is compact, connected, and orientable. An algebraic curve likewise has topological dimension two; in other words, it is a surface. A nonsingular complex projective algebraic curve will then be a smooth orientable surface as a real manifold, embedded in a compact real manifold of dimension 2n which is CPn regarded as a real manifold. The topological genus of this surface, that is the number of handles or donut holes, is the genus of the curve. By considering the complex analytic structure induced on this compact surface we are led to the theory of compact Riemann surfaces.

Compact Riemann surfaces

A Riemann surface is a connected complex analytic manifold of one complex dimension, which makes it a connected real manifold of two dimensions. It is compact if it is compact as a topological space.

There is a triple equivalence of categories between the category of smooth projective algebraic curves over the complex numbers, the category of compact Riemann surfaces, and the category of complex algebraic function fields, so that in studying these subjects we are in a sense studying the same thing. This allows complex analytic methods to be used in algebraic geometry, and algebraic-geometric methods in complex analysis, and field-theoretic methods to be used in both, which is characteristic of a much wider class of problems than simply curves and Riemann surfaces.

Singularities

Using the intrinsic concept of tangent space, points P on an algebraic curve C are classified as smooth or non-singular, or else singular. Given n−1 homogeneous polynomial functions in n+1 variables, we may find the Jacobian matrix as the (n−1)×(n+1) matrix of partial derivatives. If the rank of this matrix at a point P on the curve has the maximal value of n−1, then the point is a smooth point. In particular, if the curve is a plane projective algebraic curve, defined by a single homogeneous polynomial equation f(x,y,z) = 0, then the singular points are precisely the points P where the rank of the 1×(n+1) matrix is zero, that is, where

\frac{ \partial f }{ \partial x }(P)=\frac{ \partial f }{ \partial y }(P)=\frac{ \partial f }{ \partial z }(P)=0.

Since f is a polynomial, this definition is purely algebraic and makes no assumption about the nature of the field F, which in particular need not be the real or complex numbers. It should of course be recalled that (0,0,0) is not a point of the curve and hence not a singular point.

The singularities of a curve are not birational invariants. However, locating and classifying the singularities of a curve is one way of computing the genus, which is a birational invariant. For this to work, we should consider the curve projectively and require F to be algebraically closed, so that all the singularities which belong to the curve are considered.

Classification of singularities

x3 = y2

Singular points include multiple points where the curve crosses over itself, and also various types of cusp, for example that shown by the curve with equation x3 = y2 at (0,0).

A curve C has at most a finite number of singular points. If it has none, it can be called smooth or non-singular. For this definition to be correct, we must use an algebraically closed field and a curve C in projective space (i.e., complete in the sense of algebraic geometry). If, for example, we simply look at a curve in the real affine plane there might be singular P modulo the stalk, or alternatively as the sum of m(m−1)/2, where m is the multiplicity, over all infinitely near singular points Q lying over the singular point P. Intuitively, a singular point with delta invariant δ concentrates δ ordinary double points at P.

The Milnor number μ of the singularity is the degree of the mapping grad f(x,y)/|grad f(x,y)| on the small sphere of radius ε, in the sense of the topological degree of a continuous mapping, where grad f is the (complex) gradient vector field of f. It is related to δ and r by the Milnor-Jung formula,

\mu = 2\delta - r + 1

Another singularity invariant of note is the multiplicity m, defined as the maximum integer such that the derivatives of f to all orders up to m vanish.

Computing the delta invariants of all of the singularities allows the genus g of the curve to be determined; if d is the degree, then

g = \frac{1}{2}(d-1)(d-2) - \sum_P \delta_P,

where the sum is taken over all singular points P of the complex projective plane curve.

Singularities may be classified by the triple [m, δ, r], where m is the multiplicity, δ is the delta-invariant, and r is the branching number. In these terms, an ordinary cusp is a point with invariants [2,1,1] and an ordinary double point is a point with invariants [2,1,2]. An ordinary n-multiple point may be defined as one having invariants [n, n(n−1)/2, n].

Examples of curves

Rational curves

A rational curve, also called a unicursal curve, is any curve which is birationally equivalent to a line, which we may take to be a projective line and identify with the field of rational functions in one indeterminate F(x). If F is algebraically closed, this is equivalent to a curve of genus zero; however the field R(x,y) with x2+y2 = −1 is a field of genus zero which is not a rational function field.

Concretely, a rational curve of dimension n over F can be parameterized (except for isolated exceptional points) by means of n rational functions defined in terms of a single parameter t; by clearing denominators we can turn this into n+1 polynomial functions in projective space. An example would be the rational normal curve.

Any conic section defined over F with a rational point in F is a rational curve. It can be parameterized by drawing a line with slope t through the rational point, and intersection with the plane quadratic curve; this gives a polynomial with F-rational coefficients and one F-rational root, hence the other root is F-rational (i.e., belongs to F) also.

x2 + xy + y2 = 1

For example, consider the ellipse x2 + xy + y2 = 1, where (−1, 0) is a rational point. Drawing a line with slope t from (−1,0), y = t(x+1), substituting it in the equation of the ellipse, factoring, and solving for x, we obtain

x = \frac{1-t^2}{1+t+t^2}.

We then have that the equation for y is

y=t(x+1)=\frac{t(t+2)}{1+t+t^2}

which defines a rational parameterization of the ellipse and hence shows the ellipse is a rational curve. All points of the ellipse are given, except for (−1,1), which corresponds to t = ∞; the entire curve is parameterized therefore by the real projective line.

Viewing rational parameterizations with rational coefficients projectively, we can view them as giving number theoretical information about homogeneous equations defined over the integers. For example from the above, we obtain

X=1-t^2,\quad Y=t(t+2),\quad Z=t^2+t+1 \,\!

for which

X^2+XY+Y^2=Z^2 \,\!

is true for integer X, Y and Z if t is an integer. Hence we obtain triangles with integer length sides, such as sides of length 3, 7, and 8, where one of the angles is 60°, from relationships such as 82−3·8+32 = 72.

Many of the curves on Wikipedia's list of curves are rational, and hence have similar rational parameterizations.

Elliptic curves

An elliptic curve may be defined as any curve of genus one with a rational point: a common model is a nonsingular cubic curve, which suffices to model any genus one curve. In this model the distinguished point is commonly taken to be an inflection point at infinity; this amounts to requiring that the curve can be written in Tate-Weierstrass form, which in its projective version is

y^2z + a_1 xyz + a_3 yz^2 = x^3 + a_2 x^2z + a_4 xz^2 + a_6 z^3. \,\!

Elliptic curves carry the structure of an abelian group with the distinguished point as the identity of the group law. In a plane cubic model three points sum to zero in the group if and only if they are collinear. For an elliptic curve defined over the complex numbers the group is isomorphic to the additive group of the complex plane modulo the period lattice of the corresponding elliptic functions.

Curves of genus greater than one

Curves of genus greater than one differ markedly from both rational and elliptic curves. Such curves defined over the rational numbers, by Faltings' theorem, can have only a finite number of rational points, and they may be viewed as having a hyperbolic geometry structure. Examples are the hyperelliptic curves, the Klein quartic curve, and the Fermat curve xn+yn = zn when n is greater than three.

See also

  • Acnode
  • Bézout's theorem
  • Birational geometry
  • Conic section
  • Cubic plane curve
  • Crunode
  • Curve
  • Elliptic curve
  • Faltings' theorem
  • Fractional ideal
  • Function field of an algebraic variety
  • Function field (scheme theory)
  • Genus (mathematics)
  • Hilbert's sixteenth problem
  • Hyperelliptic curve
  • Jacobian variety
  • Klein quartic
  • List of curves
  • Quartic plane curve
  • Rational normal curve
  • Riemann-Hurwitz formula
  • Riemann–Roch theorem
  • Riemann surface
  • Weber's theorem

References