Binding energy

Binding energy is the mechanical energy required to disassemble a whole into separate parts. A bound system has a lower potential energy than its constituent parts; this is what keeps the system together. The usual convention is that this corresponds to a positive binding energy.

In general, binding energy represents the mechanical work which must be done in acting against the forces which hold an object together, while disassembling the object into component parts separated by sufficient distance that further separation requires negligible additional work.

Electron binding energy is a measure of the energy required to free electrons from their atomic orbits.

Nuclear binding energy is derived from the strong nuclear force and is the energy required to disassemble a nucleus into free unbound neutrons and protons, strictly so that the relative distances of the particles from each other are infinite (essentially far enough so that the strong nuclear force can no longer cause the particles to interact).

At the atomic level, the atomic binding energy of the atom derives from electromagnetic interaction and is the energy required to disassemble an atom into free electrons and a nucleus.

In astrophysics, gravitational binding energy of a celestial body is the energy required to disassemble it into space debris (dust and gas). This quantity is not to be confused with the gravitational potential energy, which is the energy required to separate two bodies, such as a celestial body and a satellite, to infinite distance, keeping each intact (the latter energy is lower).

1 Mass defect
2 Nucleus binding energy
- 2.1 Practice: Binding energy for atoms
- 2.2 Specific quantitative example: a deuteron
3 Nuclear binding energy curve
- 3.1 Semiempirical formula
- 3.2 Measuring the binding energy
4 References
5 External links
6 See also

Mass defect

Because a bound system is at a lower energy level than its unbound constituents, its mass must be less than the total mass of its unbound constituents. For systems with low binding energies, this "lost" mass after binding may be fractionally small. For systems with high binding energies, however, the missing mass may be an easily measurable fraction.

Since all forms of energy have mass, the question of where the missing mass of the binding energy goes is of interest. The answer is that this mass transforms to heat, light, higher energy states of the nucleus/atom or other forms of energy. The "mass defect" from binding energy is therefore mass that transforms to energy according to Einstein's equation. Once the system cools to normal temperatures and returns to ground states in terms of energy levels, there is less mass remaining in the system than there was when it first combined and was at high energy. Mass measurements, almost always made at low temperatures with systems in ground states, so this difference between the mass of a system and the sum of the masses of its isolated parts is called a mass deficit. Thus, if binding energy mass is transformed into heat, the system must be cooled (the heat removed) before the mass-deficit appears in the cooled system. In that case, the removed heat represents exactly the mass "deficit".

As an illustration, consider two objects attracting each other in space through their gravitational field. The attraction force accelerates the objects and they gain some speed toward each other converting the potential (gravity) energy into kinetic (movement) energy. Either the praticles 1) pass through each other without interaction or 2) elastically repel during the collision, the gained kinetic energy (related to speed), starts to revert into potential form driving the collided particles apart. The deaccelerating particles will return to the initial distance and beyond into infinity or stop and repeat the collision (oscillation takes place). This shows that the system, which loses no energy, does not combine (bind) into a solid object, parts of which oscillate at short distances. Therefore, in order to bind the particles, the kinetic energy gained due to the attraction must be dissipated (by resistive force). Usually, the objects in collision do smash in some extent transforming the kinetic energy into internal energy (the atomic movement), which is further radiated in the form of photons -- the light and heat. Once the momentum to escape the gravity is dissipated in the collision, the parts will oscillate at closer, possibly atomic, distance, thus looking as one solid object. This lost energy, necessary to overcome the potential barrier in order to separate the objects, that is the binding energy.

Closely analogous considerations apply in chemical and nuclear considerations. However, in nuclear reactions, the fraction of mass that may be removed as light or heat, i.e., binding energy, is often a much larger fraction of the system mass. This is because nuclear forces are comparatively stronger than Coulombic forces associated with electrons and protons.

In nuclear reactions, the energy that must be radiated or otherwise removed as binding energy may be in the form of electromagnetic waves, such as gamma radiation, or as heat. Again, however, no mass-deficit can in theory appear until this radiation has been emitted and is no longer part of the system.

The energy given off during either nuclear fusion or nuclear fission is the difference between the binding energies of the fuel and the fusion or fission products. In practice, this energy may also be calculated from the substantial mass differences between the fuel and products, once evolved heat and radiation have been removed.

Nucleus binding energy

Practice: Binding energy for atoms

The measured mass deficits of isotopes are always listed as mass deficits of the neutral atoms of that isotope, and mostly in MeV. As a consequence, the listed mass deficits are not a measure for the stability or binding energy of isolated nuclei, but for the whole atoms. This has very practical reasons, because it is very hard to totally ionize heavy elements, i.e. strip them of all of their electrons.

This practice is useful for other reasons, too: Stripping all the electrons from a heavy unstable nucleus (thus producing a bare nucleus) will change the lifetime of the nucleus, indicating that the nucleus cannot be treated independently (Experiments at the heavy ion accelerator GSI). This is also evident from phenomena like electron capture. Theoretically, in orbital models of heavy atoms, the electron orbits partially inside the nucleus (it doesn't orbit in a strict sense, but has a non-vanishing probability of being located inside the nucleus).

Of course, a nuclear decay happens to the nucleus, meaning that properties ascribed to the nucleus will change in the event. But for the following considerations and examples, you should keep in mind that "mass deficit" as a measure for "binding energy", and as listed in nuclear data tables, means "mass deficit of the neutral atom" and is a measure for stability of the whole atom.

Specific quantitative example: a deuteron

A deuteron (the nucleus of a deuterium atom, with no electron) consists of one proton and one neutron. The experimentally-measured masses of the constituents as free particles are

m_proton = 1.007825 u;

m_neutron= 1.008665 u;

m_proton + m_neutron = 1.007825 + 1.008665 = 2.01649 u.

The mass of the deuteron (also an experimentally measured quantity) is

Atomic mass ²H = 2.014102 u.

The mass difference = 2.01649−2.014102 u = 0.002388 u. Since the conversion between rest mass and energy is 931.494MeV/u, a deuteron's binding energy is calculated to be

0.002388 u × 931.494 MeV/u = 2.224 MeV.

Thus, expressed in another way, the binding energy is [0.002388/2.01649] x 100% = about 0.1184% of the total energy corresponding to the mass. This corresponds to 1.7×10¹⁴ J/kg = 107 TJ/kg.

Nuclear binding energy curve

Binding energy per nucleon of common isotopes (cf. large version of figure below).

In the periodic table of elements, the series of light elements from hydrogen up to sodium is observed to exhibit generally increasing binding energy per nucleon as the atomic mass increases. This increase is generated by increasing forces per nucleon in the nucleus, as each additional nucleon is attracted by all of the other nucleons, and thus more tightly bound to the whole.

The region of increasing binding energy is followed by a region of relative stability (saturation) in the sequence from magnesium through xenon. In this region, the nucleus has become large enough that nuclear forces no longer completely extend efficiently across its width. Attractive nuclear forces in this region, as atomic mass increases, are nearly balanced by repellent electromagnetic forces between protons, as atomic number increases.

Finally, in elements heavier than xenon, there is a decrease in binding energy per nucleon as atomic number increases. In this region of nuclear size, electromagnetic repulsive forces are beginning to gain against the strong nuclear force.

At the peak of binding energy, nickel-62 is the most tightly-bound nucleus, followed by iron-58 and iron-56.^[1] (This is the basic reason why iron and nickel are very common metals in planetary cores, since they are produced profusely as end products in supernovae and in the final stages of Silicon burning in Stars).

The existence of a maximum in binding energy in medium-sized nuclei is a consequence of the trade-off in the effects of two opposing forces which have different range characteristics. The attractive nuclear force (strong nuclear force), which binds protons and neutrons equally to each other, has a limited range due to a rapid exponential decrease in this force with distance. However, the repelling electromagnetic force, which acts between protons to force nuclei apart, falls off with distance much more slowly (as the inverse square of distance). For nuclei larger than about four nucleons in diameter, the additional repelling force of additional protons more than offsets any binding energy which results between further added nucleons as a result of additional strong force interactions; such nuclei become less and less tightly bound as their size increases, though most of them are still stable. Finally, nuclei containing more than 209 nucleons (larger than about 6 nucleons in diameter) are all too large to be stable, and are subject to spontaneous decay to smaller nuclei.

Nuclear fusion produces energy by combining the very lightest elements into more tightly-bound elements (such as hydrogen into helium), and nuclear fission produces energy by splitting the heaviest elements (such as uranium and plutonium) into more tightly-bound elements (such as barium and krypton). Both processes produce energy, because middle-sized nuclei are the most tightly bound of all.

Semiempirical formula

For more details on this topic, see Semi-empirical mass formula.

For a nucleus with A nucleons including Z protons, a semiempirical formula for the binding energy per nucleon (E/A) is:

$E/A=a-b/\sqrt[3]{A}-c(Z/\sqrt[3]{A^2})^2-d(N-Z)^2/\sqrt[3]{A^{3+3}}\pm e/\sqrt[4]{A^{3+4}}$

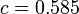where the binding energy is in MeV for the following numerical values of the constants: $a=14.0$ ; $b=13.0$ ; $c=0.585$ ; $d=19.3$ ; $e=33$ .

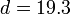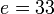Most terms in this formula can be explained by the liquid drop model for the nucleus, which treats the nucleus as a drop of uniform, incompressible fluid, whose radius can be derived from its density.

The first term 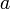$a\,$ is called the saturation contribution and ensures that the binding energy (B.E.) per nucleon is the same for all nuclei, to a first approximation.

The term 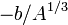$-b/A^{1/3}\,$ is a surface tension effect and is proportional to the number of nucleons that are situated on the nuclear surface. It is largest for light nuclei.

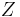The term $-cZ^2/A^{4/3}\,$ is the Coulomb electrostatic repulsion. This becomes more important as $Z$ increases.

The symmetry correction term 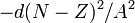$-d(N-Z)^2/A^2\,$ takes into account Pauli's exclusion principle. In the absence of other effects the most stable arrangement (i.e. one with lowest energy) has equal numbers of protons and neutrons.

The pairing term 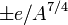$\pm e/A^{7/4}$ is + for even-even nuclei and − for odd-odd nuclei. This too is a result of Pauli's exclusion principle, together with the protons and neutrons having spin 1/2.

The following table gives the binding energy per nucleon in MeV for selected isotopes.

	Formula	Measured
²⁷Al	8.42	8.33
⁶³Cu	8.75	8.75
⁹⁸Mo	8.62	8.63
¹⁹⁵Pt	7.87	7.92
²³⁸U	7.56	7.58

Measuring the binding energy

As seen above in the example of deuterium, nuclear binding energies are large enough that they may be easily measured as fractional mass deficits, according to the equivalence of mass and energy. The atomic binding energy is simply the amount of energy (and mass) released, when a collection of free nucleons are joined together to form a nucleus.

Nuclear binding energy can be easily computed from the easily measurable difference in mass of a nucleus, and the sum of the masses of the number of free neutrons and protons that make up the nucleus. Once this mass difference, called the mass defect or mass deficiency, is known, Einstein's mass-energy equivalence formula E = mc² can be used to compute the binding energy of any nucleus. (As a historical note, early nuclear physicists used to refer to computing this value as a "packing fraction" calculation.)

For example, the atomic mass unit (1.000000 u) is defined to be 1/12 of the mass of a ¹²C atom—but the atomic mass of a ¹H atom (which is a proton plus electron) is 1.007825 u, so each nucleon in ¹²C has lost, on average, about 0.8% percent of its mass in the form of binding energy.

References

↑ Fewell, M. P. (1995). "The atomic nuclide with the highest mean binding energy". American Journal of Physics 63 (7): 653–658. doi:10.1119/1.17828. http://adsabs.harvard.edu/abs/1995AmJPh..63..653F.