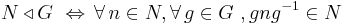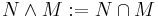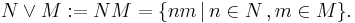Normal subgroup

Basic notions in group theory
category of groups
subgroups, normal subgroups
quotient groups
group homomorphisms, kernel, image
(semi-)direct product, direct sum
types of groups
simple,
finite, infinite
discrete, continuous
multiplicative, additive
cyclic, abelian, nilpotent, solvable

In mathematics, more specifically in abstract algebra, a normal subgroup is a special kind of subgroup. Normal subgroups are important because they can be used to construct quotient groups from a given group.

Évariste Galois was the first to realize the importance of the existence of normal subgroups.

Contents

Definitions

A subgroup N of a group G is called a normal subgroup if it is invariant under conjugation; that is, for each element n in N and each g in G, the element gng−1 is still in N. We write

N \triangleleft G\,\,\Leftrightarrow\,\forall\,n\in{N},\forall\,g\in{G}\ , gng^{-1}\in{N}

The following conditions are equivalent to requiring that a subgroup N be normal in G. Any one of them may be taken as the definition:

  1. For all g in G, gNg−1 ⊆ N.
  2. For all g in G, gNg−1 = N.
  3. The sets of left and right cosets of N in G coincide.
  4. For all g in G, gN = Ng.
  5. N is a union of conjugacy classes of G.
  6. There is some homomorphism on G for which N is the kernel.

Note that condition (1) is logically weaker than condition (2), and condition (3) is logically weaker than condition (4). For this reason, conditions (1) and (3) are often used to prove that N is normal in G, while conditions (2) and (4) are used to prove consequences of the normality of N in G.

Examples

Properties

Lattice of normal subgroups

The normal subgroups of a group G form a lattice under subset inclusion with least element {e} and greatest element G. Given two normal subgroups N and M in G, meet is defined as

N \wedge M�:= N \cap M

and join is defined as

N \vee M�:= N M = \{nm \,|\, n \in N \,, m \in M\}.

The lattice is complete and modular.

Normal subgroups and homomorphisms

Normal subgroups are of relevance because if N is normal, then the quotient group G/N may be formed: if N is normal, we can define a multiplication on cosets by

(a1N)(a2N) := (a1a2)N.

This turns the set of cosets into a group called the quotient group G/N. There is a natural homomorphism f : G → G/N given by f(a) = aN. The image f(N) consists only of the identity element of G/N, the coset eN = N.

In general, a group homomorphism f: G → H sends subgroups of G to subgroups of H. Also, the preimage of any subgroup of H is a subgroup of G. We call the preimage of the trivial group {e} in H the kernel of the homomorphism and denote it by ker(f). As it turns out, the kernel is always normal and the image f(G) of G is always isomorphic to G/ker(f) (the first isomorphism theorem). In fact, this correspondence is a bijection between the set of all quotient groups G/N of G and the set of all homomorphic images of G (up to isomorphism). It is also easy to see that the kernel of the quotient map, f: G → G/N, is N itself, so we have shown that the normal subgroups are precisely the kernels of homomorphisms with domain G.

See also

Operations taking subgroups to subgroups:

  • normalizer
  • conjugate closure
  • normal core

Subgroup properties stronger than normality:

  • characteristic subgroup
  • fully characteristic subgroup

Subgroup properties weaker than normality:

  • subnormal subgroup
  • ascendant subgroup
  • descendant subgroup
  • serial subgroup
  • quasinormal subgroup
  • seminormal subgroup
  • conjugate permutable subgroup
  • modular subgroup
  • pronormal subgroup
  • paranormal subgroup
  • polynormal subgroup
  • c normal subgroup

Subgroup properties complementary (or opposite) to normality:

  • malnormal subgroup
  • contranormal subgroup
  • abnormal subgroup
  • self-normalizing subgroup

Related notions in algebra:

  • ideal (ring theory)

References

External links