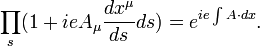Magnetic monopole
In physics, a magnetic monopole is a hypothetical particle that is a magnet with only one pole (see Maxwell's equations for more on magnetic poles). In more technical terms, it would have a net "magnetic charge". Modern interest in the concept stems from particle theories, notably Grand Unified Theories and superstring theories, which predict their existence.[1][2]
The classical theory of magnetic charge is as old as Maxwell's equations, but is considered much less important or interesting than the quantum theory of magnetic charge, which started with a 1931 paper by Paul Dirac.[3] In this paper, Dirac showed that if magnetic monopoles exist, then that would explain the quantization of electric charge in the universe. Since then, several systematic monopole searches have been performed. Experiments in 1975[4] and 1982[5] produced candidate events that were initially interpreted as monopoles, but these are now regarded to be inconclusive.[6] It therefore remains possible that monopoles do not exist at all.
Monopole detection is an open problem in experimental physics. Within theoretical physics, modern approaches agree that monopoles exist, in particular Grand Unified Theories and string theory both require them. Joseph Polchinski, a prominent string-theorist, described the existence of monopoles as "one of the safest bets that one can make about physics not yet seen".[7] These theories are not necessarily inconsistent with the experimental evidence: in some models magnetic monopoles are unlikely to be observed, because they are too massive to be created in particle accelerators, and too rare in the universe to wander into a particle detector.[7]
Contents |
Background
Magnets exert forces on one another, similar to electric charges. Like poles will repel each other, and unlike poles will attract. When a magnet (an object conventionally described as having magnetic north and south poles) is cut in half across the axis joining those "poles", the resulting pieces are two normal (albeit smaller) magnets. Each has its own north pole and south pole.
Even atoms have tiny magnetic fields. In the Bohr model of an atom, electrons orbit the nucleus. The constant change in their motion gives rise to a magnetic field. Permanent magnets have measurable magnetic fields because the atoms and molecules in them are arranged in a way that their individual magnetic fields align, combining to form large aggregate fields. In this model, the lack of a single pole makes intuitive sense; cutting a bar magnet in half does nothing to the arrangement of the molecules within. The end result is two magnetic bars whose atoms have the same orientation as before, and therefore generate a magnetic field with the same orientation as the original larger magnet.
Maxwell's equations
Maxwell's equations of electromagnetism relate the electric and magnetic fields to the motions of electric charges. The standard form of the equations provide for an electric charge, but posit no magnetic charge. Except for this, the equations are symmetric under interchange of electric and magnetic field.[8] In fact, symmetric equations can be written when all charges are zero, and this is how the wave equation is derived.
Fully symmetric equations can also be written if one allows for the possibility of "magnetic charges" analogous to electric charges.[9] With the inclusion of a variable for these magnetic charges, say 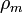 , there will also be a "magnetic current" variable in the equations,
, there will also be a "magnetic current" variable in the equations, 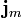 .
.
If magnetic charges do not exist, or if they exist but where they are not present in a region, then the new variables are zero, and the extended equations reduce to the conventional equations of electromagnetism such as  . Classically, the question is "Why does the magnetic charge always seem to be zero?"
. Classically, the question is "Why does the magnetic charge always seem to be zero?"
In cgs units
The extended Maxwell's equations are as follows, in cgs units:[10]
| Name | Without magnetic monopoles | With magnetic monopoles | |
|---|---|---|---|
| Gauss's law: | 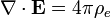 |
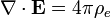 |
|
| Gauss' law for magnetism: |  |
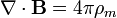 |
|
| Faraday's law of induction: |  |
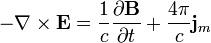 |
|
| Ampère's law (with Maxwell's extension): |
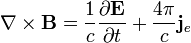 |
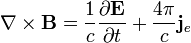 |
|
| Note: For the equations in nondimensionalized form, remove the factors of c. | |||
The Lorentz force becomes[10][11]
In SI units
In SI units, there are two conflicting conventions in use for magnetic charge. In one, magnetic charge has units of webers, while in the other, magnetic charge has units of ampere-meters. Maxwell's equations then take the following forms:[12]
| Name | Without magnetic monopoles | Weber convention | Ampere·meter convention |
|---|---|---|---|
| Gauss' Law |  |
 |
 |
| Gauss' Law for magnetism |  |
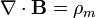 |
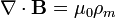 |
| Faraday's Law of induction |  |
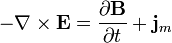 |
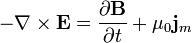 |
| Ampère's Law |  |
 |
 |
| Lorentz force | 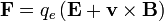 |
  |
  |
Dirac's quantization
One of the defining advances in quantum theory was Paul Dirac's work on developing a relativistic quantum electromagnetism. Before his formulation, the presence of electric charge was simply "inserted" into QM, but in 1931 Dirac showed that a discrete charge naturally "falls out" of QM.
Consider a system consisting of a single stationary electric monopole (an electron, say) and a single stationary magnetic monopole. Classically, the electromagnetic field surrounding them has a momentum density given by the Poynting vector, and it also has a total angular momentum, which is proportional to the product 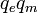 , and independent of the distance between them.
, and independent of the distance between them.
Quantum mechanics dictates, however, that angular momentum is quantized in units of ħ, and therefore the product 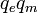 must also be quantized. This means that if even a single magnetic monopole existed in the universe, all electric charges would then be quantized.
must also be quantized. This means that if even a single magnetic monopole existed in the universe, all electric charges would then be quantized.
What are the units in which magnetic charge would be quantized? Although it would be possible simply to integrate over all space to find the total angular momentum in the above example, Dirac took a different approach, which led to new ideas. He considered a point-like magnetic charge whose magnetic field behaves as  and is directed in the radial direction. Because the divergence of
and is directed in the radial direction. Because the divergence of 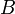 is equal to zero almost everywhere, except for the locus of the magnetic monopole at
is equal to zero almost everywhere, except for the locus of the magnetic monopole at  , one can locally define the vector potential such that the curl of the vector potential
, one can locally define the vector potential such that the curl of the vector potential 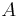 equals the magnetic field
equals the magnetic field 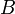 .
.
However, the vector potential cannot be defined globally precisely because the divergence of the magnetic field is proportional to the delta function at the origin. We must define one set of functions for the vector potential on the Northern hemisphere, and another set of functions for the Southern hemispheres. These two vector potentials are matched at the equator, and they differ by a gauge transformation. The wave function of an electrically charged particle (a probe) that orbits the equator generally changes by a phase, much like in the Aharonov-Bohm effect. This phase is proportional to the electric charge  of the probe, as well as to the magnetic charge
of the probe, as well as to the magnetic charge 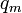 of the source. Dirac was originally considering an electron whose wave function is described by the Dirac equation.
of the source. Dirac was originally considering an electron whose wave function is described by the Dirac equation.
Because the electron returns to the same point after the full trip around the equator, the phase  of its wave function must be unchanged, which implies that the phase
of its wave function must be unchanged, which implies that the phase 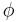 added to the wave function must be a multiple of
added to the wave function must be a multiple of  :
:
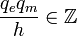 , where
, where  is the set of integers and h is Planck's constant. (SI units, weber convention)[13]
is the set of integers and h is Planck's constant. (SI units, weber convention)[13]
This is known as the Dirac quantization condition. The hypothetical existence of a magnetic monopole would imply that the electric charge must be quantized in certain units; also, the existence of the electric charges implies that the magnetic charges of the hypothetical magnetic monopoles, if they exist, must be quantized in units inversely proportional to the elementary electric charge.
At the time it was not clear if such a thing existed, or even had to. After all, another theory could come along that would explain charge quantization without need for the monopole. The concept remained something of a curiosity. However, in the time since the publication of this seminal work, no other widely accepted explanation of charge quantization has appeared. (The concept of local gauge invariance—see gauge theory below—provides a natural explanation of charge quantization, without invoking the need for magnetic monopoles; but only if the U(1) gauge group is compact, in which case we will have magnetic monopoles anyway.)
If we maximally extend the definition of the vector potential for the Southern hemisphere, it will be defined everywhere except for a semi-infinite line stretched from the origin in the direction towards the Northern pole. This semi-infinite line is called the Dirac string and its effect on the wave function is analogous to the effect of the solenoid in the Aharonov-Bohm effect. The quantization condition comes from the requirement that the phases around the Dirac string are trivial, which means that the Dirac string must be unphysical. The Dirac string is merely an artifact of the coordinate chart used and should not be taken seriously.
The Dirac monopole is a singular solution of Maxwell's equation (because it requires removing the worldline from spacetime); in more complicated theories, it is superseded by a smooth solution such as the 't Hooft-Polyakov monopole.
Topological interpretation
Dirac string
A gauge theory like electromagnetism is defined by a gauge field, which associates a group element to each path in space time. For infinitesimal paths, the group element is close to the identity, while for longer paths the group element is the successive product of the infinitesimal group elements along the way.
In electrodynamics, the group is U(1), unit complex numbers under multiplication. For infinitesimal paths, the group element is  which implies that for finite paths parametrized by s, the group element is:
which implies that for finite paths parametrized by s, the group element is:
The map from paths to group elements is called the Wilson loop or the holonomy, and for a U(1) gauge group it is the phase factor which the wavefunction of a charged particle acquires as it traverses the path. For a loop:
So that the phase a charged particle gets when going in a loop is the magnetic flux through the loop. When a small solenoid has a magnetic flux, there are interference fringes for charged particles which go around the solenoid, or around different sides of the solenoid, which reveal its presence.
But if all particle charges are integer multiples of e, solenoids with a flux of 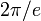 have no interference fringes, because the phase factor for any charged particle is
have no interference fringes, because the phase factor for any charged particle is  . Such a solenoid, if thin enough, is quantum mechanically invisible. If such a solenoid were to carry a flux of
. Such a solenoid, if thin enough, is quantum mechanically invisible. If such a solenoid were to carry a flux of 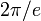 , when the flux leaked out from one of its ends it would be indistinguishable from a monopole.
, when the flux leaked out from one of its ends it would be indistinguishable from a monopole.
Dirac's monopole solution in fact describes an infinitesimal line solenoid ending at a point, and the location of the solenoid is the singular part of the solution, the Dirac string. Dirac strings link monopoles and antimonopoles of opposite magnetic charge, although in Dirac's version, the string just goes off to infinity. The string is unobservable, so you can put it anywhere, and by using two coordinate patches, the field in each patch can be made nonsingular by sliding the string to where it cannot be seen.
Grand unified theories
In a U(1) with quantized charge, the gauge group is a circle of radius 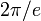 . Such a U(1) is called compact. Any U(1) which comes from a Grand Unified Theory is compact, because only compact higher gauge groups make sense. The size of the gauge group is a measure of the inverse coupling constant, so that in the limit of a large volume gauge group, the interaction of any fixed representation goes to zero.
. Such a U(1) is called compact. Any U(1) which comes from a Grand Unified Theory is compact, because only compact higher gauge groups make sense. The size of the gauge group is a measure of the inverse coupling constant, so that in the limit of a large volume gauge group, the interaction of any fixed representation goes to zero.
The U(1) case is special because all its irreducible representations are the same size--- the charge is bigger by an integer amount but the field is still just a complex number--- so that in U(1) gauge field theory it is possible to take the decompactified limit with no contradiction. The quantum of charge becomes small, but each charged particle has a huge number of charge quanta so its charge stays finite. In a non-compact U(1) gauge theory, the charges of particles are generically not integer multiples of a single unit. Since charge quantization is an experimental certainty, it is clear that the U(1) of electromagnetism is compact.
GUTs lead to compact U(1)s, so they explain charge quantization in a way that seems to be logically independent from magnetic monopoles. But the explanation is essentially the same, because in any GUT which breaks down to a U(1) at long distances, there are magnetic monopoles.
The argument is topological:
- The holonomy of a gauge field maps loops to elements of the gauge group. Infinitesimal loops are mapped to group elements infinitesimally close to the identity.
- If you imagine a big sphere in space, you can deform an infinitesimal loop which starts and ends at the north pole as follows: stretch out the loop over the western hemisphere until it becomes a great circle (which still starts and ends at the north pole) then let it shrink back to a little loop while going over the eastern hemisphere. This is called lassoing the sphere.
- Lassoing is a sequence of loops, so the holonomy maps it to a sequence of group elements, a continuous path in the gauge group. Since the loop at the beginning of the lassoing is the same as the loop at the end, the path in the group is closed.
- If the group path associated to the lassoing procedure winds around the U(1), the sphere contains magnetic charge. During the lassoing, the holonomy changes by the amount of magnetic flux through the sphere.
- Since the holonomy at the beginning and at the end is the identity, the total magnetic flux is quantized. The magnetic charge is proportional to the number of windings N, the magnetic flux through the sphere is equal to
 . This is the Dirac quantization condition, and it is a topological condition which demands that the long distance U(1) gauge field configurations are consistent.
. This is the Dirac quantization condition, and it is a topological condition which demands that the long distance U(1) gauge field configurations are consistent. - When the U(1) comes from breaking a compact Lie group, the path which winds around the U(1) enough times is topologically trivial in the big group. In a non-U(1) compact lie group, the covering space is a Lie group with the same Lie algebra but where all closed loops are contractible. Lie groups are homogenous, so that any cycle in the group can be moved around so that it starts at the identity, then its lift to the covering group ends at P, which is a lift of the identity. Going around the loop twice gets you to
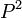 , three times to
, three times to 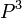 , all lifts of the identity. But there are only finitely many lifts of the identity, because the lifts can't accumulate. This number of times one has to traverse the loop to make it contractible is small, for example if the GUT group is SO(3), the covering group is SU(2), and going around any loop twice is enough.
, all lifts of the identity. But there are only finitely many lifts of the identity, because the lifts can't accumulate. This number of times one has to traverse the loop to make it contractible is small, for example if the GUT group is SO(3), the covering group is SU(2), and going around any loop twice is enough. - This means that there is a continuous gauge-field configuration in the GUT group allows the U(1) monopole configuration to unwind itself at short distances, at the cost of not staying in the U(1). In order to do this with as little energy as possible, you should only leave the U(1) in the neighborhood of one point, which is called the core of the monopole. Outside the core, the monopole has only magnetic field energy.
So the Dirac monopole is a topological defect in a compact U(1) gauge theory. When there is no GUT, the defect is a singularity--- the core shrinks to a point. But when there is some sort of short distance regulator on space time, the monopoles have a finite mass. Monopoles occur in lattice U(1), and there the core size is the lattice size. In general, they are expected to occur whenever there is a short-distance regulator.
String theory
In our universe, quantum gravity provides the regulator. When gravity is included, the monopole singularity can be a black hole, and for large magnetic charge and mass, the black hole mass is equal to the black hole charge, so that the mass of the magnetic black hole is not infinite. If the black hole can decay completely by Hawking radiation, as required by holography, the lightest charged particles can't be too heavy. The lightest monopole should have a mass less than or comparable to its charge in natural units.
So in a consistent holographic theory, and string theory is the only known example, there are always finite mass monopoles. For ordinary electromagnetism, the mass bound is not very useful because it is about the Planck mass.
Mathematical formulation
In mathematics, a gauge field is defined as a connection over a principal G-bundle over spacetime. G is the gauge group, and it acts on each fiber of the bundle separately.
A connection on a G bundle tells you how to glue F's together at nearby points of M. It starts with a continuous symmetry group G which acts on F, and then it associates a group element with each infinitesimal path. Group multiplication along any path tells you how to move from on point on the bundle to another, by acting the G element of a path on the fiber F.
In mathematics, the definition of bundle is designed to emphasize topology, so the notion of connection is added on as an afterthought. In physics, the connection is the fundamental physical object. Once you have a connection, there are nontrivial bundles which occur as connections of a trivial bundle. For example, the twisted torus is a connection on a U(1) bundle of a circle on a circle.
If space time has no topology, if it is R4 the space of all possible connections of the G-bundle is connected. But consider what happens when we remove a timelike worldline from spacetime. The resulting spacetime is homotopically equivalent to the topological sphere S2.
A principal G-bundle over S2 is defined by covering S2 by two charts, each homeomorphic to the open 2-ball such that their intersection is homeomorphic to the strip S1×I. 2-balls are homotopically trivial and the strip is homotopically equivalent to the circle S1. So a topological classification of the possible connections is reduced to classifying the transition functions. The transition function maps the strip to G, and the different ways of mapping a strip into G is given by the first homotopy group of G.
So in the G-bundle formulation, a gauge theory admits Dirac monopoles provided G is not simply connected, whenever there are paths that go around the group that cannot be deformed to nothing. U(1), which has quantized charges, is not simply connected and can have Dirac monopoles while R, its universal covering group, is simply connected, doesn't have quantized charges and does not admit Dirac monopoles. The mathematical definition is equivalent to the physics definition provided that, following Dirac, gauge fields are allowed which are only defined patch-wise and the gauge field on different patches are glued after a gauge transformation.
This argument for monopoles is a restatement of the lasso argument for a pure U(1) theory. It generalizes to d + 1 dimensions with 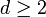 in several ways. One way is to extend everything into the extra dimensions, so that U(1) monopoles become sheets of dimension d-3. Another way is to examine the type of topological singularity at a point with the homotopy group πd−2(G).
in several ways. One way is to extend everything into the extra dimensions, so that U(1) monopoles become sheets of dimension d-3. Another way is to examine the type of topological singularity at a point with the homotopy group πd−2(G).
Grand unified theories
In more recent years, a new class of theories has also suggested the presence of a magnetic monopole.
In the early 1970s, the successes of quantum field theory and gauge theory in the development of electroweak and the strong nuclear force led many theorists to move on to attempt to combine them in a single theory known as a grand unified theory, or GUT. Several GUTs were proposed, most of which had the curious feature of suggesting the presence of a real magnetic monopole particle. More accurately, GUTs predicted a range of particles known as dyons, of which the most basic state is a monopole. The charge on magnetic monopoles predicted by GUTs is either 1 or 2gD, depending on the theory.
The majority of particles appearing in any quantum field theory are unstable, and decay into other particles in a variety of reactions that have to conserve various values. Stable particles are stable because there are no lighter particles to decay into that still conserve these values. For instance, the electron has a lepton number of 1 and an electric charge of 1, and there are no lighter particles that conserve these values. On the other hand, the muon, essentially a heavy electron, can decay into the electron and is therefore not stable.
The dyons in these same theories are also stable, but for an entirely different reason. The dyons are expected to exist as a side effect of the "freezing out" of the conditions of the early universe, or symmetry breaking. In this model the dyons arise due to the vacuum configuration in a particular area of the universe, according to the original Dirac theory. They remain stable not because of a conservation condition, but because there is no simpler topological state to which they can decay.
The length scale over which this special vacuum configuration exists is called the correlation length of the system. A correlation length cannot be larger than causality would allow, therefore the correlation length for making magnetic monopoles must be at least as big as the horizon size determined by the metric of the expanding universe. According to that logic, there should be at least one magnetic monopole per horizon volume as it was when the symmetry breaking took place. This leads to a direct prediction of the amount of monopoles in the universe today, which is about 1011 times the critical density of our universe. The universe appears to be close to critical density, so monopoles should be fairly common. For this reason, monopoles became a major interest in the 1970s and 80s, along with the other "approachable" prediction of GUTs, proton decay. The apparent problem with monopoles is resolved by cosmic inflation that greatly reduces the expected abundance of magnetic monopoles.
Many of the other particles predicted by these GUTs were beyond the abilities of current experiments to detect. For instance, a wide class of particles known as the X and Y bosons are predicted to mediate the coupling of the electroweak and strong forces, but these particles are extremely heavy and well beyond the capabilities of any reasonable particle accelerator to create.
Monopole searches
A number of attempts have been made to detect magnetic monopoles. One of the simplest is to use a loop of superconducting wire that can look for even tiny magnetic sources, a so-called "superconducting quantum interference device", or SQUID. Given the predicted density, loops small enough to fit on a lab bench would expect to see about one monopole event per year. Although there have been tantalizing events recorded, in particular the event recorded by Blas Cabrera on the night of February 14, 1982 (thus, sometimes referred to as the "Valentine's Day Monopole"), there has never been reproducible evidence for the existence of magnetic monopoles. The lack of such events places a limit on the number of monopoles of about 1 monopole per 1029 nucleons.
Another experiment in 1975 resulted in the announcement of the detection of a moving magnetic monopole in cosmic rays by the team of Price.[4] Price later retracted his claim, and a possible alternative explanation was offered by Alvarez[14]. In his paper it was demonstrated that the path of the cosmic ray event that was claimed to be due to a magnetic monopole could be reproduced by a path followed by a Platinum nucleus fragmenting to Osmium and then to Tantalum.
Other experiments rely on the strong coupling of monopoles with photons, as is the case for any electrically charged particle as well. In experiments involving photon exchange in particle accelerators, monopoles should be produced in reasonable numbers, and detected due to their effect on the scattering of the photons. The probability of a particle being created in such experiments is related to their mass — heavier particles are less likely to be created — so by examining such experiments limits on the mass can be calculated. The most recent such experiments suggest that monopoles with masses below 600 GeV/c² do not exist, while upper limits on their mass due to the existence of the universe (which would have collapsed by now if they were too heavy) are about 1017 GeV/c².
In popular culture
- In the turn-based strategy game Sid Meier's Alpha Centauri, monopole magnets are one of the researchable technologies. Once a player has developed this technology, that player is able to upgrade roads to magnetic tubes. Units moving along a magnetic tube are able to do so "instantly" (i.e., the movement does not count against the number of moves the unit may be moved each turn).
- In Larry Niven's Known Space monopoles are used widely, specifically in the propulsion systems of slower than light fusion ramjets and various interplanetary craft. They are found and harvested in asteroid belts by belters.
- In the Anime Outlaw Star Gene Starwind uses a magnetic monopole to escape from the prison colony of Hecatonchires. Having a pair of monopoles while over the magnetic north of a planet, he discards the south which slams hard into the ground, while the north monopole lifts him and another man up and away.
- In the video game Star Control II, magnetic monopoles are a valuable exotic material found on some planets.
- In the online science fiction world-building project Orion's Arm, magnetic monopoles are synthetically created particles that allow for the fabrication of exotic molecules, and are the basis of many advanced technologies.
- In the novel Omega Minor by Paul Verhaeghen, one of the key storylines in the book involves a Berlin student's attempts to detect a magnetic monopole, which she eventually manages with the help of a carefully planned nuclear explosion.
- In the video game Braid, one of the texts in the epilogue contains magnetic monopoles, naming them among other items desired by the protagonist.
See also
- Dirac string
- Dyon
- Felix Ehrenhaft
- Gauss' law for magnetism
- Halbach array
- Instanton
- Meron
- Soliton
- 't Hooft-Polyakov monopole
- Wu-Yang monopole
Notes
- ↑ Wen, Xiao-Gang;Witten, Edward, Electric and magnetic charges in superstring models,Nuclear Physics B, Volume 261, p. 651-677
- ↑ S. Coleman, The Magnetic Monopole 50 years Later, reprinted in Aspects of Symmetry
- ↑ Paul Dirac, "Quantised Singularities in the Electromagnetic Field". Proc. Roy. Soc. (London) A 133, 60 (1931). Free web link.
- ↑ 4.0 4.1 P. B. Price; E. K. Shirk; W. Z. Osborne; L. S. Pinsky (1975-08-25). "Evidence for Detection of a Moving Magnetic Monopole". Physical Review Letters (American Physical Society) 35 (8): 487–490. doi:.
- ↑ Blas Cabrera (1982-05-17). "First Results from a Superconductive Detector for Moving Magnetic Monopoles". Physical Review Letters (American Physical Society) 48 (20): 1378–1381. doi:.
- ↑ Milton p.60
- ↑ 7.0 7.1 Polchinski, arXiv 2003
- ↑ The fact that the electric and magnetic fields can be written in a symmetric way is specific to the fact that space is three-dimensional. When the equations of electromagnetism are extrapolated to other dimensions, the magnetic field is described as a rank 2 antisymmetric tensor, while the electric field remains a true vector. In dimensions other than 3, these two objects do not have the same number of components.
- ↑ http://www.ieee-virtual-museum.org/collection/tech.php?id=2345881&lid=1
- ↑ 10.0 10.1 F. Moulin (2001) (pdf). Magnetic monopoles and Lorentz force. 116B. pp. 869–877. http://arxiv.org/pdf/math-ph/0203043.
- ↑ Wolfgang Rindler (November 1989). "Relativity and electromagnetism: The force on a magnetic monopole". American Journal of Physics (American Journal of Physics) 57 (11): 993–994. doi:.
- ↑ For the convention where magnetic charge has units of webers, see Jackson 1999. In particular, for Maxwell's equations, see section 6.11, equation (6.150), page 273, and for the Lorentz force law, see page 290, exercise 6.17(a). For the convention where magnetic charge has units of ampere-meters, see (for example) arXiv:physics/0508099v1, eqn (4).
- ↑ Jackson 1999, section 6.11, equation (6.153), page 275
- ↑ Alvarez, Luis W. "Analysis of a Reported Magnetic Monopole" in International symposium on lepton and photon interactions at high energies, 21 Aug 1975. ed. Kirk, W. T. Proceedings of the 1975 international symposium on lepton and photon interactions at high energies: 967.
References
- Brau, Charles A. (2004). Modern Problems in Classical Electrodynamics. Oxford University Press. ISBN 0-19-514665-4.
- Jackson, John David (1999). Classical Electrodynamics (3rd ed. ed.). New York: Wiley. ISBN 0-471-30932-X.
- Milton, Kimball A. (June 2006). "Theoretical and experimental status of magnetic monopoles". Reports on Progress in Physics 69 (6): 1637–1711. doi:. http://arxiv.org/abs/hep-ex/0602040.
- Shnir, Yakov M. (2005). Magnetic Monopoles. Springer-Verlag. ISBN 3-540-25277-0.
External links
- Magnetic Monopole Searches (lecture notes)
- Particle Data Group summary of magnetic monopole search
- 'Race for the Pole' Dr David Milstead Freeview 'Snapshot' video by the Vega Science Trust and the BBC/OU.