Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" (after Sophus Lie, pronounced /ˈliː/ ("lee"), not /ˈlaɪ/ ("lie") ) was introduced by Hermann Weyl in the 1930s. In older texts, the name "infinitesimal group" is used.
Contents |
Definition and first properties
A Lie algebra is a type of algebra over a field; it is a vector space 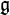 over some field F together with a binary operation [·, ·]
over some field F together with a binary operation [·, ·]
called the Lie bracket, which satisfies the following axioms:
- Bilinearity:
- for all scalars a, b in F and all elements x, y, z in

- Anticommutativity, or skew-symmetry:
- for all elements x, y in
 When F is a field of characteristic two, one has to impose the stronger condition:
When F is a field of characteristic two, one has to impose the stronger condition:
- for all x in

- The Jacobi identity:
- for all x, y, z in

For any associative algebra A with multiplication  , one can construct a Lie algebra L(A). As a vector space, L(A) is the same as A. The Lie bracket of two elements of L(A) is defined to be their commutator in A:
, one can construct a Lie algebra L(A). As a vector space, L(A) is the same as A. The Lie bracket of two elements of L(A) is defined to be their commutator in A:
The associativity of the multiplication * in A implies the Jacobi identity of the commutator in L(A). In particular, the associative algebra of n × n matrices over a field F gives rise to the general linear Lie algebra  The associative algebra A is called an enveloping algebra of the Lie algebra L(A). It is known that every Lie algebra can be embedded into one that arises from an associative algebra in this fashion. See universal enveloping algebra.
The associative algebra A is called an enveloping algebra of the Lie algebra L(A). It is known that every Lie algebra can be embedded into one that arises from an associative algebra in this fashion. See universal enveloping algebra.
Homomorphisms, subalgebras, and ideals
The Lie bracket is not an associative operation in general, meaning that [[x,y],z] need not equal [x,[y,z]]. Nonetheless, much of the terminology that was developed in the theory of associative rings or associative algebras is commonly applied to Lie algebras. A subspace 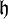 of a Lie algebra
of a Lie algebra  that is closed under the Lie bracket is called a Lie subalgebra. If a subspace
that is closed under the Lie bracket is called a Lie subalgebra. If a subspace 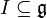 satisfies a stronger condition that
satisfies a stronger condition that
then I is called an ideal in the Lie algebra  [1] A Lie algebra in which the commutator is not identically zero and which has no proper ideals is called simple. A homomorphism between two Lie algebras (over the same ground field) is a linear map that is compatible with the commutators:
[1] A Lie algebra in which the commutator is not identically zero and which has no proper ideals is called simple. A homomorphism between two Lie algebras (over the same ground field) is a linear map that is compatible with the commutators:
for all elements x and y in  As in the theory of associative rings, ideals are precisely the kernels of homomorphisms, given a Lie algebra
As in the theory of associative rings, ideals are precisely the kernels of homomorphisms, given a Lie algebra  and an ideal I in it, one constructs the factor algebra
and an ideal I in it, one constructs the factor algebra 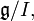 and the first isomorphism theorem holds for Lie algebras. Given two Lie algebras
and the first isomorphism theorem holds for Lie algebras. Given two Lie algebras  and
and 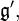 their direct sum is the vector space
their direct sum is the vector space 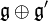 consisting of the pairs
consisting of the pairs 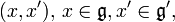 with the operation
with the operation
Examples
- Any vector space V endowed with the identically zero Lie bracket becomes a Lie algebra. Such Lie algebras are called abelian, cf. below. Any one-dimensional Lie algebra over a field is abelian, by the antisymmetry of the Lie bracket.
- The three-dimensional Euclidean space R3 with the Lie bracket given by the cross product of vectors becomes a three-dimensional Lie algebra.
- The Heisenberg algebra is a three-dimensional Lie algebra with generators:
-
 whose commutation relations are
whose commutation relations are![[x,y]=z,\quad [x,z]=0, \quad [y,z]=0.\,](/2009-wikipedia_en_wp1-0.7_2009-05/I/30d41804fdf41ad596190968f818f4d6.png)
- It is explicitly exhibited as the space of 3x3 strictly upper-triangular matrices.
- The subspace of the general linear Lie algebra
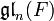 consisting of matrices of trace zero is a subalgebra[2], the special linear Lie algebra, denoted
consisting of matrices of trace zero is a subalgebra[2], the special linear Lie algebra, denoted 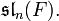
- Any Lie group G defines an associated real Lie algebra
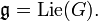 The definition in general is somewhat technical, but in the case of real matrix groups, it can be formulated via the exponential map, or the matrix exponent. The Lie algebra
The definition in general is somewhat technical, but in the case of real matrix groups, it can be formulated via the exponential map, or the matrix exponent. The Lie algebra  consists of those matrices X for which
consists of those matrices X for which
-
- for all real numbers t. The Lie bracket of
 is given by the commutator of matrices. As a concrete example, consider the special linear group SL(n,R), consisting of all n × n matrices with real entries and determinant 1. This is a matrix Lie group, and its Lie algebra consists of all n × n matrices with real entries and trace 0.
is given by the commutator of matrices. As a concrete example, consider the special linear group SL(n,R), consisting of all n × n matrices with real entries and determinant 1. This is a matrix Lie group, and its Lie algebra consists of all n × n matrices with real entries and trace 0.
- The real vector space of all n × n skew-hermitian matrices is closed under the commutator and forms a real Lie algebra denoted u(n). This is the Lie algebra of the unitary group U(n).
- An important class of infinite-dimensional real Lie algebras arises in differential topology. The space of smooth vector fields on a differentiable manifold M forms a Lie algebra, where the Lie bracket is defined to be the commutator of vector fields. One way of expressing the Lie bracket is through the formalism of Lie derivatives, which identifies a vector field X with a first order partial differential operator LX acting on smooth functions by letting LX(f) be the directional derivative of the function f in the direction of X. The Lie bracket [X,Y] of two vector fields is the vector field defined through its action on functions by the formula:
-
- This Lie algebra is related to the pseudogroup of diffeomorphisms of M.
- The commutation relations between the x, y, and z components of the angular momentum operator in quantum mechanics form a representation of a complex three-dimensional Lie algebra, which is the complexification of the Lie algebra so(3) of the three-dimensional rotation group:
- Kac–Moody algebra is an example of an infinite-dimensional Lie algebra.
Structure theory and classification
Every finite-dimensional real or complex Lie algebra has a faithful representation by matrices (Ado's theorem). Lie's fundamental theorems describe a relation between Lie groups and Lie algebras. In particular, any Lie group gives rise to a canonically determined Lie algebra, and conversely, for any Lie algebra there is a corresponding connected Lie group (Lie's third theorem). This Lie group is not determined uniquely, however, any two connected Lie groups with the same Lie algebra are locally isomorphic, and in particular, have the same universal cover. For instance, the special orthogonal group SO(3) and the special unitary group SU(2) give rise to the same Lie algebra, which is isomorphic to R3 with the cross-product, and SU(2) is a simply-connected twofold cover of SO(3). Real and complex Lie algebras can be classified to some extent, and this is often an important step toward the classification of Lie groups.
A Lie algebra  is abelian if the Lie bracket vanishes, i.e. [x,y] = 0, for all x and y in
is abelian if the Lie bracket vanishes, i.e. [x,y] = 0, for all x and y in  . Abelian Lie algebras correspond to commutative (or abelian) connected Lie groups. A more general class of Lie algebras is defined by the vanishing of all commutators of given length. A Lie algebra
. Abelian Lie algebras correspond to commutative (or abelian) connected Lie groups. A more general class of Lie algebras is defined by the vanishing of all commutators of given length. A Lie algebra  is nilpotent if the lower central series
is nilpotent if the lower central series
becomes zero eventually. By Engel's theorem, a Lie algebra is nilpotent if and only if for every u in  the adjoint endomorphism
the adjoint endomorphism
is nilpotent. More generally still, a Lie algebra  is said to be solvable if the derived series:
is said to be solvable if the derived series:
becomes zero eventually. Every Lie algebra has a unique maximal solvable ideal, called its radical. Under the Lie correspondence, nilpotent (respectively, solvable) connected Lie groups correspond to nilpotent (respectively, solvable) Lie algebras.
A Lie algebra is "simple" if it has no non-trivial ideals and is not abelian. A Lie algebra  is called semisimple if its radical is zero. Equivalently,
is called semisimple if its radical is zero. Equivalently,  is semisimple if it does not contain any non-zero abelian ideals. In particular, a simple Lie algebra is semisimple. Conversely, it can be proven that any semisimple Lie algebra is the direct sum of its minimal ideals, which are canonically determined simple Lie algebras.
is semisimple if it does not contain any non-zero abelian ideals. In particular, a simple Lie algebra is semisimple. Conversely, it can be proven that any semisimple Lie algebra is the direct sum of its minimal ideals, which are canonically determined simple Lie algebras.
In many ways, the classes of semisimple and solvable Lie algebras are at the opposite ends of the full spectrum of the Lie algebras. The Levi decomposition expresses an arbitrary Lie algebra as a semidirect product of its solvable radical and a semisimple Lie algebra, almost in a canonical way. Semisimple Lie algebras over an algebraically closed field have been completely classified through their root systems. The classification of solvable Lie algebras is a 'wild' problem, and cannot be accomplished in general.
Cartan's criterion gives conditions for a Lie agebra to be nilpotent, solvable, or semisimple. It is based on the notion of the Killing form, a symmetric bilinear form on  defined by the formula
defined by the formula
where tr denotes the trace of a linear operator. A Lie algebra  is semisimple if and only if the Killing form is nondegenerate. A Lie algebra
is semisimple if and only if the Killing form is nondegenerate. A Lie algebra  is solvable if and only if
is solvable if and only if ![K(\mathfrak{g},[\mathfrak{g},\mathfrak{g}])=0.](/2009-wikipedia_en_wp1-0.7_2009-05/I/dba47975afa1815f67b51312545dd9b9.png)
The concept of semisimplicity for Lie algebras is closely related with the complete reducibility of their representations. When the ground field F has characteristic zero, semisimplicity of a Lie algebra  over F is equivalent to the complete reducibility of all finite-dimensional representations of
over F is equivalent to the complete reducibility of all finite-dimensional representations of  An early proof of this statement proceeded via connection with compact groups (Weyl's unitary trick), but later entirely algebraic proofs were found.
An early proof of this statement proceeded via connection with compact groups (Weyl's unitary trick), but later entirely algebraic proofs were found.
Relation to Lie groups
Although Lie algebras are often studied in their own right, historically they arose as a means to study Lie groups. Given a Lie group, a Lie algebra can be associated to it either by endowing the tangent space to the identity with the differential of the adjoint map, or by considering the left-invariant vector fields as mentioned in the examples. This association is functorial, meaning that homomorphisms of Lie groups lift to homomorphisms of Lie algebras, and various properties are satisfied by this lifting: it commutes with composition, it maps Lie subgroups, kernels, quotients and cokernels of Lie groups to subalgebras, kernels, quotients and cokernels of Lie algebras, respectively.
The functor which takes each Lie group to its Lie algebra and each homomorphism to its differential is a full and faithful exact functor. This functor is not invertible; different Lie groups may have the same Lie algebra, for example SO(3) and SU(2) have isomorphic Lie algebras. Even worse, some Lie algebras need not have any associated Lie group. Nevertheless, when the Lie algebra is finite-dimensional, there is always at least one Lie group whose Lie algebra is the one under discussion, and a preferred Lie group can be chosen. Any finite-dimensional connected Lie group has a universal cover. This group can be constructed as the image of the Lie algebra under the exponential map. More generally, we have that the Lie algebra is homeomorphic to a neighborhood of the identity. But globally, if the Lie group is compact, the exponential will not be injective, and if the Lie group is not connected, simply connected or compact, the exponential map need not be surjective.
If the Lie algebra is infinite-dimensional, the issue is more subtle. In many instances, the exponential map is not even locally a homeomorphism (for example, in Diff(S1), one may find diffeomorphisms arbitrarily close to the identity which are not in the image of exp). Furthermore, some infinite-dimensional Lie algebras are not the Lie algebra of any group.
The correspondence between Lie algebras and Lie groups is used in several ways, including in the classification of Lie groups and the related matter of the representation theory of Lie groups. Every representation of a Lie algebra lifts uniquely to a representation of the corresponding connected, simply connected Lie group, and conversely every representation of any Lie group induces a representation of the group's Lie algebra; the representations are in one to one correspondence. Therefore, knowing the representations of a Lie algebra settles the question of representations of the group. As for classification, it can be shown that any connected Lie group with a given Lie algebra is isomorphic to the universal cover mod a discrete central subgroup. So classifying Lie groups becomes simply a matter of counting the discrete subgroups of the center, once the classification of Lie algebras is known (solved by Cartan et al. in the semisimple case).
Category theoretic definition
Using the language of category theory, a Lie algebra can be defined as an object A in Vec, the category of vector spaces together with a morphism [.,.]: A ⊗ A → A, where ⊗ refers to the monoidal product of Vec, such that
where τ (a ⊗ b) := b ⊗ a and σ is the cyclic permutation braiding (id ⊗ τA,A) ° (τA,A ⊗ id). In diagrammatic form:
See also
- Adjoint representation of a Lie algebra
- Anyonic Lie algebra
- Lie algebra cohomology
- Lie algebra representation
- Lie bialgebra
- Lie coalgebra
- Lie superalgebra
- Killing form
- Particle physics and representation theory
- Poisson algebra
- Quasi-Lie algebra
Notes
- ↑ Due to the anticommutativity of the commutator, the notions of a left and right ideal in a Lie algebra coincide.
- ↑ Humphreys p.2
References
- Hall, Brian C. Lie Groups, Lie Algebras, and Representations: An Elementary Introduction, Springer, 2003. ISBN 0-387-40122-9
- Erdmann, Karin & Wildon, Mark. Introduction to Lie Algebras, 1st edition, Springer, 2006. ISBN 1-84628-040-0
- Humphreys, James E. Introduction to Lie Algebras and Representation Theory, Second printing, revised. Graduate Texts in Mathematics, 9. Springer-Verlag, New York, 1978. ISBN 0-387-90053-5
- Jacobson, Nathan, Lie algebras, Republication of the 1962 original. Dover Publications, Inc., New York, 1979. ISBN 0-486-63832-4
- Kac, Victor G. et al. Course notes for MIT 18.745: Introduction to Lie Algebras, http://www-math.mit.edu/~lesha/745lec/
- O'Connor, J. J. & Robertson, E.F. Biography of Sophus Lie, MacTutor History of Mathematics Archive, http://www-history.mcs.st-and.ac.uk/Biographies/Lie.html
- O'Connor, J. J. & Robertson, E.F. Biography of Wilhelm Killing, MacTutor History of Mathematics Archive, http://www-history.mcs.st-and.ac.uk/Biographies/Killing.html
- Varadarajan, V. S. Lie Groups, Lie Algebras, and Their Representations, 1st edition, Springer, 2004. ISBN 0-387-90969-9
![[\cdot,\cdot]: \mathfrak{g}\times\mathfrak{g}\to\mathfrak{g}](/2009-wikipedia_en_wp1-0.7_2009-05/I/87109256baca5c0b5728fac15ed20220.png)
![[a x + b y, z] = a [x, z] + b [y, z], \quad [z, a x + b y] = a[z, x] + b [z, y]](/2009-wikipedia_en_wp1-0.7_2009-05/I/50d637f47c6fa9abec81c24abb5b7872.png)
![[x,y]=-[y,x]\,](/2009-wikipedia_en_wp1-0.7_2009-05/I/cedc8d491f577aef737493a4a33f11e5.png)
![[x,x]=0\](/2009-wikipedia_en_wp1-0.7_2009-05/I/6360181a3520eb081982d253748c13d5.png)
![[x,[y,z]] + [y,[z,x]] + [z,[x,y]] = 0 \quad](/2009-wikipedia_en_wp1-0.7_2009-05/I/69ee53d21fe7eb5e7b8ed7938cb8cab0.png)
![[a,b]=a * b-b * a.\](/2009-wikipedia_en_wp1-0.7_2009-05/I/75717148f9a2d7d818bdb857210f99c6.png)
![[\mathfrak{g},I]\subseteq I,](/2009-wikipedia_en_wp1-0.7_2009-05/I/cdb675dfcdf055542b0a2866da1668c2.png)
![f: \mathfrak{g}\to\mathfrak{g'}, \quad f([x,y])=[f(x),f(y)],](/2009-wikipedia_en_wp1-0.7_2009-05/I/84bc18de7a3e431be468c4afc3922e1e.png)
![[(x,x'),(y,y')]=([x,y],[x',y']), \quad x,y\in\mathfrak{g},\, x',y'\in\mathfrak{g'}.](/2009-wikipedia_en_wp1-0.7_2009-05/I/986d6d1ef97a01689ea2403c32e0a91e.png)
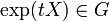
![L_{[X,Y]}f=L_X(L_Y f)-L_Y(L_X f).\,](/2009-wikipedia_en_wp1-0.7_2009-05/I/d42f8b71b6bdf21fd920bba2dcf7a362.png)
![[L_x, L_y] = i \hbar L_z](/2009-wikipedia_en_wp1-0.7_2009-05/I/47c348ae4c8c8658743df904f8454ba1.png)
![[L_y, L_z] = i \hbar L_x](/2009-wikipedia_en_wp1-0.7_2009-05/I/d3d71eb7b8e4ccb9f0fa62ffbe118dcd.png)
![[L_z, L_x] = i \hbar L_y](/2009-wikipedia_en_wp1-0.7_2009-05/I/e3a411fad7e976279abf8c5b7491846e.png)
![\mathfrak{g} > [\mathfrak{g},\mathfrak{g}] > [[\mathfrak{g},\mathfrak{g}],\mathfrak{g}] > [[[\mathfrak{g},\mathfrak{g}],\mathfrak{g}],\mathfrak{g}] > ...](/2009-wikipedia_en_wp1-0.7_2009-05/I/ac180ac5fc3b8eb705a241788116f435.png)
![ad(u):\mathfrak{g} \to \mathfrak{g}, \quad \operatorname{ad}(u)v=[u,v]](/2009-wikipedia_en_wp1-0.7_2009-05/I/fdec8f17522e05f697607e81c0173d46.png)
![\mathfrak{g} > [\mathfrak{g},\mathfrak{g}] > [[\mathfrak{g},\mathfrak{g}],[\mathfrak{g},\mathfrak{g}]] > [[[\mathfrak{g},\mathfrak{g}],[\mathfrak{g},\mathfrak{g}]],[[\mathfrak{g},\mathfrak{g}],[\mathfrak{g},\mathfrak{g}]]] > ...](/2009-wikipedia_en_wp1-0.7_2009-05/I/41bfbe4035909f3b782388839bfd7fc4.png)
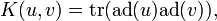
![[\cdot, \cdot] \circ (\mathrm{id} + \tau_{A,A}) = 0](/2009-wikipedia_en_wp1-0.7_2009-05/I/57b213e2a1e910a0c14c3ea6525f5c3b.png)
![[\cdot, \cdot] \circ ([\cdot, \cdot] \otimes \mathrm{id}) \circ (\mathrm{id} + \sigma + \sigma^2) = 0](/2009-wikipedia_en_wp1-0.7_2009-05/I/c1a17ab10d3f931e8faf7906bb348c25.png)
