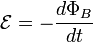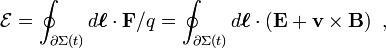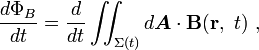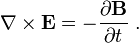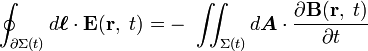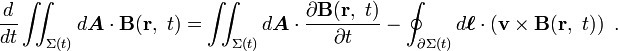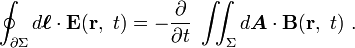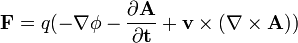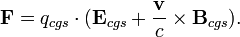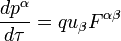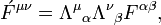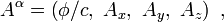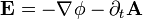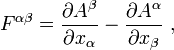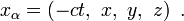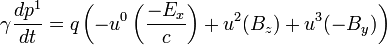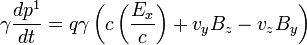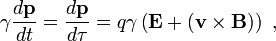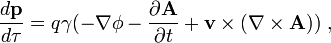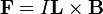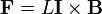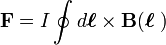Lorentz force
| Electromagnetism | ||||||||||||
 |
||||||||||||
Electricity · Magnetism
|
||||||||||||


In physics, the Lorentz force is the force on a point charge due to electromagnetic fields. It is given by the following equation in terms of the electric and magnetic fields:[1]
where
- F is the force (in newtons)
- E is the electric field (in volts per meter)
- B is the magnetic field (in teslas)
- q is the electric charge of the particle (in coulombs)
- v is the instantaneous velocity of the particle (in meters per second)
- × is the vector cross product
- ∇ and ∇ × are gradient and curl, respectively
or equivalently the following equation in terms of the vector potential and scalar potential:
where:
- A and ɸ are the magnetic vector potential and electrostatic potential, respectively, which are related to E and B by[2]
Note that these are vector equations: All the quantities written in boldface are vectors (in particular, F, E, v, B, A).
The Lorentz force law has a close relationship with Faraday's law of induction.
A positively charged particle will be accelerated in the same linear orientation as the E field, but will curve perpendicularly to both the instantaneous velocity vector v and the B field according to the right-hand rule (in detail, if the thumb of the right hand points along v and the index finger along B, then the middle finger points along F).
The term qE is called the electric force, while the term qv × B is called the magnetic force.[3] According to some definitions, the term "Lorentz force" refers specifically to the formula for the magnetic force:[4]
with the total electromagnetic force (including the electric force) given some other (nonstandard) name. This article will not follow this nomenclature: In what follows, the term "Lorentz force" will refer only to the expression for the total force.
The magnetic force component of the Lorentz force manifests itself as the force that acts on a current-carrying wire in a magnetic field. In that context, it is also called the Laplace force.
History
Hendrik Lorentz introduced this force in 1892.[5] However, the discovery of the Lorentz force was before Lorentz's time. In particular, it can be seen at equation (77) in Maxwell's 1861 paper On Physical Lines of Force. Later, Maxwell listed it as equation "D" of his 1864 paper, A Dynamical Theory of the Electromagnetic Field, as one of the eight original Maxwell's equations. In this paper the equation was written in a form which is equivalent to:
where
- A is the magnetic vector potential,
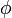 is the electrostatic potential,
is the electrostatic potential,- H is the magnetic field H,
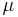 is magnetic permeability.
is magnetic permeability.
Although this equation is obviously a direct precursor of the modern Lorentz force equation, it actually differs in two respects:
- It does not contain any overt reference to electric charge. Maxwell refers to the quantity E as electromotive force. Its definition is somewhat unclear, but it would appear to correspond roughly to the modern day quantity, Electric Field, even though the word electromotive force is used nowadays for potential difference. He operated from Faraday's electro-tonic state A,[6] which he considered to be a momentum in his vortex sea. The closest term that we can trace to electric charge in Maxwell's papers is the density of free electricity, which appears to refer to the density of the aethereal medium of his molecular vortices and that gives rise to the momentum A. Maxwell believed that A was a fundamental quantity from which electromotive force can be derived.[7]
- The equation here contains the information that what we nowadays call E, which today can be expressed in terms of scalar and vector potentials according to
-
- The fact that E can be expressed this way is equivalent to one of the four modern Maxwell's equations, the Maxwell-Faraday equation.[8]
Despite its historical origins in the original set of eight Maxwell's equations, the Lorentz force is no longer considered to be one of "Maxwell's equations" as the term is currently used (that is, as reformulated by Heaviside). It now sits adjacent to Maxwell's equations as a separate and essential law.[1]
Maxwell had another equation (equation (5) in his 1861 paper) which was broadly similar in format to equation (77). Equation (5) was used to account for the forces which arise in the magnetic field, whereas equation (77) had an emphasis on electromagnetic induction. Equation (5) differed from equation (77) in the important respect that it had an additional centrifugal force term.
Significance of the Lorentz force
While the modern Maxwell's equations describe how electrically charged particles and objects give rise to electric and magnetic fields, the Lorentz force law completes that picture by describing the force acting on a moving point charge q in the presence of electromagnetic fields.[1][9] The Lorentz force law describes the effect of E and B upon a point charge, but such electromagnetic forces are not the entire picture. Charged particles are possibly coupled to other forces, notably gravity and nuclear forces. Thus, Maxwell's equations do not stand separate from other physical laws, but are coupled to them via the charge and current densities. The response of a point charge to the Lorentz law is one aspect; the generation of E and B by currents and charges is another.
In real materials the Lorentz force is inadequate to describe the behavior of charged particles, both in principle and as a matter of computation. The charged particles in a material medium both respond to the E and B fields and generate these fields. Complex transport equations must be solved to determine the time and spatial response of charges, for example, the Boltzmann equation or the Fokker–Planck equation or the Navier-Stokes equations. For example, see magnetohydrodynamics, fluid dynamics, electrohydrodynamics, superconductivity, stellar evolution. An entire physical apparatus for dealing with these matters has developed. See for example, Green–Kubo relations and Green's function (many-body theory).
Although one might suggest that these theories are only approximations intended to deal with large ensembles of "point particles", perhaps a deeper perspective is that the charge-bearing particles may respond to forces like gravity, or nuclear forces, or boundary conditions (see for example: boundary layer, boundary condition, Casimir effect, cross section (physics)) that are not electromagnetic interactions, or are approximated in a deus ex machina fashion for tractability.[10]
Lorentz force law as the definition of E and B
In many textbook treatments of classical electromagnetism, the Lorentz Force Law is used as the definition of the electric and magnetic fields E and B.[11] To be specific, the Lorentz Force is understood to be the following empirical statement:
- The electromagnetic force on a test charge at a given point and time is a certain function of its charge and velocity, which can be parameterized by exactly two vectors E and B, in the functional form:
If this empirical statement is valid (and, of course, countless experiments have shown that it is), then two vector fields E and B are thereby defined throughout space and time, and these are called the "electric field" and "magnetic field".
Note that the fields are defined everywhere in space and time, regardless of whether or not a charge is present to experience the force. In particular, the fields are defined with respect to what force a test charge would feel, if it were hypothetically placed there.
Note also that as a definition of E and B, the Lorentz force is only a definition in principle because a real particle (as opposed to the hypothetical "test charge" of infinitesimally-small mass and charge) would generate its own finite E and B fields, which would alter the electromagnetic force that it experiences. In addition, if the charge experiences acceleration, for example, if forced into a curved trajectory by some external agency, it emits radiation that causes braking of its motion. See, for example, Bremsstrahlung and synchrotron light. These effects occur through both a direct effect (called the radiation reaction force) and indirectly (by affecting the motion of nearby charges and currents).
Moreover, the electromagnetic force is not in general the same as the net force, due to gravity, electroweak and other forces, and any extra forces would have to be taken into account in a real measurement.
Lorentz force and Faraday's law of induction
Given a loop of wire in a magnetic field, Faraday's law of induction states:
where:
 is the magnetic flux through the loop,
is the magnetic flux through the loop,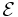 is the electromotive force (EMF) experienced,
is the electromotive force (EMF) experienced,- t is time
- The sign of the EMF is determined by Lenz's Law.
Using the Lorentz force law, the EMF around a closed path ∂Σ is given by:[12][13]
where dℓ is an element of the curve ∂Σ(t), imagined to be moving in time. The flux ΦB in Faraday's law of induction can be expressed explicitly as:
where
- Σ(t) is a surface bounded by the closed contour ∂Σ(t)
- E is the electric field,
- dℓ is an infinitesimal vector element of the contour ∂Σ,
- v is the velocity of the infinitesimal contour element dℓ,
- B is the magnetic field.
- dA is an infinitesimal vector element of surface Σ , whose magnitude is the area of an infinitesimal patch of surface, and whose direction is orthogonal to that surface patch.
- Both dℓ and dA have a sign ambiguity; to get the correct sign, the right-hand rule is used, as explained in the article Kelvin-Stokes theorem.
The surface integral at the right-hand side of this equation is the explicit expression for the magnetic flux ΦB through Σ. Thus, incorporating the Lorentz law in Faraday's equation, we find:[14] [15]
Notice that the ordinary time derivative appearing before the integral sign implies that time differentiation must include differentiation of the limits of integration, which vary with time whenever Σ(t) is a moving surface.
The above result can be compared with the version of Faraday's law of induction that appears in the modern Maxwell's equations, called here the Maxwell-Faraday equation:
The Maxwell-Faraday equation also can be written in an integral form using the Kelvin-Stokes theorem:[16]
Comparison of the Faraday flux law with the integral form of the Maxwell-Faraday relation suggests:
which is a form of the Leibniz integral rule valid because div B = 0.[17] The term in v × B accounts for motional EMF, that is the movement of the surface Σ, at least in the case of a rigidly translating body. In contrast, the integral form of the Maxwell-Faraday equation includes only the effect of the E-field generated by ∂B/∂t.
Often the integral form of the Maxwell-Faraday equation is used alone, and is written with the partial derivative outside the integral sign as:
Notice that the limits ∂Σ and Σ have no time dependence. In the context of the Maxwell-Faraday equation, the usual interpretation of the partial time derivative is extended to imply a stationary boundary. On the other hand, Faraday's law of induction holds whether the loop of wire is rigid and stationary, or in motion or in process of deformation, and it holds whether the magnetic field is constant in time or changing. However, there are cases where Faraday's law is either inadequate or difficult to use, and application of the underlying Lorentz force law is necessary. See inapplicability of Faraday's law.
If the magnetic field is fixed in time and the conducting loop moves through the field, the flux magnetic flux ΦB linking the loop can change in several ways. For example, if the B-field varies with position, and the loop moves to a location with different B-field, ΦB will change. Alternatively, if the loop changes orientation with respect to the B-field, the B•dA differential element will change because of the different angle between B and dA, also changing ΦB. As a third example, if a portion of the circuit is swept through a uniform, time-independent B-field, and another portion of the circuit is held stationary, the flux linking the entire closed circuit can change due to the shift in relative position of the circuit's component parts with time (surface Σ(t) time-dependent). In all three cases, Faraday's law of induction then predicts the EMF generated by the change in ΦB.
In a contrasting circumstance, when the loop is stationary and the B-field varies with time, the Maxwell-Faraday equation shows a nonconservative[18] E-field is generated in the loop, which drives the carriers around the wire via the q E term in the Lorentz force. This situation also changes ΦB, producing an EMF predicted by Faraday's law of induction.
Naturally, in both cases, the precise value of current that flows in response to the Lorentz force depends on the conductivity of the loop.
Lorentz force in terms of potentials
If the scalar potential and vector potential replace E and B (see Helmholtz decomposition), the force becomes:
or, equivalently (making use of the fact that v is a constant; see triple product),
where
- A is the magnetic vector potential
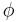 is the electrostatic potential
is the electrostatic potential- The symbols
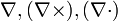 denote gradient, curl, and divergence, respectively.
denote gradient, curl, and divergence, respectively.
The potentials are related to E and B by
Lorentz force in cgs units
The above-mentioned formulae use SI units which are the most common among experimentalists, technicians, and engineers. In cgs units, which are somewhat more common among theoretical physicists, one has instead
where c is the speed of light. Although this equation looks slightly different, it is completely equivalent, since one has the following relations:
 ,
, 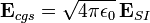 , and
, and 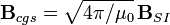
where ε0 and μ0 are the vacuum permittivity and vacuum permeability, respectively. In practice, unfortunately, the subscripts "cgs" and "SI" are always omitted, and the unit system has to be assessed from context.
Covariant form of the Lorentz force
Newton's law of motion can be written in covariant form in terms of the field strength tensor.
-
- where
 is c times the proper time of the particle,
is c times the proper time of the particle,- q is the charge,
- u is the 4-velocity of the particle, defined as:
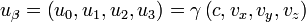
- with γ = Lorentz factor defined above, and F is the field strength tensor (or electromagnetic tensor) and is written in terms of fields as:
-
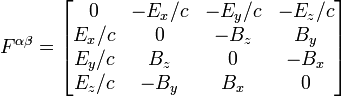 .
.
The fields are transformed to a frame moving with constant relative velocity by:
where 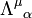 is a Lorentz transformation. Alternatively, using the four vector:
is a Lorentz transformation. Alternatively, using the four vector:
related to the electric and magnetic fields by:
the field tensor becomes:[19]
where:
Translation to vector notation
The  component (x-component) of the force is
component (x-component) of the force is
Here,  is the proper time of the particle. Substituting the components of the electromagnetic tensor F yields
is the proper time of the particle. Substituting the components of the electromagnetic tensor F yields
Writing the four-velocity in terms of the ordinary velocity yields
The calculation of the  or
or 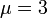 is similar yielding
is similar yielding
or, in terms of the vector and scalar potentials A and φ,
which are the relativistic forms of Newton's law of motion when the Lorentz force is the only force present.
Force on a current-carrying wire
When a wire carrying an electrical current is placed in a magnetic field, each of the moving charges, which comprise the current, experiences the Lorentz force, and together they can create a macroscopic force on the wire (sometimes called the Laplace force). By combining the Lorentz force law above with the definition of electrical current, the following equation results, in the case of a straight, stationary wire:
where
- F = Force, measured in newtons
- I = current in wire, measured in amperes
- B = magnetic field vector, measured in teslas
 = vector cross product
= vector cross product- L = a vector, whose magnitude is the length of wire (measured in metres), and whose direction is along the wire, aligned with the direction of conventional current flow.
Alternatively, some authors write
where the vector direction is now associated with the current variable, instead of the length variable. The two forms are equivalent.
If the wire is not straight but curved, the force on it can be computed by applying this formula to each infinitesimal segment of wire dℓ, then adding up all these forces via integration. Formally, the net force on a stationary, rigid wire carrying a current I is
(This is the net force. In addition, there will usually be torque, plus other effects if the wire is not perfectly rigid.)
One application of this is Ampère's force law, which describes how two current-carrying wires can attract or repel each other, since each experiences a Lorentz force from the other's magnetic field. For more information, see the article: Ampère's force law.
EMF
The magnetic force (q v × B) component of the Lorentz force is responsible for motional electromotive force (or motional EMF), the phenomenon underlying many electrical generators. When a conductor is moved through a magnetic field, the magnetic force tries to push electrons through the wire, and this creates the EMF. The term "motional EMF" is applied to this phenomenon, since the EMF is due to the motion of the wire.
In other electrical generators, the magnets move, while the conductors do not. In this case, the EMF is due to the electric force (qE) term in the Lorentz Force equation. The electric field in question is created by the changing magnetic field, resulting in an induced EMF, as described by the Maxwell-Faraday equation (one of the four modern Maxwell's equations).[20]
The two effects are not however symmetric. As one demonstration of this, a charge rotating around the magnetic axis of a stationary, cylindrically-symmetric bar magnet will experience a magnetic force, whereas if the charge is stationary and the magnet is rotating about its axis, there will be no force. This asymmetric effect is called Faraday's paradox.
Both of these EMF's, despite their different origins, can be described by the same equation, namely, the EMF is the rate of change of magnetic flux through the wire. (This is Faraday's law of induction, see above.) Einstein's theory of special relativity was partially motivated by the desire to better understand this link between the two effects.[20] In fact, the electric and magnetic fields are different faces of the same electromagnetic field, and in moving from one inertial frame to another, the solenoidal vector field portion of the E-field can change in whole or in part to a B-field or vice versa.[21]
General references
The numbered references refer in part to the list immediately below.
- Feynman, Richard Phillips; Leighton, Robert B.; Sands, Matthew L. (2006), The Feynman lectures on physics (3 vol.), Pearson / Addison-Wesley, ISBN 0-8053-9047-2: volume 2.
- Griffiths, David J. (1999), Introduction to electrodynamics (3rd ed.), Upper Saddle River, [NJ.]: Prentice-Hall, ISBN 0-13-805326-X
- Jackson, John David (1999), Classical electrodynamics (3rd ed.), New York, [NY.]: Wiley, ISBN 0-471-30932-X
- Serway, Raymond A.; Jewett, John W., Jr. (2004), Physics for scientists and engineers, with modern physics, Belmont, [CA.]: Thomson Brooks/Cole, ISBN 0-534-40846-X
- Srednicki, Mark A. (2007), Quantum field theory, Cambridge, [England] ; New York [NY.]: Cambridge University Press, ISBN 978-0-521-86449-7, http://books.google.com/books?id=5OepxIG42B4C&pg=PA315&dq=isbn:9780521864497&sig=zw7sVtby1W1S3nytX9NC0rwDN_0#PPA339,M1
Numbered footnotes and references
- ↑ 1.0 1.1 1.2 See Jackson page 2. The book lists the four modern Maxwell's equations, and then states, "Also essential for consideration of charged particle motion is the Lorentz force equation, F = q ( E+ v × B ), which gives the force acting on a point charge q in the presence of electromagnetic fields."
- ↑ These definitions use Helmholtz's theorem. Because divB = 0 (Gauss's law for magnetism), Helmholtz's theorem proves that we can define a vector field A (called the magnetic potential) such that B = ∇ × A. From the Maxwell-Faraday equation, ∇ × E = −∂t B so ∇ × [ E + ∂t A ] = 0. Applying Helmholtz's theorem again to E + ∂t A, which has zero curl, we find that we can define a scalar field ɸ (called the electric potential) with E + ∂t A = −∇ɸ. The equation for B automatically satisfies ∇•B = 0, that is, demonstrates that B is a solenoidal vector field. Also, the equation for E shows that it can have two different components: a conservative or irrotational vector field component (which originates in electric charges) and a nonconservative or curl component (which originates in the Maxwell-Faraday equation). For more details, see magnetic potential and electric potential.
- ↑ See Griffiths page 204.
- ↑ For example, see the website of the "Lorentz Institute": [1], or Griffiths.
- ↑ Darrigol, Olivier (2000), Electrodynamics from Ampère to Einstein, Oxford, [England]: Oxford University Press, p. 327, ISBN 0-198-50593-0, http://books.google.com/books?id=ysMf2pAid94C&printsec=frontcover&dq=Electrodynamics&lr=&as_brr=0&sig=RuQLjK14uJPu7mYCC0vRr0SdQFw#PPA327,M1
- ↑ "While the wire is subject to either volta-electric or magneto-electric induction it appears to be in a peculiar state, for it resists the formation of an electrical current in it. … I have … ventured to designate it as the electro-tonic state." Quoted by Maxwell from Faraday, Trans. Cam. Phil. Soc., p. 51, v. 10 (1864)
- ↑ At the experimental level in classical electromagnetism, E and B are the fundamental, measurable, physical fields. See, for example, Griffiths page 417, or Jackson page 239. However, in quantum field theory, the potentials A and
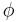 play a fundamental role. See, for example, Srednicki, Chapter 58, p. 351 ff. and R Littlejohn on quantization of the electromagnetic field; Physics 221B notes–quantizationPhysics 221B notes–interaction However, the fields themselves can be related to electromotive force (in the modern definition) only by addition of the Lorentz force. Maxwell did not formulate the equations with a separate Lorentz force equation.
play a fundamental role. See, for example, Srednicki, Chapter 58, p. 351 ff. and R Littlejohn on quantization of the electromagnetic field; Physics 221B notes–quantizationPhysics 221B notes–interaction However, the fields themselves can be related to electromotive force (in the modern definition) only by addition of the Lorentz force. Maxwell did not formulate the equations with a separate Lorentz force equation. - ↑ See Griffiths page 417, or Jackson page 239.
- ↑ See Griffiths page 326, which states that Maxwell's equations, "together with the [Lorentz] force law...summarize the entire theoretical content of classical electrodynamics".
- ↑ That is, a first-principles approach might be approximated to make calculation possible without complications that are not very significant to the results. For example, a metallic boundary might be approximated as having infinite conductivity. A statistical mechanical model of a plasma might approximate the treatment of collisions with boundaries and between particles.
- ↑ See, for example, Jackson p777-8.
- ↑ Landau, L. D., Lifshit︠s︡, E. M., & Pitaevskiĭ, L. P. (1984). Electrodynamics of continuous media; Volume 8 Course of theoretical physics (Second Edition ed.). Oxford: Butterworth-Heinemann. p. §63 (§49 pp. 205-207 in 1960 edition). ISBN 0750626348. http://worldcat.org/search?q=0750626348&qt=owc_search.
- ↑ M N O Sadiku (2007). Elements of elctromagnetics (Fourth Edition ed.). NY/Oxford: Oxford University Press. p. 391. ISBN 0-19-530048-3. http://books.google.com/books?id=w2ITHQAACAAJ&dq=isbn:0-19-530048-3&lr=&as_brr=0.
- ↑ If the boundary deforms, so velocity varies with location, the velocity v is the velocity at the location of dℓ. See Rothwell Edward J Rothwell, Michael J Cloud (2001). Electromagnetics. Boca Raton, Fla: CRC Press. p. 56. ISBN 084931397X. http://books.google.com/books?id=jCqv1UygjA4C&pg=PA55&dq=integration+%22moving+surface%22&lr=&as_brr=0&sig=z9OedzaAidQvKNKsApICeZny4fg#PPA56,M1.
- ↑ Jackson JD. Eqs. 5.141 & 5.142, p. 211. ISBN 0-471-30932-X. http://worldcat.org/search?q=047130932X&qt=owc_search.
- ↑ Roger F Harrington (2003). Introduction to electromagnetic engineering. Mineola, NY: Dover Publications. p. 56. ISBN 0486432416. http://books.google.com/books?id=ZlC2EV8zvX8C&pg=PA57&dq=%22faraday%27s+law+of+induction%22&lr=&as_brr=0&sig=P83DCljMC-sA7Pk2gBJfJ0Fp1yQ#PPA56,M1.
- ↑ If the surface deforms, the Leibniz integral rule is more complicated. A mathematical demonstration of this result for deformable surfaces has not been located.
- ↑ That is, a field that is not conservative, not expressible as the gradient of a scalar field, and not subject to the gradient theorem.
- ↑ DJ Griffiths (1999). Introduction to electrodynamics. Saddle River NJ: Pearson/Addison-Wesley. p. 541. ISBN 0-13-805326-X.
- ↑ 20.0 20.1 See Griffiths pages 301–3.
- ↑ Tai L. Chow (2006). Electromagnetic theory. Sudbury MA: Jones and Bartlett. p. 395. ISBN 0-7637-3827-1. http://books.google.com/books?id=dpnpMhw1zo8C&pg=PA153&dq=isbn=0763738271&sig=PgEEBA6TQEZ5fD_AhJQ8dd7MGHo#PPR6,M1.
Applications
The Lorentz force occurs in many devices, including:
- Cyclotrons and other circular path particle accelerators
- Mass spectrometers
- Velocity Filters
- Magnetrons
In its manifestation as the Laplace force on an electric current in a conductor, this force occurs in many devices including:
|
|
See also
|
|
|
External links
- Interactive Java tutorial on the Lorentz force National High Magnetic Field Laboratory
- Lorentz force (animation)
- Lorentz force (demonstration)
- Faraday's law: Tankersley and Mosca
- Notes from Physics and Astronomy HyperPhysics at Georgia State University; see also home page
![\mathbf{F} = q [\mathbf{E} + (\mathbf{v} \times \mathbf{B})],](/2009-wikipedia_en_wp1-0.7_2009-05/I/53a6eadab619ab497d9ea53061fd6ec7.png)
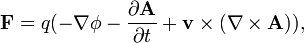
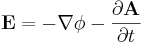


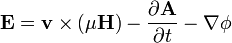
![\mathbf{F}=q[\mathbf{E}+(\mathbf{v}\times\mathbf{B})].](/2009-wikipedia_en_wp1-0.7_2009-05/I/97df3474bc68a2c0cf632c3653a6d8bd.png)