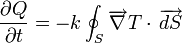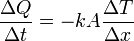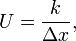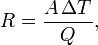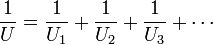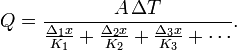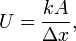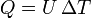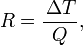Heat conduction
Heat conduction or thermal conduction is the spontaneous transfer of thermal energy through matter, from a region of higher temperature to a region of lower temperature, and acts to equalize temperature differences. It is also described as heat energy transferred from one material to another by direct contact.
Thermal energy, in the form of continuous random motion of the particles of the matter, is transferred by the same coulomb forces that act to support the structure of matter, so can be said to move by physical contact between the particles.
Heat can also be transferred by radiation and/or convection, and often more than one of these processes occur in a given situation.
Contents |
Fourier's law
The law of Heat Conduction, also know as Fourier's law, states that the time rate of heat transfer through a material is proportional to the negative gradient in the temperature and to the area at right angles, to that gradient, through which the heat is flowing. We can state this law in two equivalent forms: the integral form, in which we look at the amount of energy flowing into or out of a body as a whole, and the differential form, in which we look at the flows or fluxes of energy locally. Electrons in a metal transfer the heat from one particle to another further away.
Differential form
In the differential formulation of Fourier's law, the fundamental quantity is the local heat flux 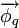 . This is the amount of energy flowing through an infinitesimal oriented surface per unit of time. The length of
. This is the amount of energy flowing through an infinitesimal oriented surface per unit of time. The length of  is given by the amount of energy flux per unit of time and the direction is given by the vector perpendicular to the surface. As a vector equation this leads to
is given by the amount of energy flux per unit of time and the direction is given by the vector perpendicular to the surface. As a vector equation this leads to
where (including the SI units)
 is the local heat flux, [W·m−2]
is the local heat flux, [W·m−2]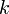 is the material's conductivity, [W·m−1·K−1],
is the material's conductivity, [W·m−1·K−1], is the temperature gradient, [K·m−1].
is the temperature gradient, [K·m−1].
Note that the thermal conductivity of a material generally varies with temperature, but the variation can be small over a significant range of temperatures for some common materials. In anisotropic materials the thermal conductivity typically varies with direction, in this case 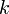 is a tensor.
is a tensor.
Integral form
By integrating the differential form over the material's total surface 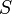 , we arrive at the integral form of Fourier's law:
, we arrive at the integral form of Fourier's law:
where (including the SI units)
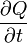 is the amount of heat transferred per unit time, <nowikiyellow>,
is the amount of heat transferred per unit time, <nowikiyellow>,
The above differential equation, when integrated for a simple exponential situation (see diagram), where uniform temperature across equally sized end surfaces and perfectly insulated sides exist, gives the heat flow rate between the end surfaces as:
where
- A is the cross-sectional surface area,
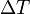 is the temperature difference between the ends,
is the temperature difference between the ends,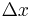 is the distance between the ends.
is the distance between the ends.
This law forms of the basis for the derivation of the heat equation. R-value is the unit for heat resistance, the reciprocal of the conductance. Ohm's law is the electrical analogue of Fourier's law.
Conductance
Writing
where U is the conductance, in W/(m2 K).
Fourier's law can also be stated as:
The reciprocal of conductance is resistance, R, given by:
and it is resistance which is additive when several conducting layers lie between the hot and cool regions, because A and Q are the same for all layers. In a multilayer partition, the total conductance is related to the conductance of its layers by:
So, when dealing with a multilayer partition, the following formula is usually used:
When heat is being conducted from one fluid to another through a barrier, it is sometimes important to consider the conductance of the thin film of fluid which remains stationary next to the barrier. This thin film of fluid is difficult to quantify, its characteristics depending upon complex conditions of turbulence and viscosity, but when dealing with thin high-conductance barriers it can sometimes be quite significant.
Intensive-property representation
The previous conductance equations written in terms of extensive properties, can be reformulated in terms of intensive properties.
Ideally, the formulae for conductance should produce a quantity with dimensions independent of distance, like Ohm's Law for electrical resistance: 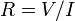 , and conductance:
, and conductance: 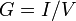 .
.
From the electrical formula:  , where ρ is resistivity, x = length, A cross sectional area, we have
, where ρ is resistivity, x = length, A cross sectional area, we have 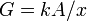 , where G is conductance, k is conductivity, x = length, A cross sectional area.
, where G is conductance, k is conductivity, x = length, A cross sectional area.
For Heat,
where U is the conductance.
Fourier's law can also be stated as:
analogous to Ohm's law: 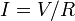 or
or 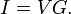
The reciprocal of conductance is resistance, R, given by:
analogous to Ohm's law: 
The sum of conductances in series is still correct.
See also
- Heat
- Thermal conductivity
- Heat flux
- Heat transfer
- Convection
- Convection diffusion equation
- Thermal radiation
- Thermal contact conductance
- Heat pipe
- Fick's law of diffusion
- Churchill-Bernstein Equation
External links
- Newton's Law of Cooling by Jeff Bryant based on a program by Stephen Wolfram, Wolfram Demonstrations Project.
- Sample Heaters
supercalifragilisticexpealidocious!