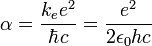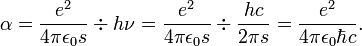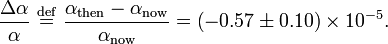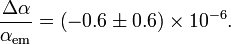Fine-structure constant

The fine-structure constant or Sommerfeld fine-structure constant, usually denoted 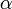\alpha \,, is the fundamental physical constant characterizing the strength of the electromagnetic interaction. It is a dimensionless quantity, and thus its numerical value is independent of the system of units used.

The best value currently is:[1]

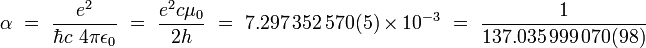\alpha\ =\ \frac{e^2}{\hbar c \ 4 \pi \epsilon_0}\ =\ \frac{e^2 c \mu_0}{2 h}\ =\ 7.297\,352\,570(5) \times 10^{-3}\ =\ \frac{1}{137.035\,999\,070(98)} .

(numbers within parentheses are uncertainties), where e is the elementary charge, ħ = h/(2π) is the reduced Planck constant, c is the speed of light in a vacuum, ε0 is the vacuum permittivity, and μ0 is the magnetic constant or vacuum permeability, a defined conversion factor.

The defining expression and the value recommended by 2006 CODATA as reported by NIST reference on constants, units, and uncertainty is:

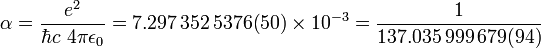\alpha = \frac{e^2}{\hbar c \ 4 \pi \epsilon_0} = 7.297\,352\,5376(50) \times 10^{-3} = \frac{1}{137.035\,999\,679(94)} .

However, after completion of the 2006 CODATA adjustment an error was discovered in one of the input data, leading to the first value given above.[1]

The name of the fine-structure constant refers to its earliest use in the theory for the fine structure of atomic energy spectra. However, its modern use is far from being as specialized as its name suggests.

Contents

Related definitions

The fine-structure constant can also be defined as

\alpha = \frac{k_e e^2}{\hbar c} = \frac{e^2}{2 \epsilon_0 h c}

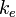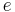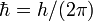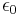where k_e \, is the electrostatic constant, e \, is the elementary charge, \hbar = h/(2 \pi) \, is the reduced Planck constant, c \, is the speed of light in a vacuum, and \epsilon_0 \, is the electric constant.

In electrostatic cgs units, the unit of electric charge (the Statcoulomb or esu of charge) is defined in such a way that the permittivity factor, 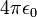4 \pi \epsilon_0 \,, is the dimensionless constant 1. Then the fine-structure constant becomes

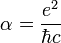\alpha = \frac{e^2}{\hbar c} .

Measurement

Two example eighth-order Feynman diagrams that contribute to the electron self interaction. The horizontal line with an arrow represents the electron while the wavy-lines are virtual photons, and the circles represent virtual electron-positron pairs.

The definition of 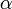\alpha\, contains several other constants which can be measured themselves. However, quantum electrodynamics (QED) provides a way to measure 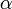\alpha\, directly using the quantum Hall effect or the anomalous magnetic moment of the electron.

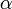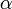QED predicts a relationship between the dimensionless magnetic moment of the electron (or the Lande g-factor, g \,) and the fine structure constant \alpha\,. A new measurement of g \, using a one-electron quantum cyclotron, together with a QED calculation involving 891 four-loop Feynman diagrams, determines the most precise current value of \alpha\,:[1]

\alpha^{-1} = 137.035\,999\,068(96)

i.e., a measurement with a precision of 0.70 ppb. The uncertainties are 10 times smaller than those of the nearest rival methods that include atom-recoil measurements. Comparisons of the measured and calculated values of g \, test QED very stringently, and set a limit on any possible internal structure of the electron.

Physical interpretation

There are several ways to interpret the reality of the Fine-structure constant, including:

  1. the square of the ratio of the elementary to Planck charges
  2. a ratio of certain energies
  3. the ratio between the electron velocity in Bohr's model of the atom and the speed of light
  4. a constant representing the strength of the interaction between electrons and photons
  5. the strength of the electromagnetic interaction, which may change, depending on the strength of the energy field.

The fine-structure constant can be thought of as the square of the ratio of the elementary charge to the Planck charge.

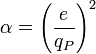\alpha = \left( \frac{e}{q_P} \right)^2.

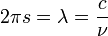For any arbitrary length s \,, the fine-structure constant is the ratio of two energies: (i) the energy needed to bring two electrons from infinity to a distance of s \, against their electrostatic repulsion, and (ii) the energy of a single photon of wavelength equal to the same length scaled by 2π (i.e. 2 \pi s = \lambda = \frac{c}{\nu} \, where \nu \, is the frequency of radiation associated with the photon):

\alpha = \frac{e^2}{4 \pi \epsilon_0 s} \div h \nu = \frac{e^2}{4 \pi \epsilon_0 s} \div \frac{h c}{2 \pi s} = \frac{e^2}{4 \pi \epsilon_0 \hbar c}.

The fine structure constant is also the ratio between the electron velocity in the Bohr atom and the speed of light. The square of alpha is the ratio between the electron rest mass (511 keV) and the Hartree energy (27.2 eV = 2 Ry).

In the theory of quantum electrodynamics, the fine structure constant plays the role of a coupling constant, representing the strength of the interaction between electrons and photons. Its value cannot be predicted by the theory, and has to be inserted based on experimental results. In fact, it is one of the twenty-odd "external parameters" in the Standard Model of particle physics.

The fact that 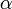\alpha \, is much less than 1 allows the use of perturbation theory in quantum electrodynamics. Physical results in this theory are expressed as power series in 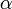\alpha \,, with higher orders of 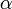\alpha \, increasingly unimportant. In contrast, the large value of the corresponding factors in quantum chromodynamics makes calculations involving the strong force extremely difficult.

In the electroweak theory, one that unifies the weak interaction with electromagnetism, the fine-structure constant is absorbed into two other coupling constants associated with the electroweak gauge fields. In this theory, the electromagnetic interaction is treated as a mixture of interactions associated with the electroweak fields.

According to the theory of renormalization group, the value of the fine-structure constant (the strength of the electromagnetic interaction) depends on the energy scale. In fact, it grows logarithmically as the energy is increased. The observed value of 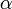\alpha \, is associated with the energy scale of the electron mass; the energy scale does not run below this because the electron (and the positron) is the lightest charged object whose quantum loops can contribute to the running. Therefore, we can say that 1/137.036 is the value of the fine-structure constant at zero energy. Moreover, as the energy scale increases, the electromagnetic interaction approaches the strength of the other two interactions, which is important for the theories of grand unification. If quantum electrodynamics were an exact theory, the fine-structure constant would actually diverge at an energy known as the Landau pole. This fact makes quantum electrodynamics inconsistent beyond the perturbative expansions.

History

The fine-structure constant was originally introduced into physics in 1916 by Arnold Sommerfeld, as a measure of the relativistic deviations in atomic spectral lines from the predictions of the Bohr model.

Historically, the first physical interpretation of the fine-structure constant, 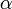\alpha \,, was the ratio of the velocity of the electron in the first circular orbit of the relativistic Bohr atom to the speed of light in vacuum. Equivalently, it was the quotient between the maximum angular momentum allowed by relativity for a closed orbit and the minimum angular momentum allowed for it by quantum mechanics. It appears naturally in Sommerfeld's analysis and determines the size of the splitting or fine-structure of the hydrogenic spectral lines.

Is the fine structure constant really constant?

Physicists have been wondering for many years whether the fine structure constant is really a constant, i.e., whether or not its value is different at different times or in different places. Historically, a varying 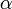\alpha \, has been proposed as a means to solve some of the perceived cosmological problems of the day.[2][3][4] More recently, theoretical interest in varying constants (not just 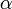\alpha \,) has been motivated by string theory and other such proposals for going beyond the Standard Model of particle physics. The first experimental tests of this question, most notably examination of spectral lines of distant astronomical objects and of radioactive decays in the Oklo natural nuclear fission reactor, found results consistent with no change.[5][6][7][8][9][10]

More recently, technology improvements have made it possible to probe the value of 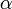\alpha \, at much larger distances and to much greater accuracy. In 1999, a team lead by John K. Webb of the University of New South Wales claimed the first detection of a variation in 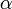\alpha \,.[11][12][13][14] Using the Keck telescopes and a data set of 128 quasars at redshifts 0.5<z<3, Webb et al. found their spectra were consistent with a slight increase in 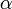\alpha \, over the last 10-12 billion years. Specifically, they found that

\frac{\Delta \alpha}{\alpha} \ \stackrel{\mathrm{def}}{=}\ \frac{\alpha _\mathrm{then}-\alpha _\mathrm{now}}{\alpha_\mathrm{now}} = \left( -0.57\pm 0.10 \right) \times 10^{-5}.

A more recent, smaller, study of 23 absorption systems by Chand et al. using the Very Large Telescope found no measureable variation:[15][16]

\frac{\Delta \alpha}{\alpha_\mathrm{em}}= \left(-0.6\pm 0.6\right) \times 10^{-6}.

The Chand et al. result apparently rules out variation at the level claimed by Webb et al., although there are still concerns about systematic uncertainties. Surveys to provide additional data are ongoing. All other astrophysical results to date are consistent with no variation.[17]

Very recently, Khatri and Wandelt of the University of Illinois at Urbana-Champaign realized that the 21 cm hyperfine transition in neutral hydrogen in the early Universe leaves a unique absorption line imprint in the cosmic microwave background radiation.[18] They proposed using this effect to measure the value of \alpha during the epoch before the formation of the first stars. 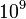In principle, this technique provides enough information to measure a variation of 1 part in 10^{9} (4 orders of magnitude better than the current quasar constraints). However, the constraint which can be placed on \alpha is strongly dependent upon effective integration time, going as 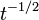t^{-1/2}. The LOFAR telescope would only be able to constrain 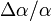\Delta\alpha/\alpha to ~0.3%[19]. The collecting area required to constrain 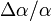\Delta\alpha/\alpha to the current level of quasar constraints is on the order of 100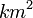km^2, which is impracticable at present.

Anthropic explanation

One controversial explanation of the value of the fine-structure constant invokes the anthropic principle and argues that the value of the fine-structure is what it is because stable matter and therefore life and intelligent beings could not exist if the value were anything else. For instance, were 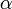\alpha\, to change by 4%, carbon would no longer be produced in stellar fusion. If 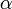\alpha\, were greater than 0.1, fusion would no longer occur in stars.[20]

The fine structure constant plays a central role in John Barrow and Frank Tipler's broad-ranging discussion of astrophysics, cosmology, quantum physics, teleology, and the anthropic principle.[21]

Mathematical explanations

Note: within the scientific community, 'numerology' has a colloquial significance and it is mostly used to refer to any scientific theory that appears to be more mathematical than scientific in its approach.

As a dimensionless constant which does not seem to be directly related to any mathematical constant, the fine-structure constant has long been an object of fascination to physicists. Richard Feynman, one of the founders of quantum electrodynamics, referred to it as "one of the greatest damn mysteries of physics: a magic number that comes to us with no understanding by man."[22]

In 1929, Arthur Eddington conjectured that its reciprocal was precisely the integer 137, constructed numerological arguments that the value could be "obtained by pure deduction", and related it to the Eddington number, his estimate of the number of protons in the Universe. Other physicists neither adopted this conjecture nor accepted his arguments, and by the 1940s, experimental values for 1⁄α deviated sufficiently from 137 to reject that value.[23]

Recently the mathematician James Gilson has suggested [2] that the fine-structure constant has the value:

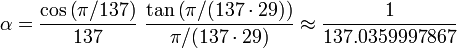\alpha = \frac{\cos \left(\pi/137 \right)}{137} \ \frac{\tan \left(\pi/(137 \cdot 29) \right)}{\pi/(137 \cdot 29)} \approx \frac{1}{137.0359997867} ,

29 and 137 being the 10th and 33rd prime numbers. This deviates from the 2006 CODATA value for α by about one standard uncertainty of measurement, but by more than seven standard deviations from the best α value currently known (2007).

The fine structure constant so intrigued the physicist Wolfgang Pauli that he even collaborated with the psychologist Carl Jung in an extraordinary quest to understand its significance.[24]

Quotes

See also

References

  1. ↑ 1.0 1.1 1.2 G. Gabrielse; D. Hanneke; T. Kinoshita; M. Nio; B. Odom (2006-07-21), "New Determination of the Fine Structure Constant from the Electron g Value and QED", Phys. Rev. Lett. 97 (030802): 030802, doi:10.1103/PhysRevLett.97.030802, http://hussle.harvard.edu/~gabrielse/gabrielse/papers/2006/NewFineStructureConstant.pdf  and G. Gabrielse; D. Hanneke; T. Kinoshita; M. Nio; B. Odom (2007-06-24), "Erratum: New Determination of the Fine Structure Constant from the Electron g Value and QED [Phys. Rev. Lett. 97, 030802 (2006)]", Phys. Rev. Lett. 99 (039902): 039902, doi:10.1103/PhysRevLett.99.039902, http://hussle.harvard.edu/~gabrielse/gabrielse/papers/2006/NewFineStructureConstant.pdf 
  2. ↑ Milne, Edward Arthur (1935). Relativity, Gravitation and World Structure. Oxford: The Clarendon press. 
  3. ↑ P. A. M. Dirac, Nature 139, 323 (1937)
  4. ↑ G. Gamow, Phys. Rev. Lett. 19, 757 and 913 (1967).
  5. ↑ Uzan, Jean-Philippe (2003). "The fundamental constants and their variation: observational status and theoretical motivations". Reviews of Modern Physics (American Physical Society) 75: 403–455. doi:10.1103/RevModPhys.75.403. http://arxiv.org/abs/hep-ph/0205340. Retrieved on 2006-08-12. 
  6. ↑ Uzan, Jean-Philippe (2004). "Variation of the constants in the late and early universe". astro-ph/0409424 (arXiv). http://arxiv.org/abs/astro-ph/0409424. Retrieved on 2006-08-12. 
  7. ↑ Olive, Keith; Qian, Yong-Zhong (2003). "Were Fundamental Constants Different in the Past?". Physics Today (American Institute of Physics) 57 (10): 40–5. doi:10.1063/1.1825267. 
  8. ↑ Barrow, John D. (2002). The Constants of Nature: From Alpha to Omega--the Numbers That Encode the Deepest Secrets of the Universe. London: Vintage. ISBN 0-09-928647-5. 
  9. ↑ Uzan, Jean-Philippe; Bénédicte Leclercq (2008). The natural laws of the universe - Understanding fundamentral constants. Berlin Heidelberg New-York: Springer Praxis. ISBN 978-0-387-73454-5. 
  10. ↑ Fujii, Yasunori (2004). "Oklo Constraint on the Time-Variability of the Fine-Structure Constant". Astrophysics, Clocks and Fundamental Constants. Lecture Notes in Physics. Heidelberg: Springer Berlin. pp. 167-185. ISBN 978-3-540-21967-5. http://www.springerlink.com/content/20dt5p8t8ene319q/. 
  11. ↑ Webb, John K.; et al (1999). "Search for Time Variation of the Fine Structure Constant". Physical Review Letters (American Physical Society) 82 (5): 884–887. doi:10.1103/PhysRevLett.82.884. http://arxiv.org/pdf/astro-ph/9803165. Retrieved on 2006-08-12. 
  12. ↑ M. T. Murphy et al, Mon. Not. Roy. Astron. Soc. 327, 1208 (2001)
  13. ↑ Webb, John K.; et al (2001). "Further Evidence for Cosmological Evolution of the Fine Structure Constant". Physical Review Letters (American Physical Society) 87 (9): 091301. doi:10.1103/PhysRevLett.87.091301. http://arxiv.org/pdf/astro-ph/0012539. Retrieved on 2006-08-12. 
  14. ↑ M.T. Murphy, J.K. Webb and V.V. Flambaum, Mon. Not R. astron. Soc. 345, 609 (2003)
  15. ↑ H. Chand et al., Astron. Astrophys. 417, 853 (2004)
  16. ↑ R. Srianand et al., Phys. Rev. Lett. 92, 121302 (2004).
  17. ↑ Barrow, John D. (2005). "Varying Constants". Philosophical Transactions of the Royal Society (Royal Society) 363: 2139–2153. http://arxiv.org/pdf/astro-ph/0511440. Retrieved on 2006-08-12. 
  18. ↑ Khatri, Rishi; Wandelt, Benjamin D. (2007), "21-cm Radiation: A New Probe of Variation in the Fine-Structure Constant", Physical Review Letters (American Physical Society) 98: 111301, doi:10.1103/PhysRevLett.98.111301, http://arxiv.org/abs/astro-ph/0701752, retrieved on 2007-07-09 
  19. ↑ Khatri, Rishi; Wandelt, Benjamin D. (2007), "21-cm Radiation: A New Probe of Variation in the Fine-Structure Constant", Physical Review Letters (American Physical Society) 98: 111301, doi:10.1103/PhysRevLett.98.111301, http://arxiv.org/abs/astro-ph/0701752, retrieved on 2007-07-09 
  20. ↑ Barrow, John D. (2001). "Cosmology, Life, and the Anthropic Principle". Annals of the New York Academy of Sciences 950 (1): 139–153. doi:10.1111/j.1749-6632.2001.tb02133.x (inactive 2008-06-25). 
  21. ↑ John D. Barrow and Frank J. Tipler, 1986. The Anthropic Cosmological Principle. Oxford University Press.
  22. ↑ Feynman, Richard P. (1985). QED: The Strange Theory of Light and Matter. Princeton University Press. pp. 129. ISBN 0-691-08388-6. 
  23. ↑ Helge Kragh, "Magic Number: A Partial History of the Fine-Structure Constant", Archive for History of Exact Sciences 57:5:395 (July, 2003) doi:10.1007/s00407-002-0065-7
  24. ↑ P.Varlaki, L.Nadai and J.Bokor 'Number Archetypes and Background Control Theory Concerning the Fine Structure Constant' Acta Polytechnica Hungarica 5 no.2 (2008)[1]

External links