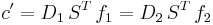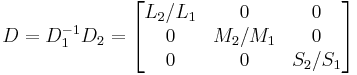Color vision

Color vision is the capacity of an organism or machine to distinguish objects based on the wavelengths (or frequencies) of the light they reflect or emit. The nervous system derives color by comparing the responses to light from the several types of cone photoreceptors in the eye. These cone photoreceptors are sensitive to different portions of the visible spectrum. For humans, the visible spectrum ranges approximately from 380 to 740 nm, and there are normally three types of cones. The visible range and number of cone types differ between species.

A 'red' apple does not emit red light. Rather, it simply absorbs all the frequencies of visible light shining on it except for a group of frequencies that is perceived as red, which are reflected. An apple is perceived to be red only because the human eye can distinguish between different wavelengths. Three things are needed to see color: a light source, a detector (e.g. the eye) and a sample to view.

Psychophysical.jpg

The advantage of color, which is a quality constructed by the visual brain and not a property of objects as such, is the better discrimination of surfaces allowed by this aspect of visual processing.

Contents

Physiology of color perception

Normalized response spectra of human cones, S, M, and L types, to monochromatic spectral stimuli, with wavelength given in nanometers.
The same figures as above represented here as a single curve in three (normalized cone response) dimensions

Perception of color is achieved in mammals through color receptors containing pigments with different spectral sensitivities. In most primates closely related to humans there are three types of color receptors (known as cone cells). This confers trichromatic color vision, so these primates, like humans, are known as trichromats. Many other primates and other mammals are dichromats, and many mammals have little or no color vision.

The cones are conventionally labeled according to the ordering of the wavelengths of the peaks of their spectral sensitivities: short (S), medium (M), and long (L) cone types, also sometimes referred to as blue, green, and red cones. While the L cones are often referred to as the red receptors, microspectrophotometry has shown that their peak sensitivity is in the greenish-yellow region of the spectrum. Similarly, the S- and M-cones do not directly correspond to blue and green, although they are often depicted as such (such as in the graph to the right). It is important to note that the RGB color model is merely a convenient means for representing color, and is not directly based on the types of cones in the human eye.

The peak response of human color receptors varies, even amongst individuals with 'normal' color vision;[1] in non-human species this polymorphic variation is even greater, and it may well be adaptive.[2]

Theories of color vision

Two complementary theories of color vision are the trichromatic theory and the opponent process theory. The trichromatic theory, or Young–Helmholtz theory, proposed in the 19th century by Thomas Young and Hermann von Helmholtz, as mentioned above states that the retina's three types of cones are preferentially sensitive to blue, green, and red. Ewald Hering proposed the opponent process theory in 1872.[3] It states that the visual system interprets color in an antagonistic way: red vs. green, blue vs. yellow, black vs. white. We now know both theories to be correct, describing different stages in visual physiology.

Cone cells in the human eye

Cone type Name Range Peak wavelength[4][5]
S β 400–500 nm 420–440 nm
M γ 450–630 nm 534–545 nm
L ρ 500–700 nm 564–580 nm

A range of wavelengths of light stimulates each of these receptor types to varying degrees. Yellowish-green light, for example, stimulates both L and M cones equally strongly, but only stimulates S-cones weakly. Red light, on the other hand, stimulates L cones much more than M cones, and S cones hardly at all; blue-green light stimulates M cones more than L cones, and S cones a bit more strongly, and is also the peak stimulant for rod cells; and violet light stimulates almost exclusively S-cones. The brain combines the information from each type of receptor to give rise to different perceptions of different wavelengths of light.

The pigments present in the L and M cones are encoded on the X chromosome; defective encoding of these leads to the two most common forms of color blindness. The OPN1LW gene, which codes for the pigment that responds to yellowish light, is highly polymorphic (a recent study by Verrelli and Tishkoff found 85 variants in a sample of 236 men[6]), so up to ten percent of women[7] have an extra type of color receptor, and thus a degree of tetrachromatic color vision.[8] Variations in OPN1MW, which codes for the bluish-green pigment, appear to be rare, and the observed variants have no effect on spectral sensitivity.

Color in the human brain

Visual pathways in the human brain. The ventral stream (purple) is important in color recognition. The dorsal stream (green) is also shown. They originate from a common source in the visual cortex.

Color processing begins at a very early level in the visual system (even within the retina) through initial color opponent mechanisms. Opponent mechanisms refer to the opposing color effect of red-green, blue-yellow, and light-dark. Visual information is then sent back via the optic nerve to the optic chiasm: a point where the two optic nerves meet and information from the temporal (contralateral) visual field crosses to the other side of the brain. After the optic chiasm the visual fiber tracts are referred to as the optic tracts, which enter the thalamus to synapse at the lateral geniculate nucleus (LGN). The LGN is segregated into six layers: two magnocellular (large cell) achromatic layers (M cells) and four parvocellular (small cell) chromatic layers (P cells). Within the LGN P-cell layers there are two chromatic opponent types: red vs. green and blue vs. green/red.

After synapsing at the LGN, the visual tract continues on back toward the primary visual cortex (V1) located at the back of the brain within the occipital lobe. Within V1 there is a distinct band (striation). This is also referred to as "striate cortex", with other cortical visual regions referred to collectively as "extrastriate cortex".It is at this stage that color processing becomes much more complicated.

In V1 the simple three-color segregation begins to break down. Many cells in V1 respond to some parts of the spectrum better than others, but this "color tuning" is often different depending on the adaptation state of the visual system. A given cell that might respond best to long wavelength light if the light is relatively bright might then become responsive to all wavelengths if the stimulus is relatively dim. Because the color tuning of these cells is not stable, some believe that a different, relatively small, population of neurons in V1 is responsible for color vision. These specialized "color cells" often have receptive fields that can compute local cone ratios. Such "double-opponent" cells were initially described in the goldfish retina by Nigel Daw;[9][10] their existence in primates was suggested by David H. Hubel and Torsten Wiesel and subsequently proven by Bevil Conway.[11] As Margaret Livingstone and David Hubel showed, double opponent cells are clustered within localized regions of V1 called blobs, and are thought to come in two flavors, red-green and blue-yellow.[12] Red-green cells compare the relative amounts of red-green in one part of a scene with the amount of red-green in an adjacent part of the scene, responding best to local color contrast (red next to green). Modeling studies have shown that double-opponent cells are ideal candidates for the neural machinery of color constancy explained by Edwin H. Land in his retinex theory.[13]

This image (when viewed in full size, 1000 pixels wide) contains 1 milion pixels, each of a different color. The human eye can distinguish about 10 million different colors.[14]

From the V1 blobs, color information is sent to cells in the second visual area, V2. The cells in V2 that are most strongly color tuned are clustered in the "thin stripes" that, like the blobs in V1, stain for the enzyme cytochrome oxidase (separating the thin stripes are interstripes and thick stripes, which seem to be concerned with other visual information like motion and high-resolution form). Neurons in V2 then synapse onto cells in area V4. Area V4 is a relatively large visual area, the largest by far cortical area outside V1, encompassing almost as much cortex as V1. Neurons in V4 were originally proposed by Semir Zeki to be exclusively dedicated to color, but this has since been shown not to be the case.[15] Quantitative studies have argued that there is no higher concentration of color cells in V4 than in primary visual cortex, although this remains controversial. Independent of color sensitivity, V4 neurons have been shown to be very sensitive to the shape of stimuli, curvature, and stereo-scopic depth. V4 neurons have also been shown to be modulated by attention. The role of V4 neurons in color vision remains to be better characterized: indeed the vast majority of scientific papers examining the function of V4 do not concern color processing.

Anatomical studies have shown that neurons in V4 provide input to the inferior temporal lobe . "IT" cortex is thought to integrate color information with shape and form, although it has been difficult to define the appropriate criteria for this claim. Despite this murkiness, it has been useful to characterize this pathway (V1 > V2 > V4 > IT) as the ventral stream or the "what pathway", distinguished from the dorsal stream ("where pathway") that is thought to analyze motion, among many other features.

In other animals

Other animals, such as tropical fish and birds, have more complex color vision systems than humans.[16] In the latter example, tetrachromacy is achieved through up to four cone types, depending on species. Brightly colored oil droplets inside the cones shift or narrow the spectral sensitivity of the cell. It has been suggested that it is likely that pigeons are pentachromats. Eutherian mammals other than primates generally have less-effective two-receptor (dichromatic) color perception systems. Marine mammals have only a single cone type and are thus monochromats. Several marsupials such as the fat-tailed dunnart (Sminthopsis crassicaudata) have been shown to have trichromatic colour vision[17]. Many invertebrates have color vision. Honey- and bumblebees have trichromatic color vision, which is insensitive to red but sensitive in ultraviolet to a color called bee purple. Papilio butterflies apparently have tetrachromatic color vision despite possessing six photoreceptor types.[18] The most complex color vision system in animal kingdom has been found in stomatopods with up to 12 different spectral receptor types which are thought to work as multiple dichromatic units.[19]

Evolution

Main article: Evolution of color vision

Color perception mechanisms are highly dependent on evolutionary factors, of which the most prominent is thought to be satisfactory recognition of food sources. In herbivorous primates, color perception is essential for finding proper (mature) leaves. In hummingbirds, particular flower types are often recognized by color as well. On the other hand, nocturnal mammals have less-developed color vision, since adequate light is needed for cones to function properly. There is evidence that ultraviolet light plays a part in color perception in many branches of the animal kingdom, especially insects. In general, the optical spectrum encompasses the most common electronic transitions in matter and is therefore the most useful for collecting information about the environment.

The evolution of trichromatic color vision in primates occurred as the ancestors of modern monkeys, apes, and humans switched to diurnal (daytime) activity and began consuming fruits and leaves from flowering plants.[20]

Some animals can distinguish colors in the ultraviolet spectrum. The UV spectrum falls below the human visible range. Birds, turtles, lizards, and fish have UV receptors in their retinas. These animals can see the UV patterns found on flowers and other wildlife that are otherwise invisible to the human eye. So far, there has not been enough evidence to show that any mammals are capable of UV vision.[21]

UV and multi-dimensional vision is an especially important adaptation in birds. It allows birds to spot small prey from a distance, navigate, avoid predators, and forage while flying at high speeds. Birds also utilize their broad spectrum vision to recognize other birds, and in sexual selection.[22][23]

Mathematics of color perception

A "physical color" is a combination of pure spectral colors (in the visible range). Since there are, in principle, infinitely many distinct spectral colors, the set of all physical colors may be thought of as an infinite-dimensional vector space, in fact a Hilbert space. We call this space Hcolor. More technically, the space of physical colors may be considered to be the (mathematical) cone over the simplex whose vertices are the spectral colors.

An element C of Hcolor is a function from the range of visible wavelengths—considered as an interval of real numbers [Wmin,Wmax]—to the real numbers, assigning to each wavelength w in [Wmin,Wmax] its intensity C(w).

A humanly perceived color may be modeled as three numbers: the extents to which each of the 3 types of cones is stimulated. Thus a humanly perceived color may be thought of as a point in 3-dimensional Euclidean space. We call this space R3color.

Since each wavelength w stimulates each of the 3 types of cone cells to a known extent, these extents may be represented by 3 functions s(w), m(w), l(w) corresponding to the response of the S, M, and L cone cells, respectively.

Finally, since a beam of light can be composed of many different wavelengths, to determine the extent to which a physical color C in Hcolor stimulates each cone cell, we must calculate the integral (with respect to w), over the interval [Wmin,Wmax], of C(w)*s(w), of C(w)*m(w), and of C(w)*l(w). The triple of resulting numbers associates to each physical color C (which is a region in Hcolor) to a particular perceived color (which is a single point in R3color). This association is easily seen to be linear. It may also easily be seen that many different regions in the "physical" space Hcolor can all result in the same single perceived color in R3color, so a perceived color is not unique to one physical color.

Thus human color perception is determined by a specific, non-unique linear mapping from the infinite-dimensional Hilbert space Hcolor to the 3-dimensional Euclidean space R3color.

Technically, the image of the (mathematical) cone over the simplex whose vertices are the spectral colors, by this linear mapping, is also a (mathematical) cone in R3color. Moving directly away from the vertex of this cone represents maintaining the same chromaticity while increasing its intensity. Taking a cross-section of this cone yields a 2D chromaticity space. Both the 3D cone and its projection or cross-section are convex sets; that is, any mixture of spectral colors is also a color.

The CIE 1931 color space chromaticity diagram. The outer curved boundary is the spectral (or monochromatic) locus, with wavelengths shown in nanometers. Note that the colors depicted depend on the color space of the device on which you are viewing the image, and therefore may not be a strictly accurate representation of the color at a particular position.

In practice, it would be quite difficult to measure an individual's cones' three responses to various physical color stimuli. So instead, three specific benchmark test lights are typically used; let us call them S, M, and L. In order to calibrate human perceptual space, scientists allowed human subjects to try to match any physical color by turning dials to create specific combinations of intensities (IS, IM, IL) for the S, M, and L lights, resp., until a match was found. This needed only to be done for physical colors that are spectral (since a linear combination of spectral colors will be matched by the same linear combination of their (IS, IM, IL) matches). Note that in practice, often at least one of S, M, L would have to be added with some intensity to the physical test color, and that combination matched by a linear combination of the remaining 2 lights. Across different individuals (without color blindness), the matchings turned out to be nearly identical.

By considering all the resulting combinations of intensities (IS, IM, IL) as a subset of 3-space, a model for human perceptual color space is formed. (Note that when one of S, M, L had to be added to the test color, its intensity was counted as negative.) Again, this turns out to be a (mathematical) cone—not a quadric, but rather all rays through the origin in 3-space passing through a certain convex set. Again, this cone has the property that moving directly away from the origin corresponds to increasing the intensity of the S, M, L lights proportionately. Again, a cross-section of this cone is a planar shape that is (by definition) the space of "chromaticities" (informally: distinct colors); one particular such cross section, corresponding to constant X+Y+Z of the CIE 1931 color space, gives the CIE chromaticity diagram.

It should be noted that this system implies that for any hue or non-spectral color, there are infinitely many distinct physical spectra that are all perceived as that hue or color. So, in general there is no such thing as the combination of spectral colors that we perceive as (say) yellow-green; instead there are infinitely many possibilities.

(The only exceptions to this rule are the perceptual colors corresponding to the boundary of the cone: in other words, those chromaticities on the simple closed curve that is the boundary of the 1931 C.I.E. diagram depicted in the figure. These comprise precisely all spectral colors plus the "line of purples" connecting the ends of the spectral colors: for each of these, there is only one physical color in Hcolor that can create that perceived color.)

The CIE chromaticity diagram is horseshoe-shaped, with its curved edge corresponding to all spectral colors (the spectral locus), and the remaining straight edge corresponding to the most saturated purples—mixtures of red and violet.

Chromatic adaptation

An object may be viewed under various conditions. For example, it may be illuminated by sunlight, the light of a fire, or a harsh electric light. In all of these situations, human vision perceives that the object has the same color: an apple always appears red, whether viewed at night or during the day. On the other hand, a camera with no adjustment for light may register the apple as having varying color. This feature of the visual system is called chromatic adaptation, or color constancy; when the correction occurs in a camera it is referred to as white balance.

Chromatic adaptation is one aspect of vision that may fool someone into observing a color-based optical illusion, such as the same color illusion.

Though the human visual system generally does maintain constant perceived color under different lighting, there are situations where the relative brightness of two different stimuli will appear reversed at different illuminance levels. For example, the bright yellow petals of flowers will appear dark compared to the green leaves in dim light while the opposite is true during the day. This is known as the Purkinje effect, and arises because the peak sensitivity of the human eye shifts toward the blue end of the spectrum at lower light levels.

Von Kries transform

The von Kries chromatic adaptation method is a technique that is sometimes used in camera image processing. The method is to apply a gain to each of the human cone cell spectral sensitivity responses so as to keep the adapted appearance of the reference white constant. The application of Johannes von Kries's idea of adaptive gains on the three cone cell types was first explicitly applied to the problem of color constancy by Herbert E. Ives,[24][25] and the method is sometimes referred to as the Ives transform[26] or the von Kries–Ives adaptation.[27]

The von Kries coefficient rule rests on the assumption that color constancy is achieved by individually adapting the gains of the three cone responses, the gains depending on the sensory context, that is, the color history and surround. Thus, the cone responses 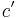c' from two radiant spectra can be matched by appropriate choice of diagonal adaptation matrices 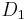D_1 and D_2[28]:

c'=D_1\,S^T\,f_1 = D_2\,S^T\,f_2

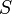where S is the cone sensitivity matrix and 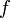f is the spectrum of the conditioning stimulus. This leads to the von Kries transform for chromatic adaptation in LMS color space (responses of long-, medium-, and short-wavelength cone response space):

D = D_1^{-1} D_2=\begin{bmatrix} L_2/L_1 & 0 & 0 \\ 0 & M_2/M_1 & 0 \\ 0 & 0 & S_2/S_1 \end{bmatrix}

This diagonal matrix D maps cone responses, or colors, in one adaptation state to corresponding colors in another; when the adaptation state is presume to be determined by the illuminant, this matrix is useful as an illuminant adaptation transform. The elements of the diagonal matrix D are the ratios of the cone responses (Long, Medium, Short) for the illuminant's white point.

The more complete von Kries transform, for colors represented in XYZ or RGB color space, includes matrix transformations into and out of LMS space, with the diagonal transform D in the middle.[29]

References

  1. ↑ Neitz, Jay & Jacobs, Gerald H. (1986). "Polymorphism of the long-wavelength cone in normal human colour vision." Nature. 323, 623-625.
  2. ↑ Jacobs, Gerald H. (1996). "Primate photopigments and primate color vision." PNAS. 93 (2), 577–581.
  3. ↑ Hering, Ewald (1872). "Zur Lehre vom Lichtsinne". Sitzungsberichte der Mathematisch–Naturwissenschaftliche Classe der Kaiserlichen Akademie der Wissenschaften LXVI. Band (III Abtheilung). http://books.google.com/books?id=u5MCAAAAYAAJ&pg=PA5&lpg=PA5&dq=1872+hering+ewald+Zur+Lehre+vom+Lichtsinne.+Sitzungsberichte+der+kaiserlichen+Akademie+der+Wissenschaften.+Mathematisch%E2%80%93naturwissenschaftliche+Classe,&source=web&ots=fAdrz1yI8x&sig=99NSKb_P8-_QSDO1RTzt35QTRyk&hl=en. 
  4. ↑ Wyszecki, Günther; Stiles, W.S. (1982). Color Science: Concepts and Methods, Quantitative Data and Formulae (2nd ed. ed.). New York: Wiley Series in Pure and Applied Optics. ISBN 0-471-02106-7. 
  5. ↑ R. W. G. Hunt (2004). The Reproduction of Colour (6th ed. ed.). Chichester UK: Wiley–IS&T Series in Imaging Science and Technology. pp. 11–12. ISBN 0-470-02425-9. 
  6. ↑ Verrelli, BC; Tishkoff, S (2004). "Color vision molecular variation." American Journal of Human Genetics. 75 (3), 363-375
  7. ↑ Biological color vision inspires artificial color processing
  8. ↑ Roth, Mark (2006). "Some women may see 100 million colors, thanks to their genes" Post-Gazette.com
  9. ↑ Nigel W. Daw (17 November 1967). "Goldfish Retina: Organization for Simultaneous Color Contrast". Science 158 (3803): 942–944. doi:10.1126/science.158.3803.942. PMID 6054169. 
  10. ↑ Bevil R. Conway (2002). Neural Mechanisms of Color Vision: Double-Opponent Cells in the Visual Cortex. Springer. ISBN 1402070926. http://books.google.com/books?id=pFodUlHfQmcC&pg=PR7&dq=goldfish+retina+by+Nigel-Daw&as_brr=3&ei=2AWqR764JI7-iAGh8vwE&sig=7vvLHGgrRP_QtPH6mjLuiqblglU. 
  11. ↑ Conway, Bevil R (2001). "Spatial structure of cone inputs to color cells in alert macaque primary visual cortex (V-1)" Journal of Neuroscience. 21 (8), 2768-2783.
  12. ↑ John E. Dowling (2001). Neurons and Networks: An Introduction to Behavioral Neuroscience. Harvard University Press. ISBN 0674004620. http://books.google.com/books?id=adeUwgfwdKwC&pg=PA376&dq=Margaret+Livingstone+David+Hubel+double+opponent+blobs&as_brr=3&ei=YQaqR9-lAY6CiQHm1cmnCg&sig=D3znxI88shgNd8onK0RAWEMh6zY. 
  13. ↑ McCann, M., ed. 1993. Edwin H. Land's Essays. Springfield, Va.: Society for Imaging Science and Technology.
  14. ↑ Judd, Deane B.; Wyszecki, Günter (1975). Color in Business, Science and Industry. Wiley Series in Pure and Applied Optics (third edition ed.). New York: Wiley-Interscience. p. 388. ISBN 0471452122. 
  15. ↑ John Allman and Steven W. Zucker, "On cytochrome oxidase blobs in visual cortex," in Laurence Harris and Michael Jenkin, editors (1993). Spatial Vision in Humans and Robots: The Proceedings of the 1991 York Conference. Cambridge University Press. ISBN 0521430712. http://books.google.com/books?id=eWBiKaOCNIYC&pg=PA34&dq=v4+zeki+color&lr=&as_brr=3&ei=KBCqR7eGF4bQiwHpnZSoCg&sig=F_rbsAj3FD69wRMzWGhB1vK4RuQ. 
  16. ↑ Kelber, A., Osorio, D., Vorobyev, M. (2003) "Animal colour vision--behavioural tests and physiological concepts." Biol Rev Camb Philos Soc. 2003 Feb; 78(1):81-118.
  17. ↑ Catherine A. Arrese, Lyn D. Beazley and Christa Neumeyer: Behavioural evidence for marsupial trichromacy. Current Biology 16:193-194 (2006)
  18. ↑ Arikawa, K. (2003) "Spectral organization of the eye of a butterfly, Papilio". J. Comp. Phys. A 189, 791-800.
  19. ↑ Cronin T.W., Marshall, N.J. (1989) "A retina with at least ten spectral types of photoreceptors in a mantis shrimp" Nature 339, 137 - 140.
  20. ↑ Steven Pinker. How the Mind Works, 1997. p. 191. ISBN 0-393-04535-8.
  21. ↑ Timothy H. Goldsmith. "What Birds See", Scientific American, July 2006, Vol. 295, Issue 1.
  22. ↑ FJ Varela, AG Palacios, and TM Goldsmith. "Vision, Brain, and Behavior in Birds", 1993. p. 77-94.
  23. ↑ IC Cuthill, JC Partridge, ATD Bennett, SC Church, NS Hart, and S Hunt. “Ultraviolet Vision in Birds”. Advances in the Study of Behavior. 2000. Vol. 29: 159-214.
  24. ↑ Ives, H.E. (1912) "The relation between the color of the illuminant and the color of the illuminated object." Trans. Illuminat. Eng. Soc. 7, 62–72, (Reprinted in: Color Res. Appl. 20, 70–75.).
  25. ↑ Hannah E. Smithson and Qasim Zaidi (2004). "Colour constancy in context: Roles for local adaptation and levels of reference". Journal of Vision 4 (9): 693–710. doi:10.1167/4.9.3. http://www.journalofvision.org/4/9/3/article.aspx. 
  26. ↑ Hannah E. Smithson (2005). "Review. Sensory, computational and cognitive components of human colour constancy". Philosophical Transactions of the Royal Society 360 (1458): 1329–1346. doi:10.1098/rstb.2005.1633. http://journals.royalsociety.org/content/px26ma7w586vq2a7/. 
  27. ↑ Karl R. Gegenfurtner, L. T. Sharpe (1999). Color Vision: From Genes to Perception. Cambridge University Press. ISBN 052100439X. http://books.google.com/books?id=9R1ogJsPHi8C&pg=PA413&dq=von-kries+ives&ei=gYuSR--JOZ6ktgOAkPVE&ie=ISO-8859-1&sig=9fSl-f7sE95QZ2mfBQSauPMhvrc. 
  28. ↑ Gaurav Sharma (2003). Digital Color Imaging Handbook. CRC Press. 
  29. ↑ Erik Reinhard (2006). High Dynamic Range Imaging: Acquisition, Display, and Image-Based Lighting. Morgan Kaufmann. ISBN 0125852630. http://books.google.com/books?id=dH2lRxTg1UsC&pg=PA39&dq=von-kries-transform&ei=fIGSR6jlBoiGsgOK4qE9&sig=OjjbcXI1dbMkdqZWm3ojUvqV92Q#PPA36,M1. 

See also

External links

Color vision
Color vision · Color blindness · Opponent process
Monochromacy · Dichromacy · Trichromacy · Tetrachromacy · Pentachromacy
See Color space for mathematical representation models.