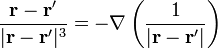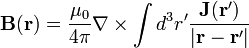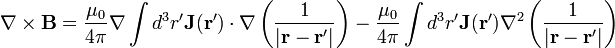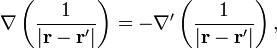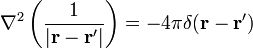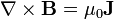Biot–Savart law
| Electromagnetism | ||||||||||||
 |
||||||||||||
Electricity · Magnetism
|
||||||||||||
The Biot–Savart Law is an equation in electromagnetism that describes the magnetic field B generated by an electric current. The vector field B depends on the magnitude, direction, length, and proximity of the electric current, and also on a fundamental constant called the magnetic constant. The law is valid in the magnetostatic approximation, and results in a B field consistent with both Ampère's circuital law and Gauss's law for magnetism.[1]
Contents |
Introduction
The Biot–Savart law is used to compute the magnetic field generated by a steady current, i.e. a continual flow of charges, for example through a wire, which is constant in time and in which charge is neither building up nor depleting at any point. The equation is as follows:
(in SI units), where
 is the current,
is the current,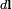 is a vector, whose magnitude is the length of the differential element of the wire, and whose direction is the direction of conventional current,
is a vector, whose magnitude is the length of the differential element of the wire, and whose direction is the direction of conventional current, is the differential contribution to the magnetic field resulting from this differential element of wire,
is the differential contribution to the magnetic field resulting from this differential element of wire,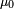 is the magnetic constant,
is the magnetic constant, is the displacement unit vector in the direction pointing from the wire element towards the point at which the field is being computed,
is the displacement unit vector in the direction pointing from the wire element towards the point at which the field is being computed,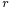 and is the distance from the wire element to the point at which the field is being computed,
and is the distance from the wire element to the point at which the field is being computed,- the symbols in boldface denote vector quantities.
To apply the equation, you choose a point in space at which you want to compute the magnetic field. Holding that point fixed, you integrate over the path of the current(s) to find the total magnetic field at that point. The application of this law implicitly relies on the superposition principle for magnetic fields, i.e. the fact that the magnetic field is a vector sum of the field created by each infinitesimal section of the wire individually.[2]
Another form of the equation is:
Here, r is the full displacement vector instead of a unit vector and it has distance r3 in the denominator to compensate. This equation is applied identically to the one above.
The formulations given above work well when the current can be approximated as running through an infinitely-narrow wire. If the current has some thickness, the proper formulation of the Biot-Savart law (again in SI units) is:
where
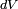 is the differential element of volume and
is the differential element of volume and is the current density vector in that volume.
is the current density vector in that volume.
The Biot-Savart law is fundamental to magnetostatics, playing a similar role to Coulomb's law in electrostatics.
Forms
General
In the magnetostatic approximation, the magnetic field can be determined if the current density j is known:
where 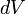 is the differential element of volume.
is the differential element of volume.
Constant uniform current
In the special case of a constant, uniform current I, the magnetic field B is
Point charge at constant velocity
In the case of a charged point particle q moving at a constant, non-relativistic velocity v, then Maxwell's equations give the following expression for the magnetic field:[3]
This equation is also sometimes called the "Biot-Savart law," due to its closely analogous form to the "standard" Biot-Savart law given above. Note that the law is only approximate, with its accuracy decreasing as the particle's velocity approaches c; this happens because the situation is not perfectly approximated by magnetostatics.
This expression can also be rewritten as[3]
where E is the electric field which the charge would create if it were stationary (as given by Coulomb's law), i.e.
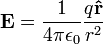 .
.
The exact, relativistic expression is as follows:[3]
where 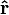 is the vector pointing from the current (non-retarded) position of the particle to the point at which the field is being measured, and θ is the angle between the velocity vector and
is the vector pointing from the current (non-retarded) position of the particle to the point at which the field is being measured, and θ is the angle between the velocity vector and 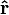 .
.
Magnetic responses applications
The Biot-Savart law can be used in the calculation of magnetic responses even at the atomic or molecular level, e.g. chemical shieldings or magnetic susceptibilities, provided that the current density can be obtained from a quantum mechanical calculation or theory.
Aerodynamics applications
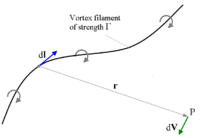
 ) by a vortex filament of strength
) by a vortex filament of strength  .
.The Biot-Savart law is also used to calculate the velocity induced by vortex lines in aerodynamic theory.
In the aerodynamic application, the roles of vorticity and current are reversed as when compared to the magnetic application.
In Maxwell's 1861 paper 'On Physical Lines of Force', magnetic field strength H was directly equated with pure vorticity (spin), whereas B was a weighted vorticity that was weighted for the density of the vortex sea. Maxwell considered magnetic permeability μ to be a measure of the density of the vortex sea. Hence the relationship,
(1) Magnetic Induction Current
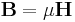
was essentially a rotational analogy to the linear electric current relationship,
(2) Electric Convection Current

where ρ is electric charge density. B was seen as a kind of magnetic current of vortices aligned in their axial planes, with H being the circumferential velocity of the vortices.
The electric current equation can be viewed as a convective current of electric charge that involves linear motion. By analogy, the magnetic equation is an inductive current involving spin. There is no linear motion in the inductive current along the direction of the B vector. The magnetic inductive current represents lines of force. In particular, it represents lines of inverse square law force.
In aerodynamics the induced air currents are forming solenoidal rings around a vortex axis that is playing the role that electric current plays in magnetism. This puts the air currents of aerodynamics into the equivalent role of the magnetic induction vector B in electromagnetism.
In electromagnetism the B lines form solenoidal rings around the source electric current, whereas in aerodynamics, the air currents form solenoidal rings around the source vortex axis.
Hence in electromagnetism, the vortex plays the role of 'effect' whereas in aerodynamics, the vortex plays the role of 'cause'. Yet when we look at the B lines in isolation, we see exactly the aerodynamic scenario in so much as that B is the vortex axis and H is the circumferential velocity as in Maxwell's 1861 paper.
For a vortex line of infinite length, the induced velocity at a point is given by
where
 is the strength of the vortex
is the strength of the vortex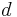 is the perpendicular distance between the point and the vortex line.
is the perpendicular distance between the point and the vortex line.
This is a limiting case of the formula for vortex segments of finite length:
where A and B are the (signed) angles between the line and the two ends of the segment.
The Biot-Savart law, Ampère's circuital law, and Gauss's law for magnetism
Here is a demonstration that the magnetic field B as computed from the Biot-Savart law will always satisfy Ampere's circuital law and Gauss's law for magnetism.[4] Click "show" in the box below for an outline of the proof.
-
Outline of proof that a magnetic field calculated by the Biot-Savart law will always satisfy Gauss's law for magnetism and Ampere's law. We start with the Biot-Savart law: Plugging in the well-known relation
and using the product rule for curls, as well as the fact that J does not depend on the unprimed coordinates, this equation can be rewritten as
Since the divergence of a curl is always zero, this establishes Gauss's law for magnetism. Next, taking the curl of both sides, using the formula for the curl of a curl (see the article Curl (mathematics)), and again using the fact that J does not depend on the unprimed coordinates, we eventually get the result
Finally, plugging in the relations
(where δ is the Dirac delta function), using the fact that the divergence of J is zero (due to the assumption of magnetostatics), and performing an integration by parts, the result turns out to be
i.e. Ampere's law.
See also
People
- Jean-Baptiste Biot
- Félix Savart
- André-Marie Ampère
- James Clerk Maxwell
Electromagnetism
Aerodynamics
- vorticity
- thin-airfoil theory
References
- Griffiths, David J. (1998). Introduction to Electrodynamics (3rd ed. ed.). Prentice Hall. ISBN 0-13-805326-X.
- Feynman, Richard (1966). The Feynman Lectures on Physics. Addison-Wesley. ISBN 0-63-20717.
- ↑ Jackson, John David (1999). Classical Electrodynamics (3rd ed. ed.). New York: Wiley. p. Chapter 5. ISBN 0-471-30932-X.
- ↑ The superposition principle holds for the electric and magnetic fields because they are the solution to a set of linear differential equations, namely Maxwell's equations, where the current is one of the "source terms".
- ↑ 3.0 3.1 3.2 See Griffiths, Example 10.4
- ↑ See Jackson, page 178–9 or Griffiths p. 222–4. The presentation in Griffiths is particularly thorough, with all the details spelled out.
External links
- Electromagnetism, B. Crowell, Fullerton College
- MISN-0-125 The Ampere-Laplace-Biot-Savart Law (PDF file) by Orilla McHarris and Peter Signell for Project PHYSNET.

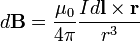

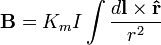

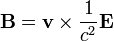
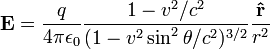
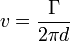
![v = \frac{\Gamma}{4 \pi d} \left[\cos A + \cos B \right]](/2009-wikipedia_en_wp1-0.7_2009-05/I/cbc751080a60234aa653ed65b5ffddb8.png)